Tìm giá trị nhỏ nhất:
A= x^2 - 12x + 18
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
CL
1
MH
12 tháng 4 2022
\(a=\left|x-2021\right|+\left|x-2022\right|\)
\(=\left|x-2021\right|+\left|2022-x\right|\)
\(\ge\left|x-2021+2022-x\right|=1\)
\(A=1\Leftrightarrow\left(x-2021\right)\left(2022-x\right)\ge0\)
\(\Rightarrow2021\le x\le2022\)
LN
2
20 tháng 9 2021
a) Do \(\left|x\right|\ge0\)
\(\Rightarrow A=\left|x\right|+5\ge5\)
\(minA=5\Leftrightarrow x=0\)
b) Do \(\left|x-\dfrac{2}{3}\right|\ge0\)
\(\Rightarrow B=\left|x-\dfrac{2}{3}\right|-4\ge-4\)
\(minB=-4\Leftrightarrow x=\dfrac{2}{3}\)
c) Do \(\left|3x-1\right|\ge0\)
\(\Rightarrow C=\left|3x-1\right|-\dfrac{1}{2}\ge-\dfrac{1}{2}\)
\(minC=-\dfrac{1}{2}\Leftrightarrow x=\dfrac{1}{3}\)
20 tháng 9 2021
\(A=\left|x\right|+5\ge5\)
Dấu \("="\Leftrightarrow x=0\)
\(B=\left|x-\dfrac{2}{3}\right|-4\ge-4\)
Dấu \("="\Leftrightarrow x-\dfrac{2}{3}=0\Leftrightarrow x=\dfrac{2}{3}\)
\(C=\left|3x-1\right|-\dfrac{1}{2}\ge-\dfrac{1}{2}\)
Dấu \("="\Leftrightarrow3x-1=0\Leftrightarrow x=\dfrac{1}{3}\)
NN
3
MT
1
TL
1
24 tháng 4 2022
\(A=\dfrac{27-12x}{x^2+9}=\dfrac{x^2-12x+36-\left(x^2+9\right)}{x^2+9}=\dfrac{\left(x-6\right)^2}{x^2+9}-1\ge-1\)
\(A_{min}=-1\Leftrightarrow x=6\)
\(A=\dfrac{27-12x}{x^2+9}=\dfrac{4\left(x^2+9\right)-\left(4x^2+12x+9\right)}{x^2+9}=4-\dfrac{\left(2x+3\right)^2}{x^2+9}\le4\)
\(A_{max}=4\Leftrightarrow x=\dfrac{-3}{2}\)
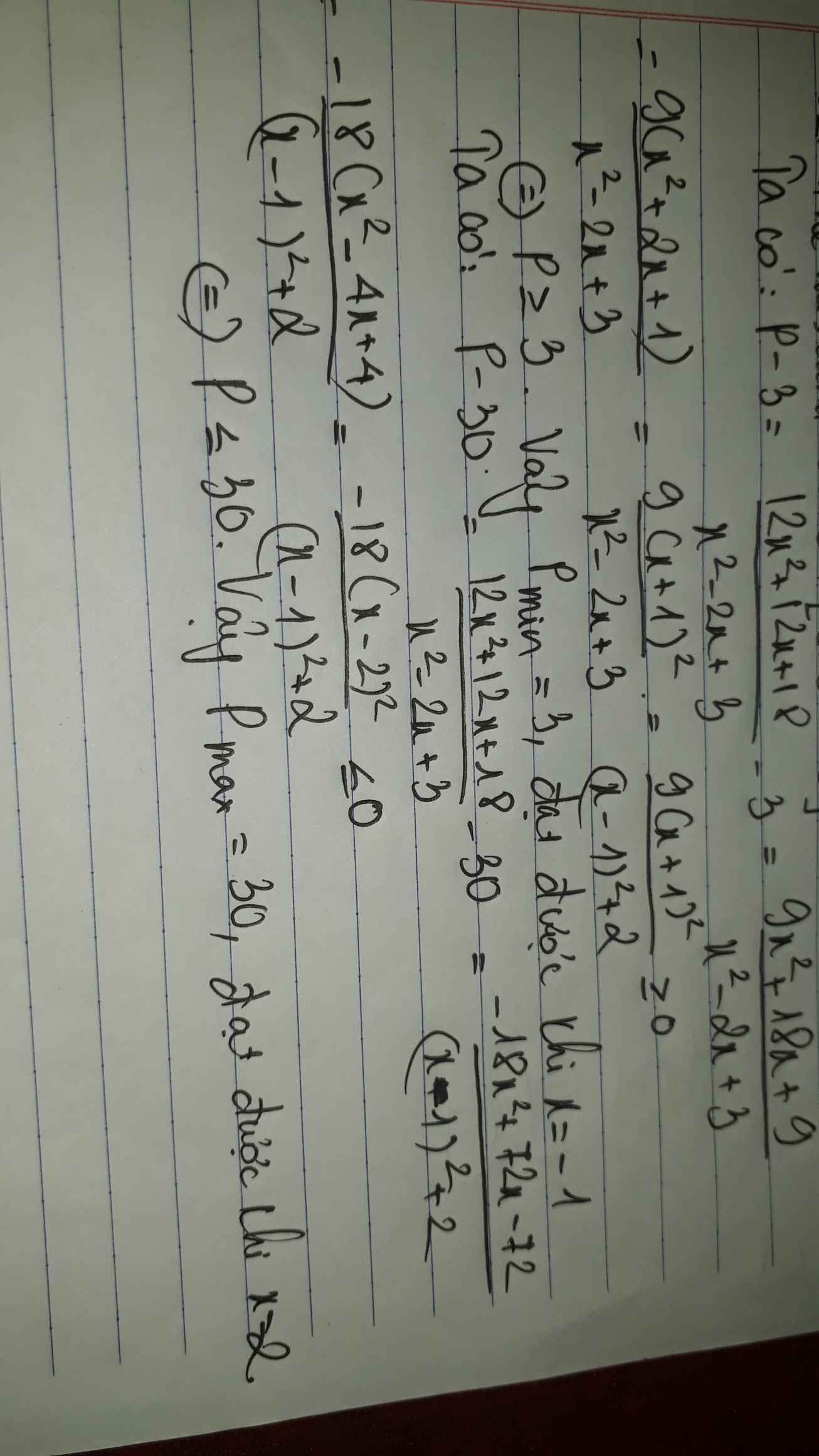
\(A=x^2-12x+18\)
\(A=x^2-2.x.6+36-36+18\)
\(A=\left(x-6\right)^2-18\)
Vì \(\left(x-6\right)^2\ge0\)
Nên \(\left(x-6\right)^2-18\ge-18\)
Vậy \(A_{MIN}=-18\Leftrightarrow x-6=0\Leftrightarrow x=6\)
Ta có : \(A=x^2-12x+18\)
\(=x^2-2.x.6+6^2-18\)
\(=\left(x-6\right)^2-18\)
Có : \(\left(x-6\right)^2\ge0\)
\(\Rightarrow\left(x-6\right)^2-18\ge-18\)
Dấu " = " xảy ra khi \(x-6=0\)
\(x=6\)
Vậy \(MIN_A=-18\) khi \(x=6\)