Xác đinh ̣ m để với moi ̣ x ta có \(-1\le\dfrac{x^2+5x+m}{2x^2-3x+2}< 7\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(-1\le\dfrac{x^2+5x+m}{2x^2-3x+2}< 7\) ∀x ∈ R
ta thấy \(2x^2-3x+2\) (*)vô nghiệm => * luôn dương ( cx dấu vs a)
\(\left\{{}\begin{matrix}\dfrac{x^2+5x+m}{2x^2-3x+2}+1\ge0\\\dfrac{x^2+5x+m}{2x^2-3x+2}-7< 0\end{matrix}\right.\)
\(\left\{{}\begin{matrix}3x^{2^{ }}+2x+m+2\ge0\\-13x^2+26x+m-14< 0\end{matrix}\right.\)
\(\left\{{}\begin{matrix}\left[{}\begin{matrix}a>0\\\Delta\le0\end{matrix}\right.\\\left[{}\begin{matrix}a< 0\\\Delta< 0\end{matrix}\right.\end{matrix}\right.\)
.....
tới đây bạn tự thế số vào làm tiếp nhé
Đ\Á :[\(\dfrac{-5}{3}\);1)

1.
\(-4\le\dfrac{x^2-2x-7}{x^2+1}\le1\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2-2x-7\le x^2+1\\-4x^2-4\le x^2-2x-7\end{matrix}\right.\) (Do \(x^2+1>0\))
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge-4\\\left[{}\begin{matrix}x\ge1\\x\le-\dfrac{3}{5}\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x\ge1\\-4\le x\le-\dfrac{3}{5}\end{matrix}\right.\)
2.
\(\dfrac{1}{13}\le\dfrac{x^2-2x-2}{x^2-5x+7}\le1\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2-5x+7\le13x^2-26x-26\\x^2-2x-2\le x^2-5x+7\end{matrix}\right.\) (Do \(x^2-5x+7>0\))
\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x\ge\dfrac{11}{4}\\x\le-1\end{matrix}\right.\\x\le3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\dfrac{11}{4}\le x\le3\\x\le-1\end{matrix}\right.\)

\(\dfrac{2}{2x-6}+\dfrac{2}{2x+2}+\dfrac{2x}{\left(x+1\right)\left(3-x\right)}=0\) ( x # 3 ; x # -1)
⇔ \(\dfrac{2}{2\left(x-3\right)}+\dfrac{2}{2\left(x+1\right)}+\dfrac{2x}{\left(x+1\right)\left(3-x\right)}=0\)
⇔ \(\dfrac{x+1}{\left(x-3\right)\left(x+1\right)}+\dfrac{x-3}{\left(x+1\right)\left(x-3\right)}-\dfrac{2x}{\left(x+1\right)\left(x-3\right)}=0\)
⇔ x + 1 + x - 3 - 2x = 0
⇔ - 2 = 0 ( vô lý )
Vậy , phương trình vô nghiệm

\(\frac{x^2+5x+a}{2x^2-3x+2}\ge-1\Leftrightarrow\frac{x^2+5x+a}{2x^2-3x+2}+1\ge0\Leftrightarrow\frac{3x^2+2x+a+2}{2x^2-3x+2}\ge0\)
\(\Leftrightarrow3x^2+2x+a+2\ge0\) \(\forall x\) (do \(2x^2-3x+2=2\left(x-\frac{3}{4}\right)^2+\frac{7}{8}>0\))
\(\Rightarrow\Delta'=1-3\left(a+2\right)=-5-3a\le0\Rightarrow a\ge\frac{-5}{3}\) (1)
Lại có: \(\frac{x^2+5x+a}{2x^2-3x+2}\le7\Leftrightarrow\frac{x^2+5x+a}{2x^2-3x+2}-7\le0\Leftrightarrow\frac{-13x^2+26x+a-14}{2x^2-3x+2}\le0\)
\(\Leftrightarrow-13x^2+26x+a-14\le0\) \(\forall x\)
\(\Rightarrow\Delta'=169+13\left(a-14\right)\le0\Rightarrow a\le-1\) (2)
Kết hợp (1) và (2) ta được: \(\frac{-5}{3}\le a\le-1\)
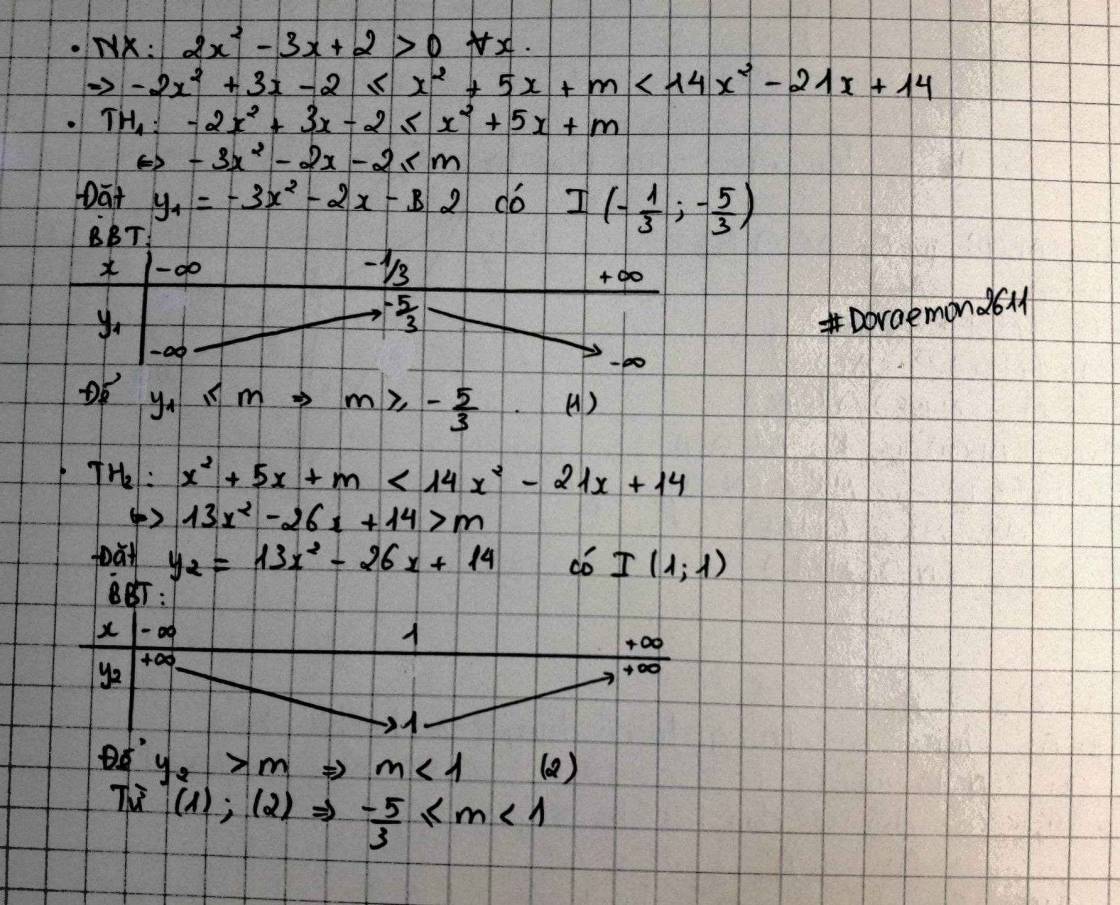
Đk: \(x\in R\)
Có \(2x^2-3x+2>0;\forall x\)
\(-1\le\dfrac{x^2+5x+m}{2x^2-3x+2}< 7\) với \(\forall x\)\(\Leftrightarrow-2x^2+3x-2\le x^2+5x+m< 14x^2-21x+14\) với mọi x
\(\Leftrightarrow\left\{{}\begin{matrix}3x^2+2x+m+2\ge0;\forall x\left(1\right)\\13x^2-26x+14-m>0;\forall x\left(2\right)\end{matrix}\right.\)
Từ \(\left(1\right)\Leftrightarrow\left\{{}\begin{matrix}a=3>0\left(lđ\right)\\\Delta\le0\end{matrix}\right.\)\(\Leftrightarrow4-4.3\left(m+2\right)\le0\)\(\Leftrightarrow-20-12m\le0\)\(\Leftrightarrow m\ge\dfrac{-5}{3}\)
Từ \(\left(2\right)\Leftrightarrow\left\{{}\begin{matrix}a=13>0\left(lđ\right)\\\Delta< 0\end{matrix}\right.\)\(\Leftrightarrow m< 1\)
Vậy \(-\dfrac{5}{3}\le m< 1\)