Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
a) Do \(\pi< \alpha< \dfrac{3\pi}{2}\) nên \(sin\alpha< 0;cot\alpha>0;tan\alpha>0\).
Vì vậy: \(sin\alpha=-\sqrt{1-cos^2\alpha}=\dfrac{-\sqrt{15}}{4}\).
\(tan\alpha=\dfrac{sin\alpha}{cos\alpha}=\dfrac{-\sqrt{15}}{4}:\dfrac{-1}{4}=\sqrt{15}\).
\(cot\alpha=\dfrac{1}{tan\alpha}=\dfrac{1}{\sqrt{15}}\).
b) Do \(\dfrac{\pi}{2}< \alpha< \pi\) nên \(cos\alpha< 0;tan\alpha< 0;cot\alpha< 0\).
\(cos\alpha=-\sqrt{1-sin^2\alpha}=-\dfrac{\sqrt{5}}{3}\);
\(tan\alpha=\dfrac{2}{3}:\dfrac{-\sqrt{5}}{3}=\dfrac{-2}{\sqrt{5}}\); \(cot\alpha=1:tan\alpha=\dfrac{-\sqrt{5}}{2}\).
b) Do \(0< \alpha< \dfrac{\pi}{2}\) nên các giá trị lượng giác của \(\alpha\) đều dương.
Vì vậy:
\(cos\alpha=\sqrt{1-0,6^2}=\dfrac{4}{5}\).
\(tan\alpha=\dfrac{sin\alpha}{cos\alpha}=0,6:\dfrac{4}{5}=0,75;cot\alpha=1:tan\alpha=\dfrac{4}{3}\).
Do \(\dfrac{\pi}{2}< \alpha< \pi\) nên \(sin\alpha>0;tan\alpha< 0;cot\alpha< 0\).
\(sin\alpha=\sqrt{1-cos^2\alpha}=\dfrac{\sqrt{51}}{10}\).
\(tan\alpha=\dfrac{sin\alpha}{cos\alpha}=\dfrac{\sqrt{51}}{10}:\left(-0,7\right)=-\dfrac{\sqrt{51}}{7}\).
\(cot\alpha=\dfrac{1}{tan\alpha}=\dfrac{-7}{\sqrt{51}}\).
a)\(\sin^2\alpha+\cos^2\alpha=1\Rightarrow\sin^2\alpha=1-\cos^2\alpha\)
\(\Rightarrow1-2^2=-3\) \(\Rightarrow\cos=-\sqrt{3}\left(0< \alpha< \dfrac{\pi}{2}\right)\)
b) \(\tan\alpha\times\cot\alpha=1\Rightarrow\tan\alpha=\dfrac{1}{\cot\alpha}\Rightarrow\tan=\dfrac{1}{4}\)
a)Do \(0< \alpha< \dfrac{\pi}{2}\) nên các giá trị lượng giác của \(\alpha\) đều dương.
\(cos\alpha=2sin\alpha\)(1)
Nếu \(sin\alpha=0\Rightarrow cos\alpha\) (vô lý).
Vì vậy \(sin\alpha\ne0\) . Từ (1) \(\Rightarrow\dfrac{cos\alpha}{sin\alpha}=2\)\(\Leftrightarrow cot\alpha=2\).
Suy ra: \(tan\alpha=\dfrac{1}{2}\).
\(sin\alpha=\sqrt{\dfrac{1}{1+cot^2\alpha}}=\dfrac{1}{\sqrt{3}}\).
\(cos\alpha=\sqrt{1-sin^2\alpha}=\sqrt{\dfrac{2}{3}}\).
Do \(\dfrac{\pi}{2}< \alpha< \pi\) nên \(tan\alpha< 0,cot\alpha< 0;cos\alpha< 0\).
Vì vậy: \(cos\alpha=-\sqrt{1-sin^2\alpha}=-\dfrac{\sqrt{7}}{4}\).
\(tan\alpha=\dfrac{sin\alpha}{cos\alpha}=\dfrac{3}{4}:\dfrac{-\sqrt{7}}{4}=\dfrac{-3}{\sqrt{7}}\).
\(cot\alpha=\dfrac{1}{tan\alpha}=\dfrac{-\sqrt{7}}{3}\).
\(A=\dfrac{2tan\alpha-3cot\alpha}{cos\alpha+tan\alpha}\)\(=\dfrac{2.\dfrac{-3}{\sqrt{7}}-3.\dfrac{-\sqrt{7}}{3}}{\dfrac{-\sqrt{7}}{4}+\dfrac{-3}{\sqrt{7}}}\)
\(=\dfrac{\dfrac{-6}{\sqrt{7}}+\sqrt{7}}{\dfrac{-7-12}{4\sqrt{7}}}\)\(=\dfrac{\dfrac{-6+7}{\sqrt{7}}.4\sqrt{7}}{-19}\)\(=\dfrac{\dfrac{1}{\sqrt{7}}.4\sqrt{7}}{-19}=-\dfrac{4}{19}\).
b) \(\dfrac{cos^2\alpha+cot^2\alpha}{tan\alpha-cot\alpha}=\dfrac{\left(-\dfrac{\sqrt{7}}{4}\right)^2+\left(\dfrac{-\sqrt{7}}{3}\right)^2}{\dfrac{-3}{\sqrt{7}}+\dfrac{\sqrt{7}}{3}}\)
\(=\dfrac{\dfrac{7}{16}+\dfrac{7}{9}}{\dfrac{-9+7}{3\sqrt{7}}}=\dfrac{\dfrac{175}{144}}{\dfrac{-2}{3\sqrt{7}}}=\dfrac{-175}{96\sqrt{7}}\).
Với 0 < α < :
a) sin(α - π) < 0; b) cos( - α) < 0;
c) tan(α + π) > 0; d) cot(α + ) < 0
Vì \(0< \alpha< \dfrac{\pi}{2}\) nên các giá trị lượng giác của \(\alpha\) đều dương.
a) \(sin\left(\alpha-\pi\right)=-sin\left(\pi-\alpha\right)=-sin\alpha< 0\).
b) \(cos\left(\dfrac{3\pi}{2}-\alpha\right)=cos\left(\dfrac{3\pi}{2}-\alpha-2\pi\right)=cos\left(-\dfrac{\pi}{2}-\alpha\right)\)
\(=cos\left(\dfrac{\pi}{2}+\alpha\right)=-sin\alpha< 0\).
c) \(tan\left(\alpha+\pi\right)=tan\alpha>0\).
d) \(cot\left(\alpha+\dfrac{\pi}{2}\right)=-tan\alpha< 0\).
Do \(\pi< \alpha< \dfrac{3\pi}{2}\) nên \(sin\alpha,cos\alpha< 0;tan\alpha,cot\alpha< 0\).
\(cos\left(\alpha-\dfrac{\pi}{2}\right)=cos\left(\dfrac{\pi}{2}-\alpha\right)=sin\alpha< 0\).
\(sin\left(\dfrac{\pi}{2}+\alpha\right)=cos\alpha< 0\).
\(tan\left(\dfrac{3\pi}{2}-\alpha\right)=tan\left(\dfrac{3\pi}{2}-\alpha-2\pi\right)\)\(=tan\left(-\dfrac{\pi}{2}-\alpha\right)\)\(=-tan\left(\dfrac{\pi}{2}+\alpha\right)=cot\left(\alpha\right)>0\).
\(cot\left(\alpha+\pi\right)=cot\left(\alpha\right)>0\).
a) Do \(\pi< \alpha< \dfrac{3\pi}{2}\) nên \(tan\alpha,cot\alpha>0\) và \(sin\alpha,cos\alpha< 0\).
\(\left\{{}\begin{matrix}tan\alpha-3cot\alpha=6\\tan\alpha cot\alpha=1\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}tan\alpha=6+3cot\alpha\\\left(6+3cot\alpha\right)cot\alpha=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}tan\alpha=6+3cot\alpha\\3cot^2\alpha+6cot\alpha-1=0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}tan\alpha=6+3cot\alpha\\cot\alpha=\dfrac{-3+2\sqrt{3}}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}tan\alpha=3+2\sqrt{3}\\cot\alpha=\dfrac{-3+2\sqrt{3}}{3}\end{matrix}\right.\).
Có \(1+tan^2\alpha=\dfrac{1}{cos^2\alpha}\Rightarrow cos^2\alpha=\dfrac{1}{tan^2\alpha+1}\).
Có thể đề sai.
a) Do 0 < α <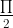
nên sinα > 0, tanα > 0, cotα > 0
sinα =
cotα =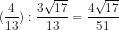
; tanα =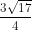 
b) π < α <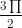
nên sinα < 0, cosα < 0, tanα > 0, cotα > 0
cosα = -√(1 - sin2 α) = -√(1 - 0,49) = -√0,51 ≈ -0,7141
tanα ≈ 0,9802; cotα ≈ 1,0202.
c)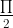
< α < π nên sinα > 0, cosα < 0, tanα < 0, cotα < 0
cosα =
≈ -0,4229.
sinα =
cotα = -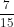
d) Vì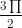
< α < 2π nên sinα < 0, cosα > 0, tanα < 0, cotα < 0
Ta có: tanα =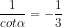
cosα =