TT
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
HP
28 tháng 8 2021
a, Vì \(-5sinx\ge-5\Rightarrow m-5sinx\ge0\forall x\Leftrightarrow m\ge5\)
b, Vì \(cos2x\ge-1\Rightarrow2m+cos2x\ge0\forall x\Leftrightarrow2m\ge1\Leftrightarrow m\ge\dfrac{1}{2}\)
c, TH1: \(m=0\) thỏa mãn yêu cầu bài toán
TH2: \(m>0\)
Khi đó: \(-m+1\le mcosx+1\le m+1\)
Yêu cầu bài toán thỏa mãn khi \(-m+1>0\Leftrightarrow m< 1\)
\(\Rightarrow0< m< 1\)
TH3: \(m< 0\)
Khi đó: \(m+1\le mcosx+1\le-m+1\)
Yêu cầu bài toán thỏa mãn khi \(m+1>0\Leftrightarrow m>-1\)
\(\Rightarrow-1< m< 0\)
Vậy \(m\in\left(-1;1\right)\)
HP
25 tháng 6 2021
1. \(D=R\)
2. \(sinx\ne0\Leftrightarrow x\ne k\pi\Rightarrow D=R\backslash\left\{k\pi|k\in R\right\}\)
3. \(cos2x\ne0\Leftrightarrow2x\ne\dfrac{\pi}{2}+k\pi\Leftrightarrow x\ne\dfrac{\pi}{4}+\dfrac{k\pi}{2}\Rightarrow D=R\backslash\left\{\dfrac{\pi}{4}+\dfrac{k\pi}{2}|k\in R\right\}\)
4. \(cos\left(x+\dfrac{\pi}{4}\right)\ne0\Leftrightarrow x+\dfrac{\pi}{4}\ne\dfrac{\pi}{2}+k\pi\Leftrightarrow x\ne\dfrac{\pi}{4}+k\pi\Rightarrow D=R\backslash\left\{\dfrac{\pi}{4}+k\pi|k\in R\right\}\)
PM
1
24 tháng 6 2021
cos2x ≠ 0
⇔ cos 2x ≠ \(\dfrac{\pi}{2}+k\pi\)
⇔ x ≠ \(\dfrac{\pi}{4}+k\dfrac{\pi}{2}\)
14 tháng 8 2023
1: ĐKXĐ: 3-cosx>0
=>cosx<3(luôn đúng)
2: ĐKXĐ: 1-sin 3x>=0
=>sin 3x<=1(luôn đúng)
3: ĐKXĐ: sin x<>0 và 2x<>pi/2+kpi
=>x<>kpi và x<>pi/4+kpi/2
4: ĐKXĐ: 2x-1>=0
=>x>=1/2
NV
Nguyễn Việt Lâm
Giáo viên
17 tháng 9 2021
Hàm xác định trên R khi với mọi x ta có:
\(sin^6x+cos^6x+m.sinx.cosx>0\)
\(\Leftrightarrow1-\dfrac{3}{4}sin^22x+\dfrac{m}{2}sin2x>0\)
\(\Leftrightarrow3sin^22x-2m.sin2x-4< 0\)
Đặt \(sin2x=t\in\left[-1;1\right]\Rightarrow3t^2-2mt-4< 0\)
\(\Leftrightarrow\left\{{}\begin{matrix}3.f\left(-1\right)< 0\\3.f\left(1\right)< 0\\\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}2m-1< 0\\-2m-1< 0\end{matrix}\right.\)
\(\Rightarrow-\dfrac{1}{2}< m< \dfrac{1}{2}\)
AH
Akai Haruma
Giáo viên
25 tháng 10 2021
Lời giải:
TXĐ: $1-\cos 2x\neq 0$
$\Leftrightarrow \cos 2x\neq 1$
$\Leftrightarrow 2x\neq 2k\pi$ với $k$ nguyên
$\Leftrightarrow x\neq k\pi $ với $k$ nguyên bất kỳ
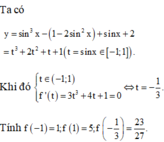
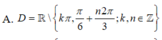
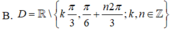
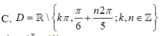
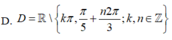
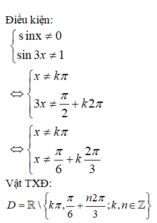
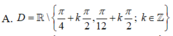
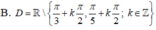
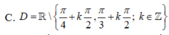
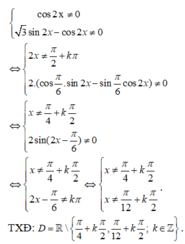
ta có hàm số \(y=\dfrac{sin3x}{cos2x-1}\) có nghĩa khi và chỉ khi \(cos2x-1\ne0\)
\(\Leftrightarrow cos2x\ne1\Leftrightarrow2x\ne k2\pi\Leftrightarrow x=k\pi\)
vậy tập xác định của hàm số là : \(D=R/\left\{k\pi\backslash k\in Z\right\}\)