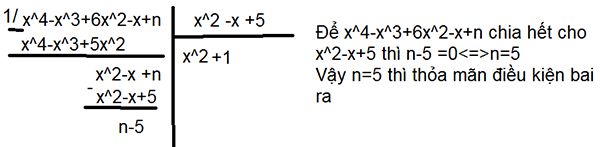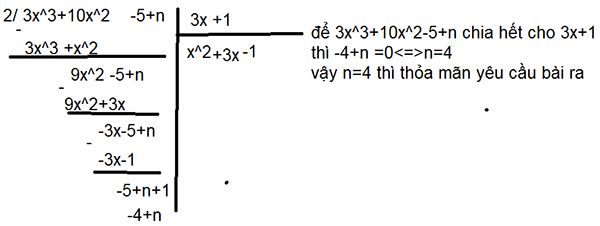HN
0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
31 tháng 1 2021
a, Theo bài ra ta có : M = N
hay \(\frac{2}{3}x-\frac{1}{3}=3x-2\left(x-1\right)\)
\(\Leftrightarrow\frac{2x-1}{3}=3x-2x+2\)
\(\Leftrightarrow\frac{2x-1}{3}=x+2\Leftrightarrow\frac{2x-1}{3}=\frac{3x+6}{3}\)
Khử mẫu : \(\Rightarrow2x-1=3x+6\Leftrightarrow-x-7=0\Leftrightarrow x=-7\)
b, Theo bài ra ta có : M + N = 8
hay \(\frac{2x}{3}-\frac{1}{3}+2x-2\left(x-1\right)=8\)
\(\Leftrightarrow\frac{2x-1}{3}+2x-2x+2=8\)
\(\Leftrightarrow\frac{2x-1}{3}-6=0\Leftrightarrow\frac{2x-1-18}{3}=0\Leftrightarrow2x-19=0\Leftrightarrow x=\frac{19}{2}\)
HY
9 tháng 2 2020
a. Để \(M=N\) thì \(\frac{2}{3}x-\frac{1}{3}=3x-2\left(x-1\right)\), ta có:
\(\frac{2}{3}x-\frac{1}{3}=3x-2\left(x-1\right)\\ \Leftrightarrow\frac{2}{3}x-\frac{1}{3}=3x-2x+2\\ \Leftrightarrow\frac{2}{3}x-3x+2x=\frac{1}{3}+2\\ \Leftrightarrow\frac{-1}{3}x=\frac{7}{3}\\ \Leftrightarrow x=-7\)
Vậy \(x=-7\) để \(M=N\)
b. Để \(M+N=8\) thì \(\frac{2}{3}x-\frac{1}{3}+\left[3x-2\left(x-1\right)\right]=8\), ta có:
\(\frac{2}{3}x-\frac{1}{3}+\left[3x-2\left(x-1\right)\right]=8\\\Leftrightarrow \frac{2}{3}x-\frac{1}{3}+\left[3x-2x+2\right]=8\\\Leftrightarrow \frac{2}{3}x-\frac{1}{3}+3x-2x+2=8\\ \Leftrightarrow\frac{2}{3}x+3x-2x=\frac{1}{3}-2+8\\\Leftrightarrow \frac{5}{3}x=\frac{19}{3}\\\Leftrightarrow x=\frac{19}{5}\)
Vậy \(x=\frac{19}{5}\) để \(M+N=8\)
DH
16 tháng 7 2017
Bài 2:
a, Sửa đề:
\(x^2-4=x^2+2x-2x-4=x\left(x+2\right)-2\left(x+2\right)\)
\(=\left(x+2\right)\left(x-2\right)\)
b, \(\left(x+2\right)\left(x+3\right)\left(x+4\right)\left(x+5\right)-24\)
\(=\left[\left(x+2\right)\left(x+5\right)\right]\left[\left(x+3\right)\left(x+4\right)\right]-24\)
\(=\left(x^2+5x+2x+10\right)\left(x^2+4x+3x+12\right)-24\)
\(=\left(x^2+7x+10\right)\left(x^2+7x+12\right)-24\)(1)
Đặt \(a=x^2+7x+10\Rightarrow a+2=x^2+7x+12\)
\(\Rightarrow\left(1\right)=a\left(a+2\right)-24=a^2+2a-24\)
\(=a^2-4a+6a-24=a.\left(a-4\right)+6.\left(a-4\right)\)
\(=\left(a-4\right)\left(a+6\right)\)(2)
Vì \(a=x^2+7x+10\) nên
\(\left(2\right)=\left(x^2+7x+10-4\right)\left(x^2+7x+10+6\right)\)
\(=\left(x^2+7x+6\right)\left(x^2+7x+16\right)\)
\(=\left(x^2+x+6x+6\right)\left(x^2+7x+16\right)\)
\(=\left[x.\left(x+1\right)+6.\left(x+1\right)\right]\left(x^2+7x+16\right)\)
\(=\left(x+1\right).\left(x+6\right)\left(x^2+7x+16\right)\)
Chúc bạn học tốt!!!
16 tháng 7 2017
1,
Dùng định lý Bơ du :
\(f\left(-\dfrac{1}{3}\right)=3\left(-\dfrac{1}{3}\right)^3+10\left(-\dfrac{1}{3}\right)^2+3.\left(-\dfrac{1}{3}\right)+a-5=0\)
\(=>a=5\)
Vậy a = 5 thì A chia hết cho B .
b,
M = \(x^2-4x+4y^2+4y+5\)
= \(\left(x^2-4x+4\right)+\left(4y^2+4y+1\right)+5-\left(1+4\right)\)
\(=\left(x-2\right)^2+\left(2y+1\right)^2+0\)
Vậy GTNN của M = 0
khi x = 2 ; 2y + 1 = 0 => y = 1/2
18 tháng 1 2019
a, Để M=N thì:
\(\dfrac{2}{3}x-\dfrac{1}{3}=3x-2\left(x-1\right)\\ \Leftrightarrow\dfrac{2}{3}x-\dfrac{1}{3}=3x-2x+2\\ \Leftrightarrow x-\dfrac{2}{3}x=2+\dfrac{1}{3}\\ \Leftrightarrow\dfrac{1}{3}x=\dfrac{7}{3}\\ \Leftrightarrow x=7\)
b, Để M+N=8 thì:
\(\dfrac{2}{3}x-\dfrac{1}{3}+3x-2x+2=8\) (mình làm tắt nhé :>)
\(\Leftrightarrow\dfrac{5}{3}x=8+\dfrac{5}{3}\)
\(\Leftrightarrow\dfrac{5}{3}x=\dfrac{29}{3}\)
\(\Leftrightarrow5x=29\\ \Leftrightarrow x=\dfrac{29}{5}\)
Chúc bạn học tốt nha
S
24 tháng 3 2020
mk gửi cho link:
https://lazi.vn/edu/exercise/tim-n-de-da-thuc-x4-x3-6x2-x-n-chia-het-cho-da-thuc-x2-x-5