
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

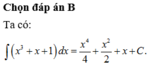

Biến đổi :
\(4\sin x+3\cos x=A\left(\sin x+2\cos x\right)+B\left(\cos x-2\sin x\right)=\left(A-2B\right)\sin x+\left(2A+B\right)\cos x\)
Đồng nhất hệ số hai tử số, ta có :
\(\begin{cases}A-2B=4\\2A+B=3\end{cases}\)\(\Leftrightarrow\begin{cases}A=2\\B=-1\end{cases}\)
Khi đó \(f\left(x\right)=\frac{2\left(\left(\sin x+2\cos x\right)\right)-\left(\left(\sin x-2\cos x\right)\right)}{\left(\sin x+2\cos x\right)}=2-\frac{\cos x-2\sin x}{\sin x+2\cos x}\)
Do đó,
\(F\left(x\right)=\int f\left(x\right)dx=\int\left(2-\frac{\cos x-2\sin x}{\sin x+2\cos x}\right)dx=2\int dx-\int\frac{\left(\cos x-2\sin x\right)dx}{\sin x+2\cos x}=2x-\ln\left|\sin x+2\cos x\right|+C\)

Chọn B.
Xét :
![]()
Có nghiệm bội chẵn x = - 1 , x = 1 nên dấu của f’(x) qua hai nghiệm này không đổi dấu => x = 1 và x = - 1 không là cực trị
Có nghiệm bội lẻ x = 2 , x = - 3 2 , nên nó là hai cực trị
Kết luận: Hàm số có hai cực trị.

1 ) f ( x ) = 1 3 + 2 x + 1 3 + 2 x = 1 3 + 2 x + 2 x 3 . 2 x + 1 = 4 x + 6 . 2 x + 1 3 . 4 x + 10 . 2 x + 3
⇒ f ' ( x ) = 2 . 4 x . ln 2 + 5 . 2 x . ln 2 3 . 4 x + 10 . 2 x + 3 3 . 4 x + 10 . 2 x + 3 2
- 6 . 4 x . ln 2 + 10 . 2 x . ln 2 4 x + 6 . 2 x + 1 3 . 4 x + 10 . 2 x + 3 2
= 2 . 2 x + 6 3 . 4 x + 10 . 2 x + 3 - 6 . 2 x + 10 4 x + 6 . 2 x + 1 3 . 4 x + 10 . 2 x + 3 2 . 2 x . ln 2 = - 8 . 4 x + 8 3 . 4 x + 10 . 2 x + 3 2 . 2 x . ln 2
f ' ( x ) = 0 ⇔ - 8 . 4 x + 8 = 0 ⇔ 4 x = 1 ⇔ x = 0
2 ) f ( x ) = 4 x + 6 . 2 x + 1 3 . 4 x + 10 . 2 x + 3
Ta có
f ( x ) - 1 3 = 4 x + 6 . 2 x + 1 3 . 4 x + 10 . 2 x + 3 - 1 = - 2 . 4 x - 4 . 2 x - 2 3 . 4 x + 10 . 2 x + 3 < 0 , ∀ x ⇒ f ( 1 ) + f ( 2 ) + . . + f ( 2017 ) < 1 + 1 + . . . + 1 = 2017 ⇒ f ( 1 ) + f ( 2 ) + . . + f ( 2017 = 2017 ⇒ 2 ) s a i
3) f ( x 2 ) = 1 3 + 2 x + 1 3 + 2 - x ⇒ f ( x 2 ) = 1 3 + 4 x + 1 3 + 4 - x l à s a i
Chọn đáp án A.