Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

b: Đặt \(x^2-6x-2=a\)
Theo đề, ta có: \(a+\dfrac{14}{a+9}=0\)
=>(a+2)(a+7)=0
\(\Leftrightarrow\left(x^2-6x\right)\left(x^2-6x+5\right)=0\)
=>x(x-6)(x-1)(x-5)=0
hay \(x\in\left\{0;1;6;5\right\}\)
c: \(\Leftrightarrow\dfrac{-8x^2}{3\left(2x-1\right)\left(2x+1\right)}=\dfrac{2x}{3\left(2x-1\right)}-\dfrac{8x+1}{4\left(2x+1\right)}\)
\(\Leftrightarrow-32x^2=8x\left(2x+1\right)-3\left(8x+1\right)\left(2x-1\right)\)
\(\Leftrightarrow-32x^2=16x^2+8x-3\left(16x^2-8x+2x-1\right)\)
\(\Leftrightarrow-48x^2=8x-48x^2+18x+3\)
=>26x=-3
hay x=-3/26

\(B=x^2-6x+y^2-2y+12=\left(x^2-6x+9\right)\left(y^2-2y+1\right)+2\)
\(B=\left(x-3\right)^2+\left(y-1\right)^2+2\text{ }\)
Ta thấy B lớn hơn hoặc bằng 2 suy ra GTNN của B là 2
Dấu = xảy ra khi x=3; y=1
\(C=2x^2-6x=\left(2x^2-6x+4,5\right)-4,5=2\left(x^2-3x+2,25\right)-4,5\)
\(C=2\left(x-1,5\right)^2-4,5\)
Ta thấy C luôn luôn lớn hơn hoặc bằng -4,5 nên GTNN của C là -4,5
Dấu = xảy ra khi x=1,5
Tối mình full cho còn giờ mình đi đá bóng đây
1) \(D=\frac{2016}{-4x^2+4x-5}\). Để D đạt giá trị nhỏ nhất suy ra \(-4x^2+4x-5\)đạt giá trị lớn nhất.
Ta có \(-4x^2+4x-5=-4x^2+4x-1-4=\left(-4x^2+4x-1\right)-4\)
\(-4\left(x^2-x+\frac{1}{4}\right)-4=-4\left(x-\frac{1}{2}\right)^2-4\).
Ta Thấy:\(-4\left(x-\frac{1}{2}\right)^2\) bé hơn hoặc bằng 0 nên \(-4\left(x-\frac{1}{2}\right)^2-4\)bé hơn hoặc bằng -4
nên ..... bạn tự kết luận

\(A=x^2+3x+7\)
\(=x^2+2.1,5x+2,25+4,75\)
\(=\left(x+1,5\right)^2+4,75\ge4,75\)
Vậy \(A_{min}=4,75\Leftrightarrow x=-1,5\)
\(B=2x^2-8x\)
\(=2\left(x^2-4x\right)\)
\(=2\left(x^2-4x+4-4\right)\)
\(=2\left[\left(x-2\right)^2-4\right]\)
\(=2\left(x-2\right)^2-8\ge-8\)
Vậy \(B_{min}=-8\Leftrightarrow x=2\)

a) 4x -8 ≥ 3(3x-1)-2x +1
⇒4x -8 ≥7x -2
⇒4x -7x ≥ -2 +8
⇒-3x ≥ 6
⇒x≤-2
Vậy bpt có nghiệm là:{x|x≤-2}
b) (x-3)(x+2)+(x+4)2≤ 2x (x+5)+4
⇔ x2+2x - 3x - 6 +x2 + 8x +16≤ 2x2 + 10x +4
⇔ x2 +2x - 3x + x2 + 8x - 2x2- 10x ≤ 4+6-16
⇔ -3x ≤ -6
⇔ x≥ 2
Vậy bpt có tập nghiệm là: {x|x≥2}

Bài 17)
(x - 2)^4 + (x - 6)^4 = 82
Đặt t = x + 3
=> x + 2 = t - 1; x + 4 = t + 1.
ta có pt: (t - 1)^4 + (t + 1)^4 = 82
<=>[(t -1)²]² + [(t + 1)²]² = 82
<=> (t² - 2t + 1)² + (t² + 2t + 1)² = 82
<=> (t²+1)² - 4t(t²+1) + 4t² + (t²+1)² + 4t(t²+1) + 4t² = 82
<=> (t² + 1)² + 4t² = 41
<=> t^4 + 6t² + 1 = 41
<=> (t²)² + 6t² - 40 = 0
<=> t² = -10 (loại) hoặc t² = 4
<=> t = 2 hoặc t = -2
với t = -2 => x = -5
với t = 2 => x = -1
vậy pt có hai nghiệm là : x = -1 hoặc x = -5
Bài 18: Phương trình đã cho được viết thành: $${({x^2} + 6x + 10)^2} + (x + 3)\left[ {3\left( {{x^2} + 6x + 10} \right) + 2\left( {x + 3} \right)} \right] = 0$$
Đặt $u = {x^2} + 6x + 10 > 0,v = x + 3$, suy ra:
$${u^2} + v\left( {3u + 2v} \right) = 0 \Leftrightarrow \left( {u + v} \right)\left( {u + 2v} \right) = 0 \Leftrightarrow \left[ \begin{gathered}
u + v = 0 \\
u + 2v = 0 \\
\end{gathered} \right.$$
$$ \Leftrightarrow \left[ \begin{gathered}
{x^2} + 6x + 10 + x + 3 = 0 \\
{x^2} + 6x + 10 + 2\left( {x + 3} \right) = 0 \\
\end{gathered} \right. \Leftrightarrow \left[ \begin{gathered}
{x^2} + 7x + 13 = 0 \\
{x^2} + 8x + 16 = 0 \\
\end{gathered} \right. \Leftrightarrow x = - 4$$

1) điều kiện xác định : \(x\notin\left\{-1;-2;-3;-4\right\}\)
ta có : \(\dfrac{1}{x^2+3x+2}+\dfrac{1}{x^2+5x+6}+\dfrac{1}{x^2+7x+12}=\dfrac{1}{6}\)
\(\Leftrightarrow\dfrac{1}{\left(x+1\right)\left(x+2\right)}+\dfrac{1}{\left(x+2\right)\left(x+3\right)}+\dfrac{1}{\left(x+3\right)\left(x+4\right)}=\dfrac{1}{6}\) \(\Leftrightarrow\dfrac{\left(x+3\right)\left(x+4\right)+\left(x+1\right)\left(x+4\right)+\left(x+1\right)\left(x+2\right)}{\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)}=\dfrac{1}{6}\)\(\Leftrightarrow\dfrac{x^2+7x+12+x^2+5x+4+x^2+3x+2}{\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)}=\dfrac{1}{6}\)
\(\Leftrightarrow\dfrac{3x^2+15x+18}{\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)}=\dfrac{1}{6}\)
\(\Leftrightarrow6\left(3x^2+15x+18\right)=\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)\)
\(\Leftrightarrow18\left(x^2+5x+6\right)=\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)\)
\(\Leftrightarrow18\left(x+2\right)\left(x+3\right)=\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)\)
\(\Leftrightarrow18=\left(x+1\right)\left(x+4\right)\) ( vì điều kiện xác định )
\(\Leftrightarrow18=x^2+5x+4\Leftrightarrow x^2+5x-14=0\)
\(\Leftrightarrow\left(x-2\right)\left(x+7\right)=0\Leftrightarrow\left[{}\begin{matrix}x-2=0\\x+7=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-7\end{matrix}\right.\left(tmđk\right)\)
vậy \(x=2\) hoặc \(x=-7\) mấy câu kia lm tương tự nha bn
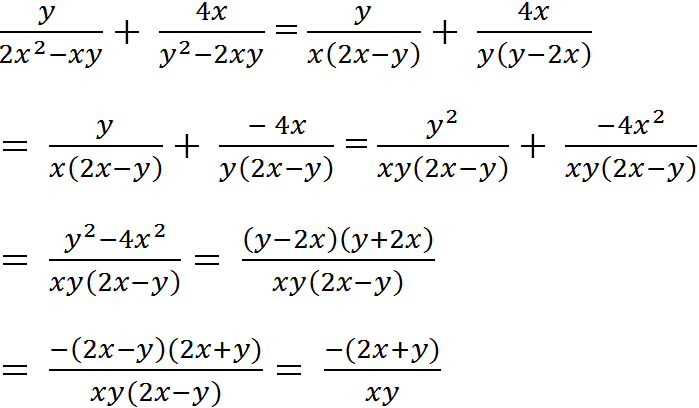
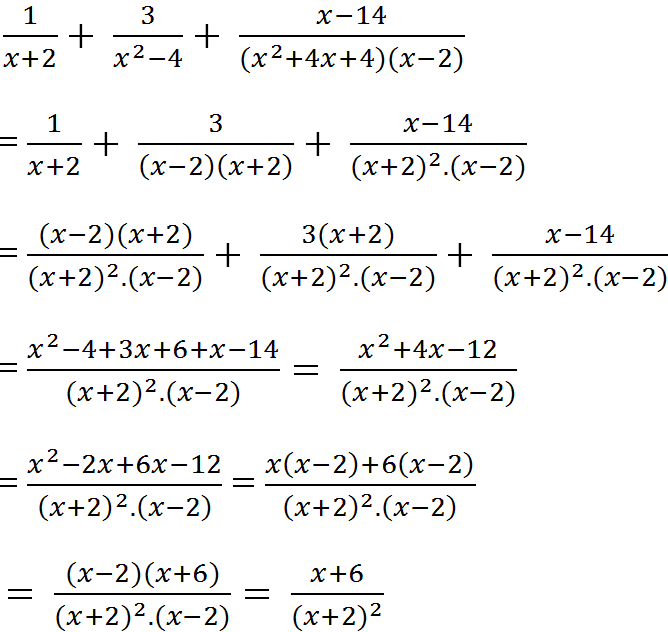
Lời giải:
Chắc bạn nhầm giữa GTLN và GTNN. Ba biểu thức này chỉ tìm đc min thôi nhé.
Biểu thức 1:
\(A=4x^2+4x+2016=(2x+1)^2+2015\)
Nhận thấy với \(x\in\mathbb{R}\Rightarrow (2x+1)^2\geq 0\Rightarrow (2x+1)^2+2015\geq 2015\)
Do đó \(A_{\min}=2015\Leftrightarrow x=-\frac{1}{2}\)
Biểu thức 2:
\(B=\frac{-7}{x^2+6x+2012}\)
Ta có \(x^2+6x+2012=(x+3)^2+2003\)
Thấy rằng \((x+3)^2\geq 0\forall x\in\mathbb{R}\Rightarrow (x+3)^2+2003\geq 2003\)
\(\Rightarrow \frac{1}{x^2+6x+2012}\leq \frac{1}{2003}\Rightarrow \frac{-7}{x^2+6x+2012}\geq \frac{-7}{2003}\)
\(\Rightarrow B_{\min}=\frac{-7}{2003}\Leftrightarrow x=-3\)
Biểu thức 3:
\(C=(x-1)(x+3)(x+2)(x+6)\)
\(\Leftrightarrow C=[(x-1)(x+6)][(x+2)(x+3)]\)
\(\Leftrightarrow C=(x^2+5x-6)(x^2+5x+6)\)
Đặt \(x^2+5x-6=t\Rightarrow C=t(t+12)=(t+6)^2-36\geq 0-36\)
\(\Leftrightarrow C\geq -36\)
Vậy \(C_{\min}=-36\Leftrightarrow t=-6\Leftrightarrow x^2+5x-6=-6\Leftrightarrow x=0\) hoặc \(x=-5\)