
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(B=\left(x^2+5x+5\right)\left[\left(x+2\right)\left(x+3\right)+1\right]\)
\(=\left(x^2+5x+5\right)\left(x^2+5x+7\right)\)
Đặt \(x^2+5x+6=t\) nên \(B=\left(t-1\right)\left(t+1\right)=t^2-1\ge-1\forall t\) có GTNN là - 1
Dấu "=" xảy ra \(\Leftrightarrow x^2+5x+6=0\Leftrightarrow\left(x+3\right)\left(x+2\right)=0\Rightarrow\orbr{\begin{cases}x=-3\\x=-2\end{cases}}\)
Vậy \(B_{min}=-1\) tại \(\orbr{\begin{cases}x=-3\\x=-2\end{cases}}\)

\(A=x\left(x+1\right)\left(x^2+x-4\right)=\left(x^2+x\right)\left(x^2+x-4\right)\)
Đặt \(x^2+x=a\) nên \(A=a\left(a-4\right)=a^2-4a+4-4=\left(a-2\right)^2-4\ge-4\)
Dấu "=" xảy ra \(\Leftrightarrow a-2=0\Leftrightarrow x^2+x-2=0\Leftrightarrow\left(x-1\right)\left(x+2\right)=0\Rightarrow\orbr{\begin{cases}x=1\\x=-2\end{cases}}\)
Vậy \(A_{min}=-4\) tại \(\orbr{\begin{cases}x=1\\x=-2\end{cases}}\)
B;C tương tự

a) A= x2 + 4x + 5
=x2+4x+4+1
=(x+2)2+1≥0+1=1
Dấu = khi x+2=0 <=>x=-2
Vậy Amin=1 khi x=-2
b) B= ( x+3 ) ( x-11 ) + 2016
=x2-8x-33+2016
=x2-8x+16+1967
=(x-4)2+1967≥0+1967=1967
Dấu = khi x-4=0 <=>x=4
Vậy Bmin=1967 <=>x=4
Bài 2:
a) D= 5 - 8x - x2
=-(x2+8x-5)
=21-x2+8x+16
=21-x2+4x+4x+16
=21-x(x+4)+4(x+4)
=21-(x+4)(x+4)
=21-(x+4)2≤0+21=21
Dấu = khi x+4=0 <=>x=-4
Bài 1:
c)C=x2+5x+8
=x2+5x+\(\left(\dfrac{5}{2}\right)^2\)+\(\dfrac{7}{4}\)
=\(\left(x+\dfrac{5}{2}\right)^2\)+\(\dfrac{7}{4}\)\(\ge\dfrac{7}{4}\)
Vậy \(C_{min}=\dfrac{7}{4}\Leftrightarrow x=-\dfrac{5}{2}\)

My Nguyễn ơi,bạn truy cập vào đường link này để tìm câu hỏi tương tự của câu a/Bài 1 nhé
https://vn.answers.yahoo.com/question/index?qid=20110206184834AAokV5m&sort=N

2, TC: \(\frac{5x^2-4x+4}{x^2}=\frac{4x^2+x^2-4x+4}{x^2}\)\(=\frac{4x^2}{x^2}+\frac{\left(x-2\right)^2}{x^2}=4+\frac{\left(x-2\right)^2}{x^2}\)
Ta có \(\frac{\left(x-2\right)^2}{x^2}\ge0\forall x\left(x\ne0\right)\)\(\Rightarrow4+\frac{\left(x-2\right)^2}{x^2}\ge4\)
Vậy GTNN của A là 4 tại \(\frac{\left(x-2^2\right)}{x^2}=0\Rightarrow x=2\)

Ta có:B=(x-1/x+2)+(2-5x/4-x^2)
=[(x-1)*(x-2)/(x+2)-(2-5x)/(x-2)*(x+2)]
=(x^2+2x)/(x-2)*(x+2)
=x/(x-2)
=> 5B=5x/(x-2)
=>A-5B = (x^3+2/x-2)-(5x/x-2)=x^3-5x+2/x-2=(x-2)*(x^2+2x-1)/(x-2)=x^2+2x-1=(x+1)^2-2
vì (x+1)^2>= 0
=> A-5B= (x+1)^2-2>= -2
Dấu `=' xảu ra<=> (x+1)^2 =0
=>x=-1
vậy GTNN của P=-2 <=> x=-1
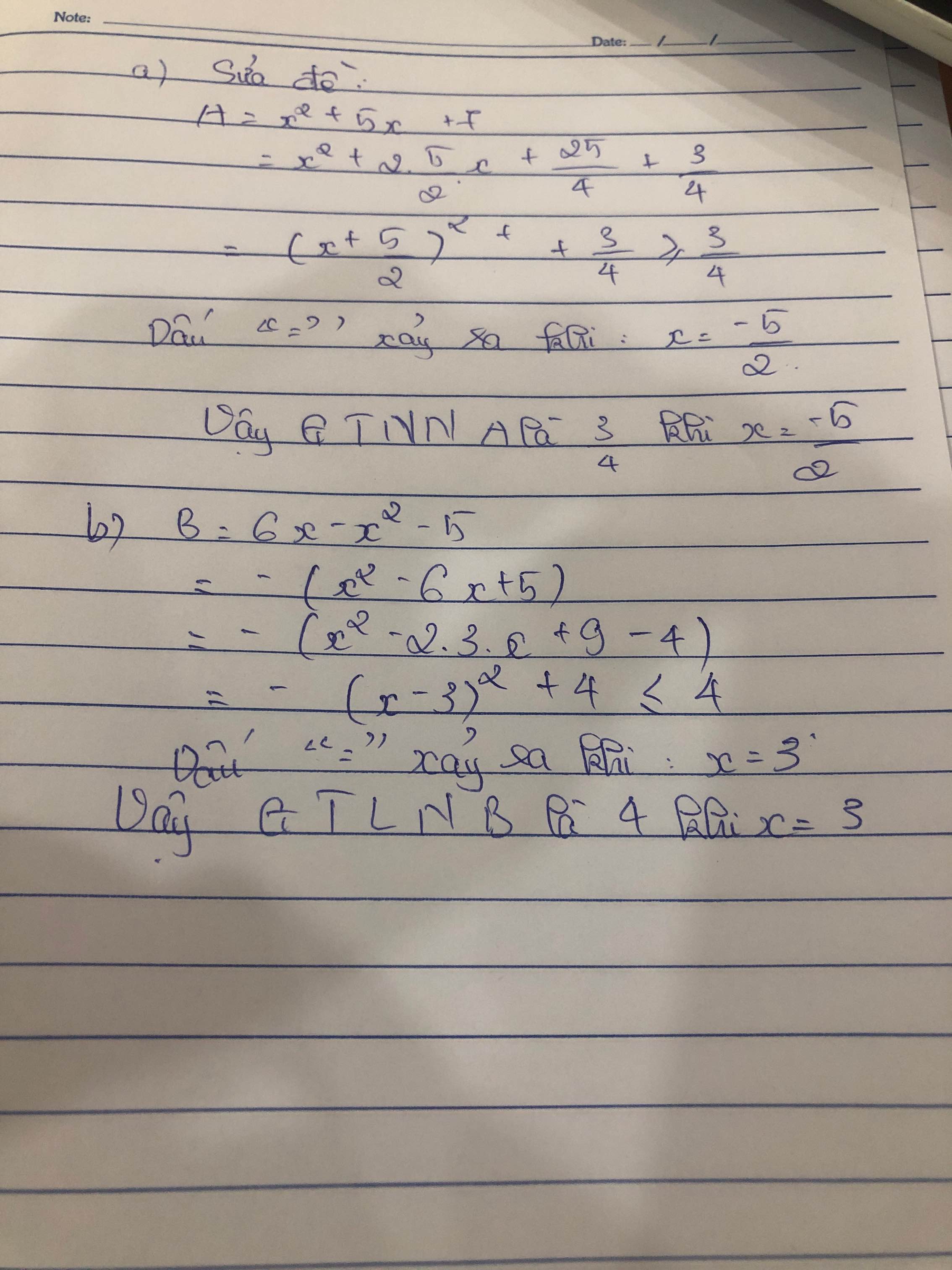
\(B=\left(x^2+5x+5\right)\left[\left(x+2\right)\left(x+3\right)+1\right]\)
\(=\left(x^2+5x+5\right)\left(x^2+5x+7\right)\)
\(=\left(x^2+5x+5\right)^2+2\left(x^2+5x+5\right)+1-1\)
\(=\left(x^2+5x+6\right)^2-1\ge-1\)
Vậy GTNN là - 1
Dấu = xảy ra khi \(x^2+5x+6=0\)
\(\Leftrightarrow\left(x^2+3x\right)+\left(2x+6\right)=0\)
\(\Leftrightarrow\left(x+3\right)\left(x+2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=-3\\x=-2\end{cases}}\)