Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

1) a) Căn thức có nghĩa \(\Leftrightarrow4-2x\ge0\Leftrightarrow2x\le4\Leftrightarrow x\le2\)
b) Thay x = 2 vào biểu thức A, ta được: \(A=\sqrt{4-2.2}=\sqrt{0}=0\)
Thay x = 0 vào biểu thức A, ta được: \(A=\sqrt{4-2.0}=\sqrt{4}=2\)
Thay x = 1 vào biểu thức A, ta được: \(A=\sqrt{4-2.1}=\sqrt{2}\)
Thay x = -6 vào biểu thức A, ta được: \(A=\sqrt{4-2.\left(-6\right)}=\sqrt{16}=4\)
Thay x = -10 vào biểu thức A, ta được: \(A=\sqrt{4-2.\left(-10\right)}=\sqrt{24}=2\sqrt{6}\)
c) \(A=0\Leftrightarrow\sqrt{4-2x}=0\Leftrightarrow4-2x=0\Leftrightarrow x=2\)
\(A=5\Leftrightarrow\sqrt{4-2x}=5\Leftrightarrow4-2x=25\Leftrightarrow x=\frac{-21}{2}\)
\(A=10\Leftrightarrow\sqrt{4-2x}=10\Leftrightarrow4-2x=100\Leftrightarrow x=-48\)

(1)^2=> a^2+b^2+c^2+2ab+2bc+2ac=0
=> ab+bc+ac=-2
(...)^2=4
(ab)^2+(bc)^2+(ac)^2=4
(2)^2=>A+2(ab)^2+2(bc)^2+2(ac)^2=16
A=16-4=12
nhầm giờ mới có máy tính
\(\left(a^2+b^2+c^2\right)^2=A+2\left(\left(ab\right)^2+\left(bc\right)^2+\left(ac\right)^2\right)=16\)
\(A=16-2.4=8\)

\(1-2x\ge0\Leftrightarrow2x\le1\Leftrightarrow x\le\frac{1}{2}\)
\(x-\sqrt{1-2x}>0\)
X>0
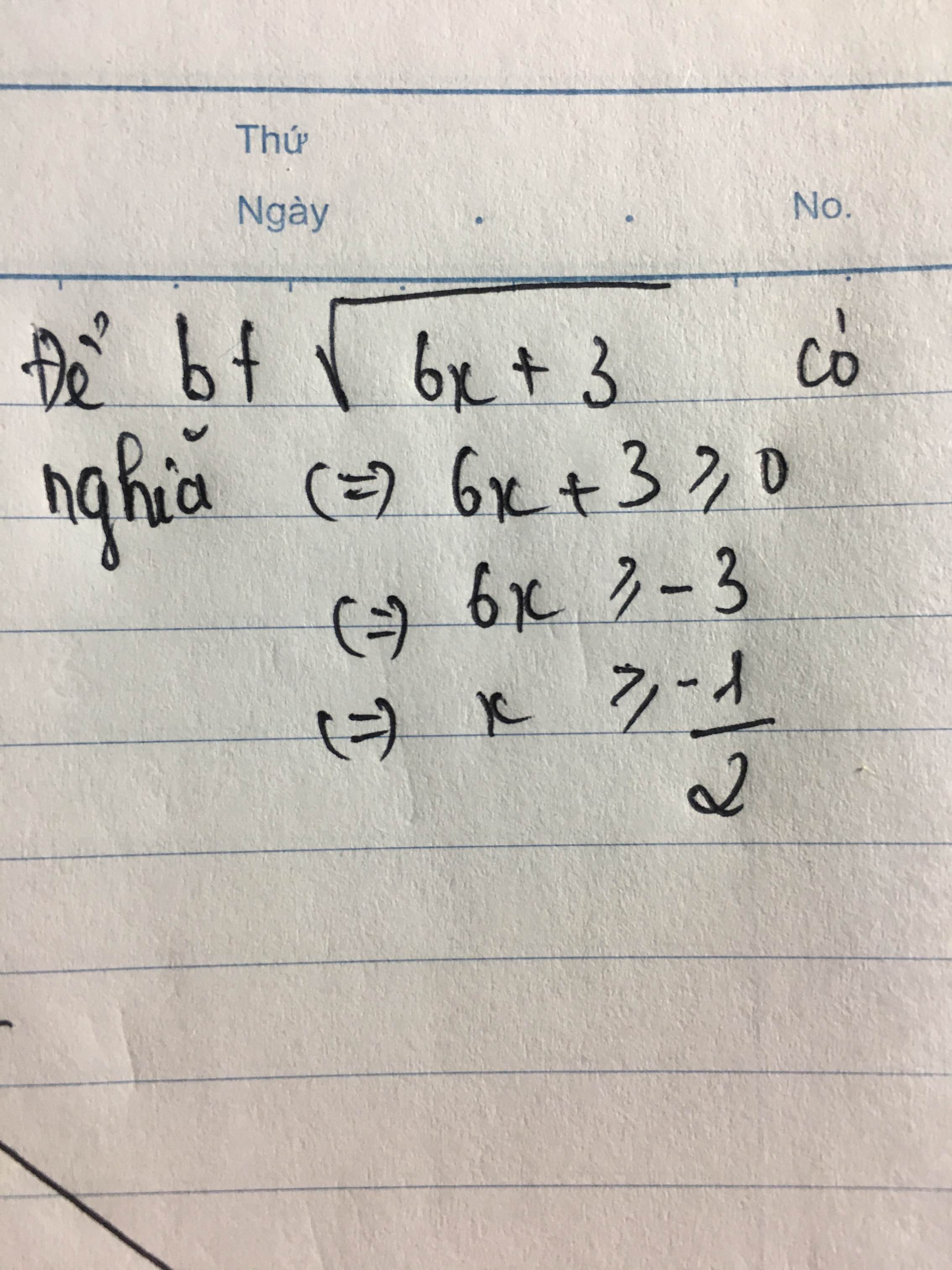
câu này hay thế!
a) \(\sqrt{9-a^2}\)
Để biểu thức xác định thì \(9-a^2\ge0\Leftrightarrow a^2\le9\Leftrightarrow-3\le a\le3\)
b) \(\sqrt{a^2-4}\)
Để biểu thức xác định thì \(a^2-4\ge0\Leftrightarrow a^2\ge4\Leftrightarrow\left[{}\begin{matrix}a\ge2\\a\le-2\end{matrix}\right.\)