Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(M=a^4+a^3+a^2-a^3-a^2-a-5a^2-5a-5\)
\(M=a^2\left(a^2+a+1\right)-a\left(a^2+a+1\right)-5\left(a^2+a+1\right)\)
\(M=\left(a^2+a+1\right)\left(a^2-a-5\right)\)
M là số nguyên tố khi và chỉ khi \(a^2+a+1\) là SNT và \(a^2-a-5=1\)
\(\Rightarrow a^2-a-6=0\Rightarrow\left[{}\begin{matrix}a=3\\a=-2\left(loại\right)\end{matrix}\right.\)
Thay \(a=3\) vào ta được \(a^2+a+1=13\) là SNT (thỏa mãn)
Vậy \(a=3\)

Câu 1:
a) \(A=\left[\dfrac{2}{3x}-\dfrac{2}{x+1}.\left(\dfrac{x+1}{3x}-x-1\right)\right]:\dfrac{x-1}{x}\)
\(=\left[\dfrac{2}{3x}-\dfrac{2}{3x}+\dfrac{2x}{x+1}+\dfrac{2}{x+1}\right]\dfrac{x}{x-1}\)
\(=\left[\dfrac{2x}{x+1}+\dfrac{2}{x+1}\right]\dfrac{x}{x-1}\)
\(=\dfrac{2x+2}{x+1}.\dfrac{x}{x-1}\)
\(=\dfrac{2\left(x+1\right)}{x+1}.\dfrac{x}{x-1}\)
\(=2.\dfrac{x}{x-1}\)
\(=\dfrac{2x}{x-1}\)
Câu 1:
ĐKXĐ: \(x\notin\left\{0;-1;1\right\}\)
a) Ta có: \(A=\left(\dfrac{2}{3x}-\dfrac{2}{x+1}\cdot\left(\dfrac{x+1}{3x}-x-1\right)\right):\dfrac{x-1}{x}\)
\(=\left(\dfrac{2}{3x}-\dfrac{2}{x+1}\cdot\left(\dfrac{x+1}{3x}-\dfrac{3x\left(x+1\right)}{3x}\right)\right):\dfrac{x-1}{x}\)
\(=\left(\dfrac{2}{3x}-\dfrac{2}{x+1}\cdot\dfrac{x+1-3x^2-3x}{3x}\right):\dfrac{x-1}{x}\)
\(=\left(\dfrac{2}{3x}-\dfrac{2}{x+1}\cdot\dfrac{-3x^2-2x+1}{3x}\right):\dfrac{x-1}{x}\)
\(=\left(\dfrac{2\left(x+1\right)}{3x\left(x+1\right)}-\dfrac{2\cdot\left(-3x^2-2x+1\right)}{3x\left(x+1\right)}\right):\dfrac{x-1}{x}\)
\(=\dfrac{2x+2+6x^2+4x-2}{3x\left(x+1\right)}:\dfrac{x-1}{x}\)
\(=\dfrac{6x^2+6x}{3x\left(x+1\right)}:\dfrac{x-1}{x}\)
\(=\dfrac{6x\left(x+1\right)}{3x\left(x+1\right)}:\dfrac{x-1}{x}\)
\(=2\cdot\dfrac{x}{x-1}=\dfrac{2x}{x-1}\)
b) Để A nguyên thì \(2x⋮x-1\)
\(\Leftrightarrow2x-2+2⋮x-1\)
mà \(2x-2⋮x-1\)
nên \(2⋮x-1\)
\(\Leftrightarrow x-1\inƯ\left(2\right)\)
\(\Leftrightarrow x-1\in\left\{1;-1;2;-2\right\}\)
\(\Leftrightarrow x\in\left\{2;0;3;-1\right\}\)
Kết hợp ĐKXĐ, ta được: \(x\in\left\{2;3\right\}\)
Vậy: Để A nguyên thì \(x\in\left\{2;3\right\}\)

\(B=\left(n+3\right)^2-\left(n-4\right)^2\)
\(=\left(n+3-n+4\right)\left(n+3+n-4\right)\)
\(=7\left(2n-1\right)\)
Dễ thấy B là số nguyên tố khi
\(2n-1=1\Leftrightarrow n=1\)
Vậy n = 1 thì B là số nguyên tố

1: \(D=\dfrac{1}{x+4}+\dfrac{x}{x-4}+\dfrac{24-x^2}{x^2-16}\)
\(=\dfrac{1}{x+4}+\dfrac{x}{x-4}+\dfrac{24-x^2}{\left(x+4\right)\left(x-4\right)}\)
\(=\dfrac{x-4+x\left(x+4\right)+24-x^2}{\left(x+4\right)\left(x-4\right)}\)
\(=\dfrac{-x^2+x+20+x^2+4x}{\left(x+4\right)\left(x-4\right)}=\dfrac{5x+20}{\left(x+4\right)\left(x-4\right)}\)
\(=\dfrac{5\left(x+4\right)}{\left(x+4\right)\left(x-4\right)}=\dfrac{5}{x-4}\)
2: Khi x=10 thì \(D=\dfrac{5}{10-4}=\dfrac{5}{6}\)
3: \(M=\left(x-2\right)\cdot D=\dfrac{5\left(x-2\right)}{x-4}\)
Để M là số nguyên thì \(5\cdot\left(x-2\right)⋮x-4\)
=>\(5\left(x-4+2\right)⋮x-4\)
=>\(5\left(x-4\right)+10⋮x-4\)
=>\(10⋮x-4\)
=>\(x-4\in\left\{1;-1;2;-2;5;-5;10;-10\right\}\)
=>\(x\in\left\{5;3;6;2;9;-1;14;-6\right\}\)
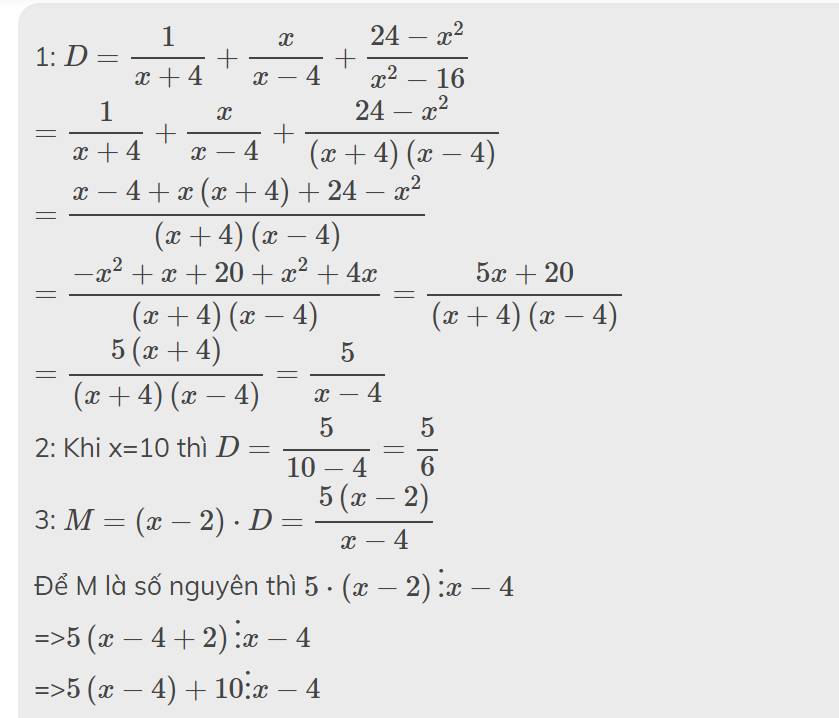
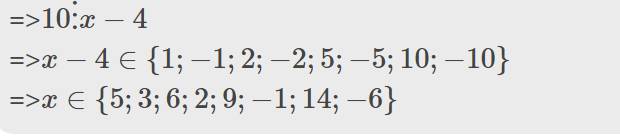
ai do tra loi di ma