Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(A=\left(\dfrac{1}{x+2\sqrt{x}}-\dfrac{1}{\sqrt{x}+2}\right):\dfrac{1-\sqrt{x}}{x+4\sqrt{x}+4}\left(x>0;x\ne1\right)\)
\(A=\left[\dfrac{1}{\sqrt{x}\left(\sqrt{x}+2\right)}-\dfrac{\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+2\right)}\right]:\dfrac{1-\sqrt{x}}{\left(\sqrt{x}\right)^2+2\cdot\sqrt{x}\cdot2+2^2}\)
\(A=\dfrac{1-\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+2\right)}:\dfrac{1-\sqrt{x}}{\left(\sqrt{x}+2\right)^2}\)
\(A=\dfrac{1-\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+2\right)}\cdot\dfrac{\left(\sqrt{x}+2\right)^2}{1-\sqrt{x}}\)
\(A=\dfrac{\left(\sqrt{x}+2\right)^2}{\sqrt{x}\left(\sqrt{x}+2\right)}\)
\(A=\dfrac{\sqrt{x}+2}{\sqrt{x}}\)
Vậy: ...

\(ĐK:x\ge0;x\ne1;x\ne4\\ P=\dfrac{\sqrt{x}+1-\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}:\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)-\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}-2\right)}\\ P=\dfrac{2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\cdot\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}-2\right)}{x-1-x+4}\\ P=\dfrac{2\left(\sqrt{x}-2\right)}{3\left(\sqrt{x}+1\right)}=\dfrac{2\sqrt{x}-4}{3\sqrt{x}+3}\)

a: \(A=\sqrt{x}+1+\sqrt{x}+1=2\sqrt{x}+2\)
b: Để A=6 thì \(2\sqrt{x}+2=6\)
=>x=4

a) \(P=\left(3-\dfrac{3}{\sqrt{x}-1}\right):\left(\dfrac{x+2}{x+\sqrt{x}-2}-\dfrac{\sqrt{x}}{\sqrt{x}+2}\right)\)
\(=\left(\dfrac{3\left(\sqrt{x}-1\right)-3}{\sqrt{x}-1}\right):\left[\dfrac{x+2}{\left(\sqrt{x}-1\right)\left(\sqrt{x+2}\right)}-\dfrac{\sqrt{x}}{\sqrt{x}+2}\right]\)
\(=\dfrac{3\sqrt{x}-3-3}{\sqrt{x}-1}:\dfrac{x+2-\sqrt{x}\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{3\sqrt{x}-6}{\sqrt{x}-1}:\dfrac{x+2-x+\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{3\sqrt{x}-6}{\sqrt{x}-1}:\dfrac{\sqrt{x}+2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{3\sqrt{x}-6}{\sqrt{x}-1}:\dfrac{1}{\sqrt{x}-1}\)
\(=\dfrac{3\sqrt{x}-6}{\sqrt{x}-1}.\left(\sqrt{x}-1\right)\)
\(=3\sqrt{x}-6\)
b) \(P=\dfrac{4\sqrt{x}-1}{\sqrt{x}}\)
\(\Leftrightarrow3\sqrt{x}-6=\dfrac{4\sqrt{x}-1}{\sqrt{x}}\) (1)
ĐKXĐ: \(x>0\)
\(\left(1\right)\Leftrightarrow3x-6\sqrt{x}=4\sqrt{x}-1\)
\(\Leftrightarrow3x-6\sqrt{x}-4\sqrt{x}+1=0\)
\(\Leftrightarrow3x-10\sqrt{x}+1=0\) (2)
Đặt \(t=\sqrt{x}\ge0\)
\(\left(2\right)\Leftrightarrow3t^2-10t+1=0\)
\(\Delta'=25-4=22\)
Phương trình có hai nghiệm phân biệt:
\(t_1=\dfrac{5+\sqrt{22}}{3}\) (nhận)
\(t_2=\dfrac{5-\sqrt{22}}{3}\) (nhận)
Với \(t=\dfrac{5+\sqrt{22}}{3}\) \(\Leftrightarrow\sqrt{x}=\dfrac{5+\sqrt{22}}{3}\Leftrightarrow x=\dfrac{47+10\sqrt{22}}{9}\) (nhận)
Với \(t=\dfrac{5-\sqrt{22}}{3}\Leftrightarrow\sqrt{x}=\dfrac{5-\sqrt{22}}{3}\Leftrightarrow x=\dfrac{47-10\sqrt{22}}{9}\) (nhận)
Vậy \(x=\dfrac{47+10\sqrt{22}}{9};x=\dfrac{47-10\sqrt{22}}{9}\) thì \(P=\dfrac{4\sqrt{x}-1}{\sqrt{x}}\)
a: \(P=\dfrac{3\sqrt{x}-3-3}{\sqrt{x}-1}:\dfrac{x+2-x+\sqrt{x}}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{3\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}{\sqrt{x}+2}=3\sqrt{x}-6\)
b: P=(4căn x-1)/căn x
=>3x-6căn x-4căn x+1=0
=>3x-10căn x+1=0
=>x=(47+10căn 22)/9 hoặc x=(47-10căn 22)/9

\(B=\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)\cdot\dfrac{\left(\sqrt{x}+1\right)}{\sqrt{x}-1}=\left(\sqrt{x}+1\right)^2\)

Lời giải:
1. \(P=\left[\frac{1}{\sqrt{x}(\sqrt{x}-1)}+\frac{\sqrt{x}}{\sqrt{x}(\sqrt{x}-1)}\right]:\frac{x}{(\sqrt{x}-1)^2}\)
\(=\frac{\sqrt{x}+1}{\sqrt{x}(\sqrt{x}-1)}.\frac{(\sqrt{x}-1)^2}{x}=\frac{(\sqrt{x}+1)(\sqrt{x}-1)}{x\sqrt{x}}=\frac{x-1}{x\sqrt{x}}\)
2.
\(P>\frac{1}{2}\Leftrightarrow \frac{x-1}{x\sqrt{x}}> \frac{1}{2}\)
\(\Leftrightarrow \frac{2x-2-x\sqrt{x}}{2x\sqrt{x}}>0\)
\(\Leftrightarrow 2x-2-x\sqrt{x}>0\)
\(\Leftrightarrow x\sqrt{x}+2< 2x\)
Điều này vô lý do theo BĐT Cô-si thì:\(x\sqrt{x}+2=\frac{x\sqrt{x}}{2}+\frac{x\sqrt{x}}{2}+2\geq 3\sqrt[3]{\frac{x^3}{2}}>\frac{3x}{\sqrt[3]{2}}> 2x\)
Vậy không tồn tại $x$ thỏa mãn.
1) Ta có: \(P=\left(\dfrac{1}{x-\sqrt{x}}+\dfrac{1}{\sqrt{x}-1}\right):\dfrac{x}{x-2\sqrt{x}+1}\)
\(=\left(\dfrac{1}{\sqrt{x}\left(\sqrt{x}-1\right)}+\dfrac{\sqrt{x}}{\sqrt{x}\left(\sqrt{x}-1\right)}\right)\cdot\dfrac{\left(\sqrt{x}-1\right)^2}{x}\)
\(=\dfrac{x-1}{x\sqrt{x}}\)

\(P=\left(\dfrac{\sqrt{x}-2}{x-4}+\dfrac{\sqrt{x}+2}{x-4}\right).\dfrac{\sqrt{x}\left(\sqrt{x}-2\right)}{2}\)
\(=\left(\dfrac{\sqrt{x}-2+\sqrt{x}+2}{x-4}\right).\dfrac{\sqrt{x}\left(\sqrt{x}-2\right)}{2}\)
\(=\dfrac{2\sqrt{x}.\sqrt{x}.\left(\sqrt{x}-2\right)}{2.\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\dfrac{x}{\sqrt{x}+2}\)
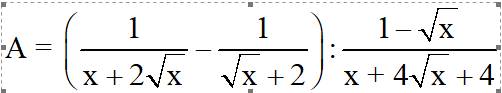
Bạn nên viết đề bằng công thức toán (biểu tượng $\sum$ góc trái khung soạn thảo) để mọi người hiểu đề hơn.