K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
10 tháng 11 2018
câu a đề có sai số mũ ko vậy
b) \(\dfrac{x^4+x^3-x-1}{x^4+x^3+2x^2+x+1}\)
\(=\dfrac{x^3\left(x+1\right)-\left(x+1\right)}{x^4+x^3+x^2+x^2+x+1}\)
\(=\dfrac{\left(x^3-1\right)\left(x+1\right)}{x^2\left(x^2+x+1\right)+\left(x^2+x+1\right)}\)
\(=\dfrac{\left(x+1\right)\left(x-1\right)\left(x^2+x+1\right)}{\left(x^2+x+1\right)\left(x^2+1\right)}=\dfrac{x^2-1}{x^2+1}\)
c) \(\dfrac{x^4+6x^3+9x^2-1}{x^4+6x^3+7x^2-6x+1}\)
\(=\dfrac{\left(x^2+3x\right)^2-1}{x^4+6x^3+9x^2-2x^2-6x+1}\)
\(=\dfrac{\left(x^2+3x-1\right)\left(x^2+3x+1\right)}{\left(x^2+3x\right)^2-2\left(x^2+3x\right)+1}\)
\(=\dfrac{\left(x^2+3x-1\right)\left(x^2+3x+1\right)}{\left(x^2+3x-1\right)^2}=\dfrac{x^2+3x+1}{x^2-3x+1}\)
23 tháng 12 2019
\(\frac{6x^3\left(2y+1\right)}{5y}\cdot\frac{15}{2x^3\left(2y+1\right)}=\frac{9}{y}\)
\(\frac{3}{x^2-1}:\frac{6x}{2x^3\left(2y+1\right)}=\frac{3}{x^2-1}\cdot\frac{2x^3\left(2y+1\right)}{6x}=\frac{x^2\left(2y+1\right)}{x^2-1}\)
hok tốt.
29 tháng 12 2019
\(\frac{6x^3\left(2y+1\right)}{5y}\cdot\frac{15}{2x^3\left(2y+1\right)}\)
\(=\frac{6x^3\left(2y+1\right)}{5y}\cdot\left[\frac{15}{2x^3\left(2y+1\right)}\right]\)
\(=\frac{180x^3y+90x^3}{20x^3y^2+10x^3y}\)
\(=\frac{180y+90}{20y^2+10y}\)
\(=\frac{18y+9}{2y^2+y}\)
\(=\frac{9\left(2y+1\right)}{y\left(2y+1\right)}\)
\(=\frac{9}{y}\)
26 tháng 8 2020
a) ĐKXĐ: \(x\notin\left\{\frac{1}{2};\frac{-1}{2}\right\}\)
Ta có: \(\frac{1+8x}{8x+4}=\frac{2x}{6x-3}-\frac{8x^2}{3-12x^2}\)
\(\Leftrightarrow\frac{8x+1}{4\left(2x+1\right)}=\frac{2x}{3\left(2x-1\right)}+\frac{8x^2}{3\left(4x^2-1\right)}\)
\(\Leftrightarrow\frac{3\left(8x+1\right)\left(2x-1\right)}{12\left(2x+1\right)\left(2x-1\right)}=\frac{2x\cdot4\cdot\left(2x+1\right)}{12\left(2x+1\right)\left(2x-1\right)}+\frac{32x^2}{12\left(2x-1\right)\left(2x+1\right)}\)
Suy ra: \(3\left(8x+1\right)\left(2x-1\right)=8x\left(2x+1\right)+32x^2\)
\(\Leftrightarrow3\left(16x^2-8x+2x-1\right)=16x^2+8x+32x^2\)
\(\Leftrightarrow3\left(16x^2-6x-1\right)=48x^2+8x\)
\(\Leftrightarrow48x^2-18x-3-48x^2-8x=0\)
\(\Leftrightarrow-26x-3=0\)
\(\Leftrightarrow-26x=3\)
hay \(x=-\frac{3}{26}\)
Vậy: \(S=\left\{-\frac{3}{26}\right\}\)
b) Ta có: \(\left(x-2\right)\left(x-3\right)< \left(x-4\right)^2-2\left(x+3\right)\)
\(\Leftrightarrow x^2-5x+6< x^2-8x+16-2x-6\)
\(\Leftrightarrow x^2-5x+6< x^2-10x+10\)
\(\Leftrightarrow x^2-5x+6-x^2+10x-10< 0\)
\(\Leftrightarrow5x-4< 0\)
\(\Leftrightarrow5x< 4\)
hay \(x< \frac{4}{5}\)
Vậy: S={x|\(x< \frac{4}{5}\)}
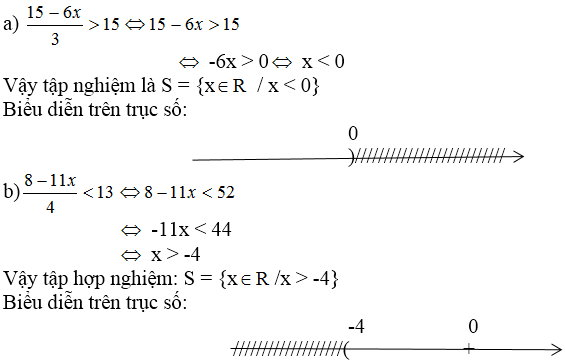
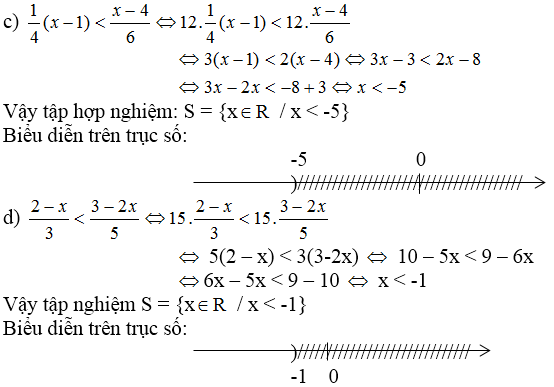
4(x - 1) + 6x \(\le\) 3(x + 1) + 15
<=> 4x - 4 + 6x \(\le\) 3x + 3 + 15
<=> 7x \(\le\) 22
<=> x \(\le\) \(\dfrac{22}{7}\) S = {x|x\(\le\) \(\dfrac{22}{7}\)}
4(x-1) + 6x ≤ 3(x+1) + 15
<=> 4x + 6x - 3x ≤ 3 + 15 + 4
<=> 7x ≤ 22 <=> x ≤ 22/7