
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a )
Để A \(⋮\) B thì \(x^n\ge x^3\) \(\Rightarrow n\ge3\)
Để M \(⋮\) N thì \(y^n\ge y^2\Rightarrow n\ge2\)
a, A= 5\(x^ny^3\)
B= 4\(x^3y\)
=> A\(⋮\)B -> n \(\ge\)3
b, làm tương tự như trên

c)(x2+x)2-2(x2+x)-15
đặt x2+x=a ta có
a2-2a-15
=a2+3a-5a-15
=(a2+3a)-(5a+15)
=a(a+3)-5(a+3)
=(a+3)(a-5)
thay a=x2+x
(x2+x+3)(x2+x-5)

a)\(\dfrac{a^2+b^2-c^2+2ab}{a^2-b^2+c^2+2ac}=\dfrac{\left(a+b\right)^2-c^2}{\left(a+c\right)^2-b^2}=\dfrac{\left(a+b+c\right)\left(a+b-c\right)}{\left(a+b+c\right)\left(a-b+c\right)}=\dfrac{a+b-c}{a-b+c}\)Giá trị của biểu thức trên tại \(a=4;b=-5;c=6\) là:
\(\dfrac{4-5-6}{4-\left(-5\right)+6}=-\dfrac{7}{15}\)
b: \(=\dfrac{8x\left(2x-5y\right)}{8x\left(x-3y\right)}=\dfrac{2x-5y}{x-3y}\)
Đặt x/10=y/3=k
=>x=10k; y=3k
\(A=\dfrac{2\cdot10k-5\cdot3k}{10k-3\cdot3k}=\dfrac{5k}{k}=5\)
c: \(C=\left(\dfrac{x^3-y^3-x^3-y^3}{\left(x+y\right)\left(x-y\right)}\right):\dfrac{x^2-y^2-x^2}{x+y}\)
\(=\dfrac{-2y^3}{\left(x+y\right)\left(x-y\right)}\cdot\dfrac{x+y}{-y^2}=\dfrac{2y}{x-y}\)
\(=\dfrac{20}{9-10}=-20\)

a,
\(\dfrac{18\left(x-y\right)^{10}}{2\left(x-y\right)^5}=9\left(x-y\right)^5\)
b, \(\dfrac{10\left(x-2\right)^{12}}{\left(2-x\right)^{10}}=\dfrac{10\left(x-2\right)^{12}}{\left(x-2\right)^{10}}=10\left(x-2\right)^2\)
c, \(\dfrac{-18\left(x-3\right)^5}{2\left(3-x\right)^3}=\dfrac{-18\left(x-3\right)^5}{-2\left(x-3\right)^3}=9\left(x-3\right)^2\)
d,\(\dfrac{x^2-6x+9}{x-3}=\dfrac{\left(x-3\right)^2}{x-3}=x-3\)
e, \(\dfrac{x^2-x-2}{x+1}=\dfrac{x^2-2x+x-2}{x+1}=\dfrac{\left(x-2\right)\left(x+1\right)}{x+1}=x-2\)

a: \(x^2-10x+26+y^2+2y=0\)
\(\Leftrightarrow x^2-10x+25+y^2+2y+1=0\)
\(\Leftrightarrow\left(x-5\right)^2+\left(y+1\right)^2=0\)
=>x=5 hoặc y=-1
b: \(x^2-6x+13+y^2+4y=0\)
\(\Leftrightarrow x^2-6x+9+y^2+4y+4=0\)
\(\Leftrightarrow\left(x-3\right)^2+\left(y+2\right)^2=0\)
=>x=3 và y=-2
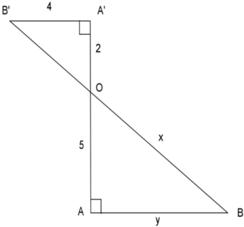

Áp dụng định lý Py-ta-go cho tam giác vuông OA’B’, ta có: O A ’ 2 + A ’ B ’ 2 = O B ' 2
⇔ 2 2 + 4 2 = O B ’ 2 ⇔ O B ' 2 = 20 ⇒ O B ’ = 20
A’B’ ⊥ AA’, AB ⊥ AA’ => A’B’// AB
(Theo định lý từ vuông góc đến song song)
Áp dụng định lý Ta-lét, ta có: O A ' O A = O B ' O B = A ' B ' A B
⇒ 20 x = 2 5 4 y = 2 5 ⇒ x = 5 20 2 = 5 5 y = 4.5 2 = 10
Vậy x = 5 5 , y = 10
Đáp án: D