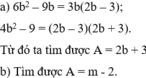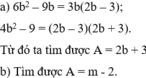
a) ...">
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời. a) Rút gọn: \(M=\left(x+3\right).\left(x^2-3x+9\right)-\left(x^3+54-x\right)\) \(M=\left(x+3\right).\left(x^2-3x+3^2\right)-\left(x^3+54-x\right)\) \(M=x^3+3^3-\left(x^3+54-x\right)\) \(M=x^3+27-x^3-54+x\) \(M=x-27.\) + Thay \(x=27\) vào biểu thức M ta được: \(M=27-27\) \(\Rightarrow M=0.\) Vậy giá trị của biểu thức M tại \(x=27\) là: \(0.\) Chúc bạn học tốt! \(x^2+2xy+y^2-9\\
=\left(x+y\right)^2-3^2\\
=\left(x+y+3\right)\left(x+y-3\right)\) \(x^4-x^3-3x^2+x+2\\
=x^4-x^2-x^3+x-2x^2+2\\
=x^2\left(x^2-1\right)-x\left(x^2-1\right)-2\left(x^2-1\right)\\
=\left(x^2-1\right)\left(x^2-x-2\right)\\
\left(x^4-x^3-3x^2+x+2\right):\left(x^2-1\right)\\
=\dfrac{x^4-x^3-3x^2+x+2}{x^2-1}\\
=\dfrac{\left(x^2-1\right)\left(x^2-x-2\right)}{x^2-1}\\
=x^2-x-2\) \(x^2-2x+5\\
=x^2-2x+1+4\\
=\left(x-1\right)^2+4\\
\left(x-1\right)^2\ge0\\
\Leftrightarrow\left(x-1\right)^2+4\ge4>0\) Vậy biểu thức luôn dương với mọi x Bài 3: a) ta có: \(A=x^2+4x+9\) \(=x^2+4x+4+5=\left(x+2\right)^2+5\) Ta có: \(\left(x+2\right)^2\ge0\forall x\) \(\Rightarrow\left(x+2\right)^2+5\ge5\forall x\) Dấu '=' xảy ra khi \(\left(x+2\right)^2=0\Leftrightarrow x+2=0\Leftrightarrow x=-2\) Vậy: GTNN của đa thức \(A=x^2+4x+9\) là 5 khi x=-2 b) Ta có: \(B=2x^2-20x+53\) \(=2\left(x^2-10x+\frac{53}{2}\right)\) \(=2\left(x^2-10x+25+\frac{3}{2}\right)\) \(=2\left[\left(x-5\right)^2+\frac{3}{2}\right]\) \(=2\left(x-5\right)^2+2\cdot\frac{3}{2}\) \(=2\left(x-5\right)^2+3\) Ta có: \(\left(x-5\right)^2\ge0\forall x\) \(\Rightarrow2\left(x-5\right)^2\ge0\forall x\) \(\Rightarrow2\left(x-5\right)^2+3\ge3\forall x\) Dấu '=' xảy ra khi \(2\left(x-5\right)^2=0\Leftrightarrow\left(x-5\right)^2=0\Leftrightarrow x-5=0\Leftrightarrow x=5\) Vậy: GTNN của đa thức \(B=2x^2-20x+53\) là 3 khi x=5 c) Ta có : \(M=1+6x-x^2\) \(=-x^2+6x+1\) \(=-\left(x^2-6x-1\right)\) \(=-\left(x^2-6x+9-10\right)\) \(=-\left[\left(x-3\right)^2-10\right]\) \(=-\left(x-3\right)^2+10\) Ta có: \(\left(x-3\right)^2\ge0\forall x\) \(\Rightarrow-\left(x-3\right)^2\le0\forall x\) \(\Rightarrow-\left(x-3\right)^2+10\le10\forall x\) Dấu '=' xảy ra khi \(-\left(x-3\right)^2=0\Leftrightarrow\left(x-3\right)^2=0\Leftrightarrow x-3=0\Leftrightarrow x=3\) Vậy: GTLN của đa thức \(M=1+6x-x^2\) là 10 khi x=3 Bài 2: a) \(\left(x+y\right)^2+\left(x^2-y^2\right)\) \(=\left(x+y\right)^2+\left(x-y\right).\left(x+y\right)\) \(=\left(x+y\right).\left(x+y+x-y\right)\) \(=\left(x+y\right).2x\) c) \(x^2-2xy+y^2-z^2+2zt-t^2\) \(=\left(x^2-2xy+y^2\right)-\left(z^2-2zt+t^2\right)\) \(=\left(x-y\right)^2-\left(z-t\right)^2\) \(=\left[x-y-\left(z-t\right)\right].\left(x-y+z-t\right)\) \(=\left(x-y-z+t\right).\left(x-y+z-t\right)\) Chúc bạn học tốt! Bài 1 : a , * A = x2 -2x+9 = x2 -2x +1 +8 = ( x-1) 2 +8 > = 8 với mọi x Dấu ''='' xảy ra <=> x-1 =0 <=> x=1 vậy GTNN của A = 8 <=> x = 1 * B = x2 +6x -3 = x2 +6x +9 - 12 = ( x+ 3 )2 - 12 >= -12 dấu ''='' xảy ra <=> x+3=0 <=> x = -3 Vậy GTNN của B = -12 <=> x = - 3 *C = (x-1) (x-3) +9 = x2 - 4x +3+9 = x2 -4x +4 + 8 = (x-4 )2 +8 >= 8 Dấu ''='' xảy ra <=> x-4 = 0 <=> x=4 vậy GTNN của C = 8 <=> x =4 b, * D = -x2 -4x + 7 = - ( x2 +4x ) +7 = - ( x2 + 4x +4 ) +4 -7 ( vì có dấu trừ đằng trước nên phải bù ra ngoài là + 4 chứ ko phải - 4 = - ( x+2)2 -3 < hoặc = -3 với mọi x Dấu ''='' xảy ra <=> x+2 =0 <=> x = -2 Vậy GTLN của D = -3 <=> x = -2 * E ( đề sao sao ý ) Bài 2 ; a , x2 +2x -15 = x2 +5x -3x -15 = (x2 +5x ) - ( 3x +15) = x( x+5)-3(x+5) = (x-3)(x+5) = 0 <=> \(\left[{}\begin{matrix}x-3=0\\x+5=0\end{matrix}\right.\) <=> x= 3 or x=-5 b, tách tương tự 1/ A= \(\dfrac{2x+6}{\left(x+3\right)\left(x-2\right)}\) = 0 ;(ĐKXĐ : x ≠ -3; x ≠ 2) ⇔ A = \(\dfrac{2\left(x+3\right)}{\left(x+3\right)\left(x-2\right)}\) = 0 ⇔ A = \(\dfrac{2}{x-2}\) = 0 ⇒ x = 2 (loại) ⇒ pt vô nghiệm