
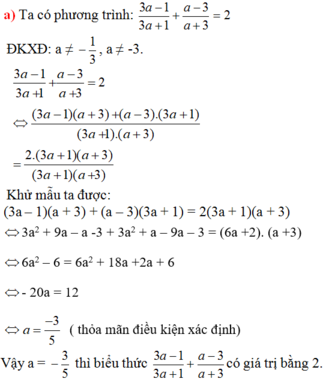
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

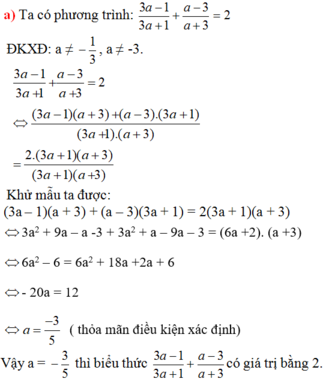

ĐKXĐ của phương trình : \(\orbr{\begin{cases}x\ne-\frac{1}{3}\\x\ne-3\end{cases}}\)
\(\frac{3a-1}{3a+1}+\frac{a-3}{a+3}=2\)
\(\Leftrightarrow\left(3a-1\right)\left(a+3\right)+\left(a-3\right)\left(3a+1\right)=2\left(3a+1\right)\left(a+3\right)\)\(\Leftrightarrow3a^2+8a-3+3a^2-8a-3=2\left(3a^2+10a+3\right)\)
\(\Leftrightarrow6a^2-6-6a^2-20a-6=0\)
\(\Leftrightarrow-20a-12=0\Leftrightarrow a=\frac{-12}{20}=-\frac{3}{5}\)(NHẬN)
vậy tập nghiệm của phương trình là : S = { -3/5 }
Tk mk nka !!! Th@nks !!

a) \(\frac{2a^2-3a-2}{a^2-4}=2\)
\(\Rightarrow2a^2-3a-2=2\left(a^2-4\right)\)
\(\Rightarrow2a^2-3a-2=2a^2-4\)
\(\Rightarrow-3a-2=-4\)
\(\Rightarrow-3a=-2\Rightarrow a=\frac{2}{3}\)
b) \(\frac{3a-1}{3a+1}+\frac{a-3}{a+3}=2\)
\(\Rightarrow\frac{\left(3a-1\right)\left(a+3\right)+\left(3a+1\right)\left(a-3\right)}{\left(3a+1\right)\left(a+3\right)}=2\)
\(\Rightarrow\frac{6a^2-6}{3a^2+10a+3}=2\)
\(\Rightarrow6a^2-6=2\left(3a^2+10a+3\right)\)
\(\Rightarrow6a^2-6=6a^2+20a+6\)
\(\Rightarrow-6=20a+6\Rightarrow20a=-12\)
\(\Rightarrow a=\frac{-3}{5}\)
Tìm giá trị của a sao cho biểu thức sau có giá trị bằng 2
\(\frac{3a-1}{3a+1}+\frac{a-3}{a+3}=\)\(2\)

\(ĐKXĐ:\)\(a\ne-3\)\(;a\ne\frac{-1}{3}\)
\(\frac{3a-1}{3a+1}+\frac{a-3}{a+3}=\)\(2\)
\(\Leftrightarrow\frac{\left(3a-1\right)\left(a+3\right)}{\left(3a+1\right)\left(a+3\right)}+\frac{\left(3a+1\right)\left(a-3\right)}{\left(3a+1\right)\left(a+3\right)}\)\(=\frac{2\left(3a+1\right)\left(a+3\right)}{\left(3a+1\right)\left(a+3\right)}\)
\(\Leftrightarrow\left(3a-1\right)\left(a+3\right)+\left(3a+1\right)\left(a-3\right)-2\left(3a+1\right)\left(a+3\right)\)\(=0\)
\(\Leftrightarrow3a^2+9a-a-3+3a^2-9a+a-3-6a^2-18a-2a-6\)\(=0\)
\(\Leftrightarrow\left(3a^2+3a^2-6a^2\right)+(9a-a-9a+a-18a-2a)-\left(3+3+6\right)\)\(=0\)
\(\Leftrightarrow-20a-12=0\)
\(\Leftrightarrow-20a=12\)
\(\Leftrightarrow a=\frac{-12}{20}=\frac{-3}{5}\)( thỏa mãn )
\(Vậy\) \(a=\frac{-3}{5}\)khi biểu thức có giá trị là 2

Bài 2:
(1 + x)3 + (1 - x)3 - 6x(x + 1) = 6
<=> x3 + 3x2 + 3x + 1 - x3 + 3x2 - 3x + 1 - 6x2 - 6x = 6
<=> -6x + 2 = 6
<=> -6x = 6 - 2
<=> -6x = 4
<=> x = -4/6 = -2/3
Bài 3:
a) (7x - 2x)(2x - 1)(x + 3) = 0
<=> 10x3 + 25x2 - 15x = 0
<=> 5x(2x - 1)(x + 3) = 0
<=> 5x = 0 hoặc 2x - 1 = 0 hoặc x + 3 = 0
<=> x = 0 hoặc x = 1/2 hoặc x = -3
b) (4x - 1)(x - 3) - (x - 3)(5x + 2) = 0
<=> 4x2 - 13x + 3 - 5x2 + 13x + 6 = 0
<=> -x2 + 9 = 0
<=> -x2 = -9
<=> x2 = 9
<=> x = +-3
c) (x + 4)(5x + 9) - x2 + 16 = 0
<=> 5x2 + 9x + 20x + 36 - x2 + 16 = 0
<=> 4x2 + 29x + 52 = 0
<=> 4x2 + 13x + 16x + 52 = 0
<=> 4x(x + 4) + 13(x + 4) = 0
<=> (4x + 13)(x + 4) = 0
<=> 4x + 13 = 0 hoặc x + 4 = 0
<=> x = -13/4 hoặc x = -4

a, A xác định
\(\Leftrightarrow3x^3-19x^2+33x-9\ne0\)
\(\Leftrightarrow3x^3-x^2-18x^2+6x+27x-9\ne0\)
\(\Leftrightarrow x^2\left(3x-1\right)-6x\left(3x-1\right)+9\left(3x-1\right)\ne0\)
\(\Leftrightarrow\left(3x-1\right)\left(x-3\right)^2\ne0\Leftrightarrow\hept{\begin{cases}x\ne\frac{1}{3}\\x\ne3\end{cases}}\)
b, \(\frac{3x^3-14x^2+3x+36}{3x^2-19x^2+33x-9}=\frac{3x^2\left(x-3\right)-5x\left(x-3\right)-12\left(x-3\right)}{\left(3x-1\right)\left(x-3\right)^2}\)
\(=\frac{\left(3x^2-5x-12\right)\left(x-3\right)}{\left(3x-1\right)\left(x-3\right)^2}=\frac{\left(3x+4\right)\left(x-3\right)^2}{\left(3x-1\right)\left(x-3\right)^2}=\frac{3x+4}{3x-1}\)
\(A=0\Leftrightarrow\frac{3x+4}{3x-1}=0\Leftrightarrow3x+4=0\Leftrightarrow x=-\frac{4}{3}\) (thỏa mãn ĐKXĐ)
c, \(A=\frac{3x+4}{3x-1}=1+\frac{5}{3x-1}\in Z\Rightarrow5⋮\left(3x-1\right)\)
\(\Rightarrow3x-1\inƯ\left(5\right)=\left\{-5;-1;1;5\right\}\)
\(\Rightarrow x\in\left\{-\frac{4}{3};0;\frac{2}{3};2\right\}\)
Mà \(x\in Z,x\ne\left\{\frac{1}{3};3\right\}\Rightarrow x\in\left\{0;2\right\}\)
Bài của Hùng rất thông minh
Đang định có cách khác mà dài hơn cách Hùng nên thui
^^ 2k5 kết bạn nhé