Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Cái này là toán lp 9 mà :D
a/ Để...\(\Leftrightarrow\left\{{}\begin{matrix}m-3\ne1\\2m+1=1\end{matrix}\right.\Leftrightarrow m=0\)
b/ Vì (d1) cắt...
Ta có PTHĐGĐ:
(m-3)x+2m+1=3x-2
Thay x= 2 vào có:
(m-3).2+2m+1= 3.2-2
\(\Leftrightarrow2m-6+2m+1=4\)
\(\Leftrightarrow m=\frac{9}{4}\) (tm)
c/ Vì...
Thay y= -3 vào y= x-5
\(\Rightarrow x=2\)
Thay x= 2; y= -3 vào (d1)
(m-3).2+2m+1= -3
\(\Leftrightarrow2m-6+2m+1=-3\)
\(\Leftrightarrow m=\frac{1}{2}\)

a/ Để đường thẳng đi qua gốc tọa độ
\(\Leftrightarrow m+1=0\Rightarrow m=-1\)
b/ Để đường thẳng cắt trục tung tại điểm có tung độ -5
\(\Leftrightarrow m+1=-5\Leftrightarrow m=-6\)
c/ Đường thẳng cắt trục hoành tại điểm 3 nghĩa là gì bạn??? Chắc là có hoành độ bằng 3?
\(\Leftrightarrow3\left(m+2\right)+m+1=0\)
\(\Leftrightarrow4m+6=0\Rightarrow m=-\frac{3}{2}\)

Phương trình hoành độ giao điểm:
\(x^2+2x-m+1=x+1\)
\(\Leftrightarrow x^2+x-m=0\left(1\right)\)
\(\left(d\right),\left(P\right)\) cắt nhau tại hai điểm phân biệt khi phương trình \(\left(1\right)\) có hai nghiệm phân biệt
\(\Leftrightarrow\Delta=4m+1>0\Leftrightarrow m>-\dfrac{1}{4}\)
Phương trình \(\left(1\right)\) có hai nghiệm phân biệt \(x=\dfrac{-1\pm\sqrt{4m+1}}{2}\)
\(x=\dfrac{-1+\sqrt{4m+1}}{2}\Rightarrow y=\dfrac{1+\sqrt{4m+1}}{2}\Rightarrow A\left(\dfrac{-1+\sqrt{4m+1}}{2};\dfrac{1+\sqrt{4m+1}}{2}\right)\)
\(x=\dfrac{-1-\sqrt{4m+1}}{2}\Rightarrow y=\dfrac{1-\sqrt{4m+1}}{2}\Rightarrow B\left(\dfrac{-1-\sqrt{4m+1}}{2};\dfrac{1-\sqrt{4m+1}}{2}\right)\)
\(AB=8\Leftrightarrow\sqrt{8m+2}=8\Leftrightarrow m=\dfrac{31}{4}\left(tm\right)\)
2.
a, \(AB=2\sqrt{5},BC=5\sqrt{10},CA=\sqrt{170}\)
\(AM^2=\dfrac{AB^2+AC^2}{2}-\dfrac{BC^2}{4}=\dfrac{65}{2}\Rightarrow AM=\dfrac{\sqrt{130}}{2}\)
b, \(\left\{{}\begin{matrix}x_D-4-2\left(x_D-2\right)+4\left(x_D+3\right)=0\\y_D-3-2\left(y_D-7\right)+4\left(y_D+8\right)=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_D=-4\\y_D=-\dfrac{14}{3}\end{matrix}\right.\)
\(\Rightarrow D\left(-4;-\dfrac{14}{3}\right)\)
c, \(\left\{{}\begin{matrix}\overrightarrow{AA'}=\left(x_{A'}-4;y_{A'}-3\right)\\\overrightarrow{BC}=\left(-5;-15\right)\\\overrightarrow{BA'}=\left(x_{A'}-2;y_{A'}-7\right)\end{matrix}\right.\)
\(AA'\perp BC\Leftrightarrow\left\{{}\begin{matrix}\overrightarrow{AA'}.\overrightarrow{BC}=0\left(1\right)\\\overrightarrow{BA'}=k\overrightarrow{BC}\left(2\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow-5\left(x_{A'}-4\right)-15\left(y_{A'}-3\right)=0\Leftrightarrow x_{A'}+3y_{A'}=13\)
\(\left(2\right)\Leftrightarrow\left\{{}\begin{matrix}x_{A'}-2=-5k\\y_{A'}-7=-15k\end{matrix}\right.\Leftrightarrow3x_{A'}-y_{A'}=-1\)
\(\left\{{}\begin{matrix}x_{A'}+3y_{A'}=13\\3x_{A'}-y_{A'}=-1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_{A'}=1\\y_{A'}=4\end{matrix}\right.\Rightarrow A'\left(1;4\right)\)


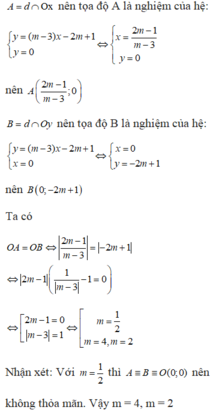
Đáp án B