Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) 45 . 80
= 5 . 9 . 5 . 16 = 5 2 . 3 2 . 4 2 = 5 . 3 . 4 = 60

sin α - sin α c o s 2 α = sin α (1 – c o s 2 α )
= sin α [( sin 2 α + c o s 2 α ) – c o s 2 α ]
= sin α .( sin 2 α + c o s 2 α – c o s 2 α )
= sin α . sin 2 α = sin 3 α

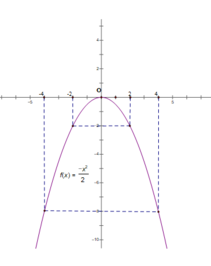
a) Vẽ đồ thị hàm số (P): y = (-1)/2 x 2
Bảng giá trị :
| x | -4 | -2 | 0 | 2 | 4 |
| y = (-1)/2 x2 | -8 | -2 | 0 | -2 | -8 |
Đồ thị hàm số y = (-1)/2 x 2 là một đường Parabol nằm phía dưới trục hoành, nhận trục tung làm trục đối xứng, nhận gôc tọa độ O(0;0) làm đỉnh và là điểm cao nhất.

\(\sin^4\alpha+\cos^4\alpha+2\sin^2\alpha.\cos^2\alpha=\left(\sin^2\alpha+\cos^2\alpha\right)^2=1\)
\(\tan^2\alpha\left(2.\cos^2\alpha+\sin^2\alpha-1\right)=\tan^2\alpha\left(\cos^2\alpha+\left(\sin^2\alpha+\cos^2\alpha\right)-1\right)\)\(=\tan^2\alpha.\cos^2\alpha=\left(\frac{1}{\cos^2\alpha}-1\right)\cos^2\alpha=1-\cos^2\alpha=\sin^2\alpha\)

1.
a) \(\left(1-cos_x\right)\left(1+cos_x\right)-sin^2_x=1-cos^2_x-sin^2_x=1-\left(cos^2_x+sin^2_x\right)=1-1=0\)
b) \(tan^2_x\left(2.cos^2_x+sin^2_x-1\right)+cos^2_x=tan^2_x\left(cos^2_x+sin^2_x+cos^2_x-1\right)+cos^2_x=tan^2_x\left(1-1+cos^2_x\right)+cos^2_x=tan^2_x.cos^2_x+cos^2_x=\left(tan_x.cos_x\right)^2+cos^2_x=sin^2_x+cos^2_x=1\)2. Ta có \(9>5\Leftrightarrow\sqrt{9}>\sqrt{5}\Leftrightarrow3>\sqrt{5}\Leftrightarrow3-\sqrt{5}>0\)
Vậy \(3-\sqrt{5}>0\)
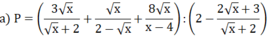
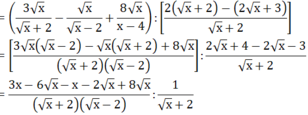
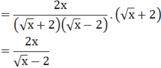
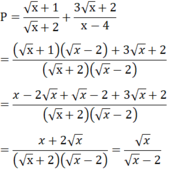
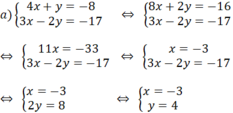
a) 1 - sin 2 α = cos 2 α