Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(a,2x\left(x-3\right)+5\left(x-3\right)=0\)
\(\Leftrightarrow\left(2x+5\right)\left(x-3\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}2x+5=0\\x-3=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}2x=-5\\x=3\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=-\frac{5}{2}\\x=3\end{cases}}\)
Vậy .........
\(b,\left(x^2-4\right)+\left(x-2\right)\left(3-2x=0\right)\)
\(\Leftrightarrow x^2-4-2x^2+7x-6=0\)
\(\Leftrightarrow-x^2+7x-10=0\)
\(\Leftrightarrow-\left(x-5\right)\left(x-2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=5\\x=2\end{cases}}\)
Vậy ..................
\(c,x^3-3x^2+3x-1=0\)
\(\Leftrightarrow\left(x-1\right)^3=0\)
\(\Leftrightarrow x=1\)
\(d,x\left(2x-7\right)-4x+14=0\)
\(\Leftrightarrow2x^2-7x-4x+14=0\)
\(\Leftrightarrow2x^2-11x+14=0\)
\(\Leftrightarrow\left(2x-7\right)\left(x-2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=\frac{7}{2}\\x=2\end{cases}}\)
Vậy ............
\(e,\left(2x-5\right)^2-\left(x+2\right)^2=0\)
\(\Leftrightarrow4x^2-20x+25-x^2-4x-4=0\)
\(\Leftrightarrow3x^2-24x+21=0\)
\(\Leftrightarrow3\left(x-7\right)\left(x-1\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x-7=0\\x-1=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=7\\x=1\end{cases}}\)
Vậy .....................
\(f,x^2-x-\left(3x-3\right)=0\)
\(\Leftrightarrow x^2-x-3x+3=0\)
\(\Leftrightarrow x^2-4x+3=0\)
\(\Leftrightarrow\left(x-3\right)\left(x-1\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x-3=0\\x-1=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=3\\x=1\end{cases}}\)
Vậy ..............

(x2 – 4) + (x – 2)(3 – 2x) = 0
⇔ (x – 2)(x + 2) + (x – 2)(3 – 2x) = 0
⇔ (x – 2)[(x + 2) + (3 – 2x)] = 0
⇔ (x – 2)(5 – x) = 0
⇔ x – 2 = 0 hoặc 5 – x = 0
+ x – 2 = 0 ⇔ x = 2
+ 5 – x = 0 ⇔ x = 5.
Vậy tập nghiệm của phương trình là S = {2; 5}.

(2x – 5)2 – (x + 2)2 = 0
⇔ [(2x – 5) + (x + 2)].[(2x – 5) – (x + 2)]= 0
⇔ (2x – 5 + x + 2).(2x – 5 – x - 2) = 0
⇔ (3x – 3)(x – 7) = 0
⇔ 3x – 3 = 0 hoặc x – 7 = 0
+ 3x – 3 = 0 ⇔3x = 3 ⇔ x = 1.
+ x – 7 = 0 ⇔ x = 7.
Vậy phương trình có tập nghiệm S = {1; 7}.

bài 1:
a) ĐKXĐ: x khác 0; x khác -1
\(\frac{x-1}{x}+\frac{1-2x}{x^2+x}=\frac{1}{x+1}\)
<=> \(\frac{x-1}{x}+\frac{1-2x}{x\left(x+1\right)}=\frac{1}{x+1}\)
<=> (x - 1)(x + 1) + 1 - 2x = x
<=> x^2 - 2x = x
<=> x^2 - 2x - x = 0
<=> x^2 - 3x = 0
<=> x(x - 3) = 0
<=> x = 0 hoặc x - 3 = 0
<=> x = 0 hoặc x = 0 + 3
<=> x = 0 (ktm) hoặc x = 3 (tm)
=> x = 3
b) ĐKXĐ: x khác +-3; x khác -7/2
\(\frac{13}{\left(x-3\right)\left(2x+7\right)}+\frac{1}{2x+7}=\frac{6}{x^2-9}\)
<=> \(\frac{13}{\left(x-3\right)\left(2x+7\right)}+\frac{1}{2x+7}=\frac{6}{\left(x-3\right)\left(x+3\right)}\)
<=> 13(x + 3) + (x - 3)(x + 3) = 6(2x + 7)
<=> 13x + 30 + x^2 = 12x + 42
<=> 13x + 30 + x^2 - 12x - 42 = 0
<=> x - 12 + x^2 = 0
<=> (x - 3)(x + 4) = 0
<=> x - 3 = 0 hoặc x + 4 = 0
<=> x = 0 + 3 hoặc x = 0 - 4
<=> x = 3 (ktm) hoặc x = -4 (tm)
=> x = -4
c) ĐKXĐ: x khác +-1
\(\frac{x}{x-1}-\frac{2x}{\left(x-1\right)\left(x+1\right)}=0\)
<=> x(x + 1) - 2x = 0
<=> x^2 + x - 2x = 0
<=> x^2 - x = 0
<=> x(x - 1) = 0
<=> x = 0 hoặc x - 1 = 0
<=> x = 0 hoặc x = 0 + 1
<=> x = 0 (tm) hoặc x = 1 (ktm)
=> x = 0
d) \(\frac{x^2+2x}{x^2+1}-2x=0\)
<=> \(\frac{x\left(x+2\right)}{x^2+1}-2x=0\)
<=> x(x + 2) - 2x(x^2 + 1) = 0
<=> x^2 - 2x^3 = 0
<=> x^2(1 - 2x) = 0
<=> x^2 = 0 hoặc 1 - 2x = 0
<=> x = 0 hoặc -2x = 0 - 1
<=> x = 0 hoặc -2x = -1
<=> x = 0 hoặc x = 1/2
bài 2:
(x - 1)(x^2 + 3x - 2) - (x^3 - 1) = 0
<=> x^3 + 3x^2 - 2x - x^2 - 3x + 2 - x^2 + 1 = 0
<=> 2x^2 - 2x - 3x + 3 = 0
<=> 2x(x - 1) - 3(x - 1) = 0
<=> (2x - 3)(x - 1) = 0
<=> 2x - 3 = 0 hoặc x - 1 = 0
<=> 2x = 0 + 3 hoặc x = 0 + 1
<=> 2x = 3 hoặc x = 1
<=> x = 3/2 hoặc x = 1
bài 3:
(x^3 + x^2) + (x^2 + x) = 0
<=> x^3 + x^2 + x^2 + x = 0
<=> x^3 + 2x^2 + x = 0
<=> x(x^2 + 2x + 1) = 0
<=> x(x + 1)^2 = 0
<=> x = 0 hoặc x + 1 = 0
<=> x = 0 hoặc x = 0 - 1
<=> x = 0 hoặc x = -1

x(2x – 7) – 4x + 14 = 0
⇔ x.(2x - 7) – (4x – 14) = 0
⇔ x(2x – 7) – 2(2x – 7) = 0
⇔(x – 2)(2x – 7) = 0
⇔ x – 2 = 0 hoặc 2x – 7 = 0
+ x – 2 = 0 ⇔ x = 2.
+ 2x – 7 = 0 ⇔ 2x = 7 ⇔ x = 7/2
Vậy tập nghiệm của phương trình là 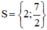

x2 – x – (3x – 3) = 0
⇔ x(x – 1) – 3(x – 1) = 0
⇔ (x – 3)(x – 1) = 0
⇔ x – 3 = 0 hoặc x – 1 = 0
+ x – 3 = 0 ⇔ x = 3
+ x – 1 = 0 ⇔ x = 1.
Vậy phương trình có tập nghiệm S = {1; 3}.

a) 2x(x - 3) + 5(x - 3) = 0 ⇔ (x - 3)(2x + 5) = 0 ⇔ x - 3 = 0 hoặc 2x + 5 = 0
1) x - 3 = 0 ⇔ x = 3
2) 2x + 5 = 0 ⇔ 2x = -5 ⇔ x = -2,5
Vậy tập nghiệm của phương trình là S = {3;-2,5}
b) (x2 - 4) + (x - 2)(3 - 2x) = 0 ⇔ (x - 2)(x + 2) + (x - 2)(3 - 2x) = 0
⇔ (x - 2)(x + 2 + 3 - 2x) = 0 ⇔ (x - 2)(-x + 5) = 0 ⇔ x - 2 = 0 hoặc -x + 5 = 0
1) x - 2 = 0 ⇔ x = 2
2) -x + 5 = 0 ⇔ x = 5
Vậy tập nghiệm của phương trình là S = {2;5}
a) 2x(x - 3) + 5(x - 3) = 0 ⇔ (x - 3)(2x + 5) = 0 ⇔ x - 3 = 0 hoặc 2x + 5 = 0 1) x - 3 = 0 ⇔ x = 3 2) 2x + 5 = 0 ⇔ 2x = -5 ⇔ x = -2,5 Vậy tập nghiệm của phương trình là S = {3;-2,5} b) (x2 - 4) + (x - 2)(3 - 2x) = 0 ⇔ (x - 2)(x + 2) + (x - 2)(3 - 2x) = 0 ⇔ (x - 2)(x + 2 + 3 - 2x) = 0 ⇔ (x - 2)(-x + 5) = 0 ⇔ x - 2 = 0 hoặc -x + 5 = 0 1) x - 2 = 0 ⇔ x = 2 2) -x + 5 = 0 ⇔ x = 5 Vậy tập nghiệm của phương trình là S = {2;5}

a) 2x(x - 3) + 5(x - 3) = 0 ⇔ (x - 3)(2x + 5) = 0 ⇔ x - 3 = 0 hoặc 2x + 5 = 0
1) x - 3 = 0 ⇔ x = 3
2) 2x + 5 = 0 ⇔ 2x = -5 ⇔ x = -2,5
Vậy tập nghiệm của phương trình là S = {3;-2,5}
b) (x2 - 4) + (x - 2)(3 - 2x) = 0 ⇔ (x - 2)(x + 2) + (x - 2)(3 - 2x) = 0
⇔ (x - 2)(x + 2 + 3 - 2x) = 0 ⇔ (x - 2)(-x + 5) = 0 ⇔ x - 2 = 0 hoặc -x + 5 = 0
1) x - 2 = 0 ⇔ x = 2
2) -x + 5 = 0 ⇔ x = 5
Vậy tập nghiệm của phương trình là S = {2;5}
Câu a)
2x(x - 3) + 5(x - 3) = 0
⇔ (x - 3)(2x + 5) = 0
⇔ x - 3 = 0 hoặc 2x + 5 = 0
1) x - 3 = 0 ⇔ x = 3
2) 2x + 5 = 0 ⇔ 2x = -5 ⇔ x = -2,5
Vậy tập nghiệm của phương trình là S = {3;-2,5}
Câu b)
(x2 - 4) + (x - 2)(3 - 2x) = 0
⇔ (x - 2)(x + 2) + (x - 2)(3 - 2x) = 0
⇔ (x - 2)(x + 2 + 3 - 2x) = 0
⇔ (x - 2)(-x + 5) = 0
⇔ x - 2 = 0 hoặc -x + 5 = 0
1) x - 2 = 0 ⇔ x = 2
2) -x + 5 = 0 ⇔ x = 5
Vậy tập nghiệm của phương trình là S = {2;5}
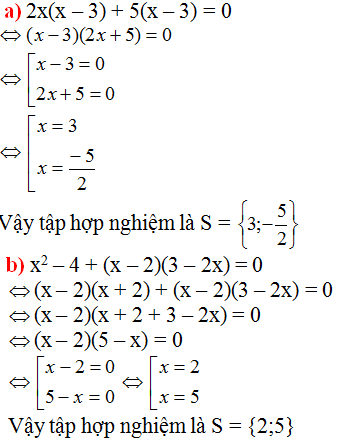
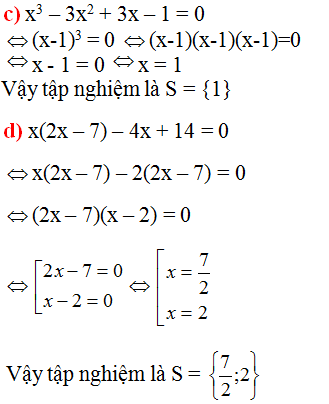
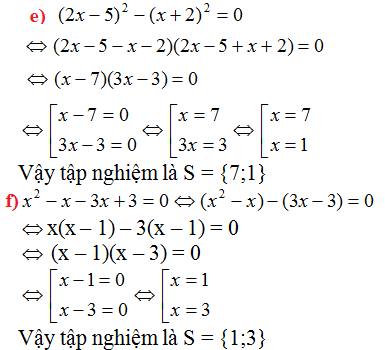
2x(x – 3) + 5(x – 3) = 0
⇔ (2x + 5)(x – 3) = 0
⇔ 2x + 5 = 0 hoặc x – 3 = 0
+ 2x + 5 = 0 ⇔2x = -5 ⇔ x = -5/2
+ x – 3 = 0 ⇔x = 3.
Vậy phương trình có tập nghiệm