Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Câu này cách làm tương tự câu mũ 4 ở trên nhé, đặt ẩn phụ và biến đổi như vậy

Mk chỉ làm đc câu a) thôi còn câu b mk cũng đang hỏi.
Đặt \(4-x=a\); \(x-2=b\) \(\Rightarrow\) \(a+b=2\)
\(\Leftrightarrow\)\(\left(a^3+b^3\right)\left(a^2+b^2\right)-a^2b^2\left(a+b\right)=32\)
\(\Leftrightarrow\)\(\left[\left(a+b\right)^3-3ab\left(a+b\right)\right]\left[\left(a+b\right)^2-2ab\right]-a^2b^2\left(a+b\right)=32\)
thay \(a+b=2\) ta có:
\(\left(8-6ab\right)\left(4-2ab\right)-2\left(ab\right)^2=32\)
\(\Leftrightarrow\) \(32-40ab+10\left(ab\right)^2=32\)
\(\Leftrightarrow\)\(10ab\left(-4+ab\right)+32-32=0\)
\(\Leftrightarrow\)\(ab\left(ab-4\right)=0\)
\(\Leftrightarrow\)\(\left[{}\begin{matrix}ab=0\\ab-4=0\end{matrix}\right.\)
Với \(ab=0\) thì \(\left(4-x\right)\left(x-2\right)=0\)
\(\Leftrightarrow\)\(\left[{}\begin{matrix}4-x=0\\x-2=0\end{matrix}\right.\) \(\Rightarrow\) \(\left[{}\begin{matrix}x=4\\x=2\end{matrix}\right.\)
Với \(ab-4=0\) thì \(\left(4-x\right)\left(x-2\right)-4=0\)
\(\Leftrightarrow\)\(6x-8-x^2-4=0\)
\(\Leftrightarrow\)\(6x-12-x^2=0\)
\(\Leftrightarrow\)\(-\left(x^2-6x+12\right)=0\)
\(\Leftrightarrow\)\(-\left(x^2-6x+9+3\right)=0\)
\(\Leftrightarrow\)\(-\left(x-3\right)^2-3=0\) ( vô lí )
Vậy pt có tập nghiệm \(S=\left\{2;4\right\}\)

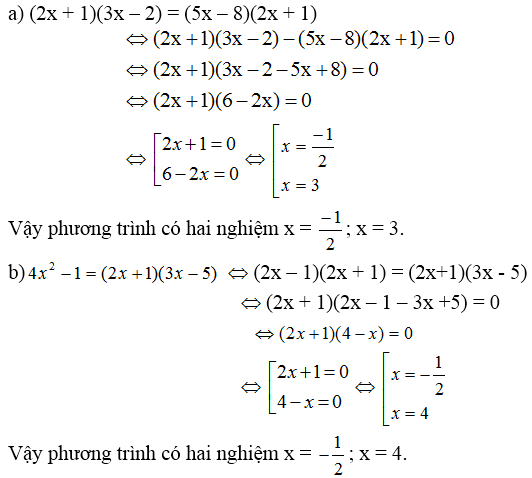
a)(2x+1)(3x-2)=(5x-8)(2x+1)
⇔(2x+1)(3x-2)-(5x-8)(2x+1)=0
⇔(2x+1)(3x-2-5x+8)=0
⇔(2x+1)(-2x+6)=0
⇔2x+1=0 hoặc -2x+6=0
1.2x+1=0⇔2x=-1⇔x=-1/2
2.-2x+6=0⇔-2x=-6⇔x=3
phương trình có 2 nghiệm x=-1/2 và x=3

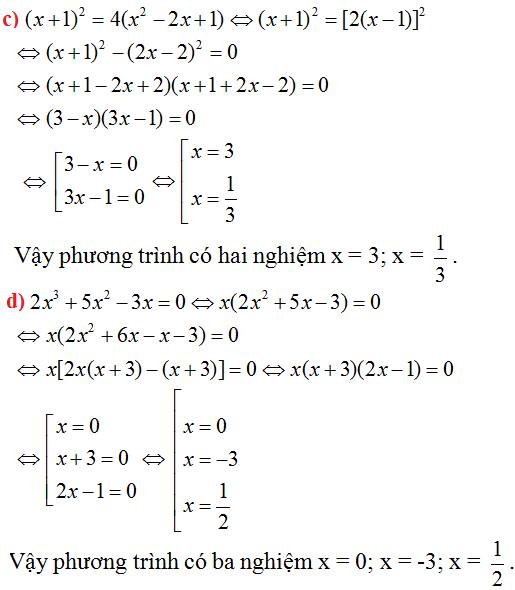
Đặt \(4-x=a\) và \(x-2=b\) \(\Rightarrow a+b=2\)
Ta có:
\(a^5+b^5=32\\\Leftrightarrow \left(a^3+b^3\right)\left(a^2+b^2\right)-a^2b^2\left(a+b\right)=32\\ \Leftrightarrow\left[\left(a+b\right)^3-3ab\left(a+b\right)\right]\left[\left(a+b\right)^2-2ab\right]-a^2b^2\left(a+b\right)=32\\\Leftrightarrow \left(8-6ab\right)\left(4-2ab\right)-2\left(ab\right)^2=32\\\Leftrightarrow 12\left(ab\right)^2-40ab+32=32\\\Leftrightarrow 4ab\left(3ab-10\right)=0\\\Leftrightarrow \left[{}\begin{matrix}4ab=0\\3ab-10=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}ab=0\\ab=\frac{10}{3}\end{matrix}\right.\)
Cho \(ab=\frac{10}{3}\) thì \(a,b\) là nghiệm của phương trình:
\(x^2-2x+\frac{10}{3}=0\) \(\Rightarrow\) Vô nghiệm
Cho \(ab=0\) thì \(a,b\) là nghiệm của phương trình:
\(x^2-2x=0\\\Leftrightarrow x\left(x-2\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x-2=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=0\\x=2\end{matrix}\right.\)
\(\Rightarrow a=2vab=0\) hoặc \(a=0vab=2\)
Thử:
Với \(a=2vab=0\) ta có:
\(\Rightarrow4-x=2\\ \Leftrightarrow x=2\\ \Rightarrow x-2=0\\ \Leftrightarrow x=2\)
Vậy nghiệm của phương trình là \(2\)
Với \(a=0vab=2\) ta có:
\(\Rightarrow4-x=0\\ \Leftrightarrow x=4\\ \Rightarrow x-2=2\\\Leftrightarrow x=4\)
Vậy nghiệm của phương trình là 4
Do đó tập nghiệm của phương trình là: \(S=\left\{2;4\right\}\)