K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PT
1
TT
2
DD
Đoàn Đức Hà
Giáo viên
7 tháng 7 2021
ĐK: \(cosx\ne0\Leftrightarrow x\ne\frac{\pi}{2}+k\pi,k\inℤ\).
\(1+tanx=2\left(sinx+cosx\right)\)
\(\Leftrightarrow cosx+sinx=2cosx\left(sinx+cosx\right)\)
\(\Leftrightarrow\orbr{\begin{cases}sinx+cosx=0\\cosx=\frac{1}{2}\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}cosx=cos\left(-x-\frac{\pi}{2}\right)\\cosx=cos\frac{\pi}{3}\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=\pm\left(-x-\frac{\pi}{2}\right)+k2\pi\\x=\pm\frac{\pi}{3}+k2\pi\end{cases}},\left(k\inℤ\right)\)
\(\Leftrightarrow\orbr{\begin{cases}x=\frac{-\pi}{4}+k\pi\\x=\pm\frac{\pi}{3}+k2\pi\end{cases}},\left(k\inℤ\right)\)(thỏa mãn)
A
6 tháng 7 2021
\(1+\tan x=2\left(\sin x+\cos x\right)\)
Bạn áp dụng đẳng thức lượng giác nhé :
\(\frac{\sin x+\cos x}{\cos x}=2\sin x+2\cos x\)
Biệt thức :
\(D=b^2-4ac\)
\(\Leftrightarrow\left(-1\right)^2-4\left(1.1\right)=-3\)
Phương trình không có nghiệm thực :
\(D< 0\)
Nghiệm tuần hoàn :
\(2\pi k-\frac{\pi}{4}\)
\(2\pi k+\frac{3\pi}{4}\)
\(2\pi k+\frac{\pi}{3}\)
\(2\pi k-\frac{\pi}{3}\)
Ps : không hiểu chỗ nào thì bạn hỏi mình nhé, nhớ k :33
# Aeri #
9D
1
3 tháng 10 2021
\(sinx=\dfrac{2tan\dfrac{x}{2}}{tan^2\dfrac{x}{2}+1}\)
\(cosx=\dfrac{1-tan^2\dfrac{x}{2}}{1+tan^2\dfrac{x}{2}}\)
Đặt \(t=tan\dfrac{x}{2}\)
Khi đó pt: \(\Rightarrow a\cdot\dfrac{2t}{t^2+1}+b\cdot\dfrac{1-t^2}{1+t^2}=c\)
\(\Rightarrow2t\cdot a+\left(1-t^2\right)\cdot b=\left(1+t^2\right)\cdot c\)
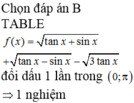
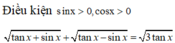
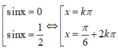
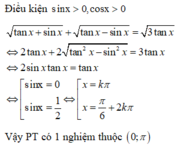
\(tanx=tan20^0\)
\(\Rightarrow x=20^0+k180^0\) (\(k\in Z\))