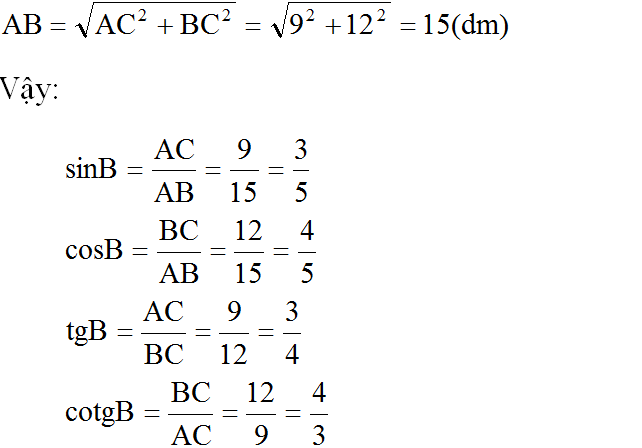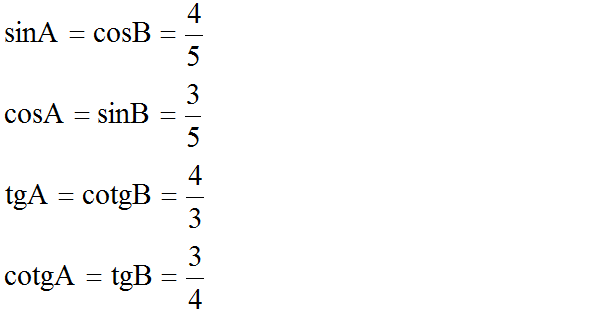Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

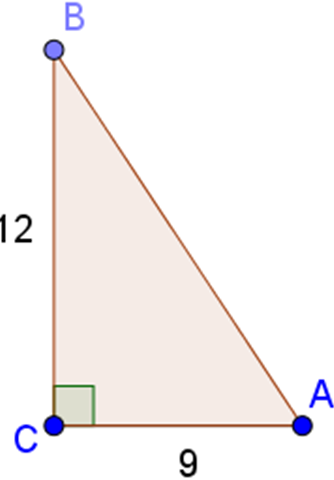
Áp dụng định lý Pitago:
\(AB=\sqrt{AC^2+BC^2}=1,5\left(cm\right)\)
\(sinB=\dfrac{AC}{AB}=0,6\) \(\Rightarrow cosA=sinB=0,6\)
\(cosB=\dfrac{BC}{AB}=0,8\) \(\Rightarrow sinA=cosB=0,8\)
\(tanB=\dfrac{AC}{BC}=\dfrac{3}{4}\) \(\Rightarrow cotA=tanB=\dfrac{3}{4}\)
\(cotB=\dfrac{BC}{AB}=\dfrac{4}{3}\) \(\Rightarrow tanA=cotB=\dfrac{4}{3}\)

Áp dụng PTG: \(BC=\sqrt{AB^2+AC^2}=5\left(cm\right)\)
\(\sin\widehat{B}=\cos\widehat{C}=\dfrac{AC}{BC}=\dfrac{4}{5}\\ \cos\widehat{B}=\sin\widehat{C}=\dfrac{AB}{BC}=\dfrac{3}{5}\\ \tan\widehat{B}=\cot\widehat{C}=\dfrac{AC}{AB}=\dfrac{4}{3}\\ \cot\widehat{B}=\tan\widehat{C}=\dfrac{AB}{AC}=\dfrac{3}{4}\)

tam giác ABC ( A=90*)
=> \(AB=\sqrt{BC^2-AC^2}=\sqrt{1,2^2-0,9^2}\approx0,79\)( theo đlý pytago)
=> \(\sin B=\frac{AC}{BC}=\frac{0,9}{1,2}\approx1,14\)\(\Rightarrow\sin B=\cos A\approx1,14\)
\(\Rightarrow\cos B=\frac{AB}{BC}=\frac{0,79}{1,2}\approx0,66\Rightarrow\cos B=\sin A\approx0,66\)
\(\Rightarrow\tan B=\frac{AC}{AB}=\frac{0,9}{0,79}\approx1,139\Rightarrow\tan B=\cot A\approx1,139\)
\(\Rightarrow\cot B=\frac{AB}{AC}=\frac{0,79}{0,9}\approx0,87\Rightarrow\cot B=\tan A\approx0,87\)

Sử dụng các tỉ số lượng giác, tính được:
sinB = 3 5 ; cosB = 4 5 ; tanB = 3 4 ; cotB = 4 3
=> sinA = 4 5 ; cosA = 3 5 ; tanA = 4 3 ; cotA = 3 4

a: Xét ΔBAC vuông tại A có
\(BC^2=AB^2+AC^2\)
hay BC=5(cm)
b: Xét ΔABC vuông tại A có AH là đường cao ứng với cạnh huyền BC
nên \(\left\{{}\begin{matrix}AH\cdot BC=AB\cdot AC\\AB^2=BH\cdot BC\\AC^2=CH\cdot BC\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}AH=2,4\left(cm\right)\\BH=1,8\left(cm\right)\\CH=3,2\left(cm\right)\end{matrix}\right.\)

Áp dụng định lí pytago vào Δvuông ABC có:
AB²=AC²+BC²=0,9²+1,2²=2,25
⇒AB=1,5(cm)
Có góc A và góc B phụ nhau, ta có:
sin B = cosA= AC/AB = 3/5
cos B = sin A = BC/AB = 4/5
tan B = cot A = AC/BC = 3/4
cot B = tan A = BC/AC = 4/3
Ta có: AC = 0,9m = 9dm; BC = 1,2m = 12dm
Theo định lí Pitago, ta có:
Vì ∠A và ∠B là hai góc phụ nhau nên suy ra:
Bạn tham khảo nha

\(AC=\sqrt{BC^2-AB^2}=4\left(cm\right)\left(pytago\right)\\ \sin\widehat{B}=\cos\widehat{C}=\dfrac{AC}{BC}=\dfrac{4}{5}\\ \cos\widehat{B}=\sin\widehat{C}=\dfrac{AB}{AC}=\dfrac{3}{5}\\ \tan\widehat{B}=\cot\widehat{C}=\dfrac{AC}{AB}=\dfrac{4}{3}\\ \cot\widehat{B}=\tan\widehat{C}=\dfrac{AB}{AC}=\dfrac{3}{4}\)