Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

TL:
sinA+sinB+sinC=1-cosA+cosB+cosC => Tam giác ABC Vuông tại A
Vế trái = sinA + sinB + sinC
= 2sin(A + B)/2.cos(A - B)/2 + 2sinC/2.cosC/2
= 2cosC/2.cos(A - B)/2 + 2sinC/2.cosC/2
= 2cosC/2[cos(A - B)/2 + sinC/2]
=2.cosC/2.[cos(A - B)/2 + cos(A + B)/2]
= 4.cosC/2.cosB/2.cosA/2
Vế phải = 1 - cosA + cosB + cosC
= 2sin²A/2 + 2cos(B + C)/2.cos(B - C)/2
= 2.sinA/2[sinA/2 + cos(B - C)/2] (vì cos(B + C)/2 = sinA/2)
= 2.sinA/2[cos(B + C)/2 + cos(B - C)/2
= 4.sinA/2.cosB/2.cosC/2
Vậy sinA + sinB + sinC = 1 - cosA + cosB + cosC
<=> cosA/2.cosB/2.cosC/2 = sinA/2.cosB/2.cosC/2
<=> cosB/2.cosC/2(sinA/2 - cosA/2) = 0
mà cosB/2 ≠ 0 và cosC/2 ≠ 0
=> sinA/2 = cosA/2
<=> A/2 = 45o
<=> A = 90o
tam giác ABC vuông tại A

\(A=cos^2a+cos^2b+2cosa.cosb+sin^2a+sin^2b+2sina.sinb\)
\(=cos^2a+sin^2a+cos^2b+sin^2b+2\left(cosa.cosb+sina.sinb\right)\)
\(=2+2cos\left(a-b\right)=2+2cos\frac{\pi}{3}=3\)
\(\left(cosa+sina\right)^2=\frac{36}{25}\Leftrightarrow1+2sina.cosa=\frac{36}{25}\)
\(\Rightarrow sin2a=\frac{36}{25}-1=\frac{11}{25}\)
\(cos2a=cos^2a-sin^2a=\left(cosa-sina\right)\left(cosa+sina\right)>0\)
\(\Rightarrow cos2a=\sqrt{1-sin^22a}=\frac{6\sqrt{14}}{25}\)

\(2sinB.sinC=1+cosA\Leftrightarrow cos\left(B-C\right)-cos\left(B+C\right)=1+cosA\)
\(\Leftrightarrow cos\left(B-C\right)+cosA=1+cosA\)
\(\Leftrightarrow cos\left(B-C\right)=1\)
\(\Rightarrow B-C=0\Rightarrow B=C\)
\(sinA=\frac{cosA+cosB}{sinB+sinC}=\frac{cosA+cosB}{2sinB}\) (do \(B=C\))
\(\Leftrightarrow2sinA.sinB=cosA+cosB\)
\(\Leftrightarrow cos\left(A-B\right)-cos\left(A+B\right)=cosA+cosB\)
\(\Leftrightarrow cos\left(A-B\right)+cosC=cosA+cosB\)
\(\Leftrightarrow cos\left(A-B\right)+cosB=cosA+cosB\)
\(\Leftrightarrow cos\left(A-B\right)=cosB\)
\(\Rightarrow A-B=B\Rightarrow A=2B=B+C\)
Mà \(A+B+C=180^0\Rightarrow2A=180^0\Rightarrow A=90^0\)
\(\Rightarrow\Delta ABC\) vuông cân tại A

Chọn C.
Theo giả thiết ta có:
P = ( sina + sinb) 2 + ( cosa + cosb) 2
= sin2a + 2.sina.sinb + sin2b + cos2a + 2cosa. cosb + cos2b
= 2 + 2( sina.sinb + cos a. cosb)
= 2 + 2.cos( a - b) ( sử dụng công thức cộng)
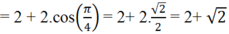

1.
\(sinA+sinB-sinC=2sin\dfrac{A+B}{2}.cos\dfrac{A-B}{2}-sin\left(A+B\right)\)
\(=2sin\dfrac{A+B}{2}.cos\dfrac{A-B}{2}-2sin\dfrac{A+B}{2}.cos\dfrac{A+B}{2}\)
\(=2sin\dfrac{A+B}{2}.\left(cos\dfrac{A-B}{2}-cos\dfrac{A+B}{2}\right)\)
\(=2sin\dfrac{A+B}{2}.2sin\dfrac{A}{2}.sin\dfrac{B}{2}\)
\(=4sin\dfrac{A}{2}.sin\dfrac{B}{2}.cos\dfrac{C}{2}\)
Sao t lại đc như này v, ai check hộ phát

Chọn B.
Ta có: góc A tù nên cos A < 0 ; sinA > 0 ; tan A < 0 ; cot A < 0
Do góc A tù nên góc B và C là các góc nhọn có các giá trị lượng giác đều dương
Do đó: M > 0 ; N > 0 ; P > 0 và Q < 0.
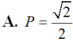
\(sinA+sinB=cosA+cosB\)
\(\Leftrightarrow2sin\frac{A+B}{2}.cos\frac{A-B}{2}=2cos\frac{A+B}{2}cos\frac{A-B}{2}\)
\(\Rightarrow\left[{}\begin{matrix}cos\frac{A-B}{2}=0\\sin\frac{A+B}{2}=cos\frac{A+B}{2}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}A-B=180^0\left(l\right)\\\frac{A+B}{2}=90^0-\frac{A+B}{2}\end{matrix}\right.\)
\(\Rightarrow A+B=90^0\Rightarrow C=90^0\)