

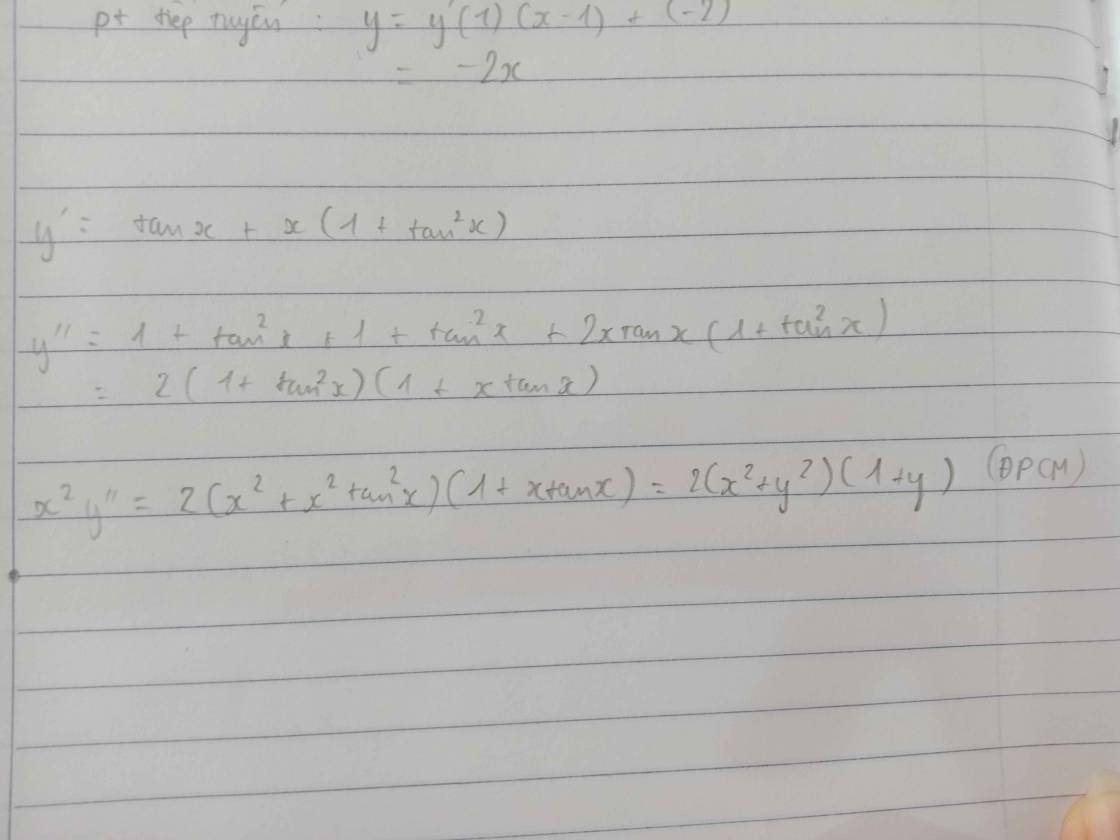
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


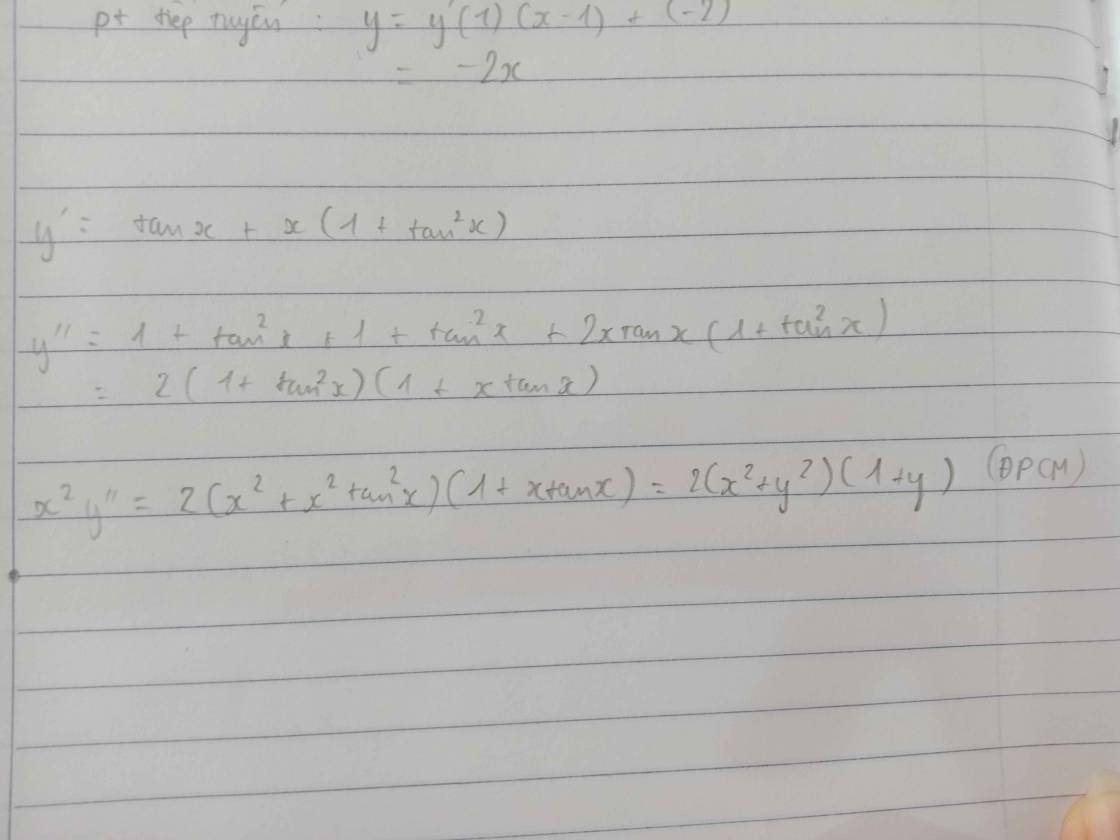

\(y'=\tan x+\frac{x}{\cos^2x}\)
\(y''=\frac{1}{\cos^2x}+\frac{\cos^2-x.2\cos x.\left(-\sin x\right)}{\cos^4x}=\frac{2\cos^2x+2x.\sin x.\cos x}{\cos^4x}\)
\(VT=\frac{2x^2\left(\cos^2x+x\sin x.\cos x\right)}{\cos^4x}\)
\(VP=2\left(x^2+x^2\tan^2x\right)\left(1+x\tan x\right)\)
\(=\frac{2x^2\left(1+x\tan x\right)}{\cos^2x}=\frac{2x^2\left(\cos^2x+x\sin x.\cos x\right)}{\cos^4x}=VT\)

a) Cách 1: y' = (9 -2x)'(2x3- 9x2 +1) +(9 -2x)(2x3- 9x2 +1)' = -2(2x3- 9x2 +1) +(9 -2x)(6x2 -18x) = -16x3 +108x2 -162x -2.
Cách 2: y = -4x4 +36x3 -81x2 -2x +9, do đó
y' = -16x3 +108x2 -162x -2.
b) y' = .(7x -3) +
(7x -3)'=
(7x -3) +7
.
c) y' = (x -2)'√(x2 +1) + (x -2)(√x2 +1)' = √(x2 +1) + (x -2) = √(x2 +1) + (x -2)
= √(x2 +1) +
=
.
d) y' = 2tanx.(tanx)' - (x2)' =
.
e) y' = sin
=
sin
.

a: \(y'=\left[tan\left(e^x+1\right)\right]'=\dfrac{\left(e^x+1\right)'}{cos^2\left(e^x+1\right)}=\dfrac{e^x}{cos^2\left(e^x+1\right)}\)
b: \(y'=\left(\sqrt{sin3x}\right)'\)
\(=\dfrac{\left(sin3x\right)'}{2\sqrt{sin3x}}=\dfrac{3\cdot cos3x}{2\sqrt{sin3x}}\)
c: \(y=cot\left(1-2^x\right)\)
=>\(y'=\left[cot\left(1-2^x\right)\right]'\)
\(=\dfrac{-2}{sin^2\left(1-2^x\right)}\cdot\left(-2^x\cdot ln2\right)\)
\(=\dfrac{2^{x+1}\cdot ln2}{sin^2\left(1-2^x\right)}\)

\(a,y=\left(u\left(x\right)\right)^2=\left(x^2+1\right)^2=x^4+2x^2+1\\ b,y'\left(x\right)=4x^3+4x,u'\left(x\right)=2x,y'\left(u\right)=2u\\ \Rightarrow y'\left(u\right)\cdot u'\left(x\right)=2u\cdot2x=4x\left(x^2+1\right)=4x^3+4x\)
Vậy \(y'\left(x\right)=y'\left(u\right)\cdot u'\left(x\right)\)

\(y=\dfrac{x+3}{x+2}\)
=>\(y'=\dfrac{\left(x+3\right)'\left(x+2\right)-\left(x+3\right)\left(x+2\right)'}{\left(x+2\right)^2}=\dfrac{x+2-x-3}{\left(x+2\right)^2}=\dfrac{-1}{\left(x+2\right)^2}\)
=>C

a.
\(y=\left\{{}\begin{matrix}x-2\left(x\ge2\right)\\2-x\left(x\le2\right)\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}y'\left(2^+\right)=1\\y'\left(2^-\right)=-1\end{matrix}\right.\)
\(\Rightarrow y'\left(2^+\right)\ne y'\left(2^-\right)\Rightarrow\) không tồn tại đạo hàm tại \(x=2\)
b.
\(y=\left|x-2\right|^2=x^2-4x+4\Rightarrow y'=2x-4\)
\(\Rightarrow y'\left(2\right)=0\)
c.
\(y=\left\{{}\begin{matrix}4-x^2\left(\text{với }-2< x< 2\right)\\x^2-4\left(\text{với }x\ge2;x\le-2\right)\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}y'\left(2^+\right)=2x=4\\y'\left(2^-\right)=-2x=-4\end{matrix}\right.\)
\(\Rightarrow y'\left(2^+\right)\ne y'\left(2^-\right)\Rightarrow\) ko tồn tại đạo hàm tại \(x=2\)
d. Tương tự a và c

Đặt \(h\left( x \right) = f\left( x \right) + g\left( x \right) = \frac{1}{{x - 1}} + \sqrt {4 - x} \). Ta có:
\(\begin{array}{l}h\left( 2 \right) = \frac{1}{{2 - 1}} + \sqrt {4 - 2} = 1 + \sqrt 2 \\\mathop {\lim }\limits_{x \to 2} h\left( x \right) = \mathop {\lim }\limits_{x \to x} \left( {\frac{1}{{x - 1}} + \sqrt {4 - x} } \right) = \frac{1}{{2 - 1}} + \sqrt {4 - 2} = 1 + \sqrt 2 \end{array}\)
Vì \(\mathop {\lim }\limits_{x \to 2} h\left( x \right) = h\left( 2 \right)\) nên hàm số \(y = f\left( x \right) + g\left( x \right)\) liên tục tại \(x = 2\).

Bài 1:
Cho $y=0$ thì: $f(x^3)=xf(x^2)$
Tương tự khi cho $x=0$
$\Rightarrow f(x^3-y^3)=xf(x^2)-yf(y^2)=f(x^3)-f(y^3)$
$\Rightarrow f(x-y)=f(x)-f(y)$ với mọi $x,y\in\mathbb{R}$
Cho $x=0$ thì $f(-y)=0-f(y)=-f(y)$
Cho $y\to -y$ thì: $f(x+y)=f(x)-f(-y)=f(x)--f(y)=f(x)+f(y)$ với mọi $x,y\in\mathbb{R}$
Đến đây ta có:
$f[(x+1)^3+(x-1)^3]=f(2x^3+6x)=f(2x^3)+f(6x)$
$=2f(x^3)+6f(x)=2xf(x^2)+6f(x)$
$f[(x+1)^3+(x-1)^3]=f[(x+1)^3-(1-x)^3]$
$=(x+1)f((x+1)^2)-(1-x)f((1-x)^2)$
$=(x+1)f(x^2+2x+1)+(x-1)f(x^2-2x+1)$
$=(x+1)[f(x^2)+2f(x)+f(1)]+(x-1)[f(x^2)-2f(x)+f(1)]$
$=2xf(x^2)+4f(x)+2xf(1)$
Do đó:
$2xf(x^2)+6f(x)=2xf(x^2)+4f(x)+2xf(1)$
$2f(x)=2xf(1)$
$f(x)=xf(1)=ax$ với $a=f(1)$

\(VT\ge3\sqrt[3]{\dfrac{x^3y^3z^3\left(x+y\right)\left(y+z\right)\left(z+x\right)}{\left(x+y\right)\left(y+z\right)\left(z+x\right)}}=3xyz\) (dpcm)