Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Bài 9 : Tìm x, biết :
a, (x - 2)(x - 3) + (x - 2) - 1 = 0
\(\Leftrightarrow\left(x-2\right)\left(x-3+1\right)-1=0\)
\(\Leftrightarrow\left(x-2\right)^2-1=0\)
\(\Leftrightarrow\left(x-2+1\right)\left(x-2-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x-3=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=3\end{matrix}\right.\)
Vậy x ={1; 3}
b, (x + 2)2 - 2x(2x + 3) = (x + 1)2
\(\Leftrightarrow\left(x+2\right)^2-\left(x+1\right)^2-2x\left(2x+3\right)=0\)
\(\Leftrightarrow\left(x+2+x+1\right)\left(x+2-x-1\right)-2x\left(2x+3\right)=0\)
\(\Leftrightarrow2x+3-2x\left(2x+3\right)=0\)
\(\Leftrightarrow\left(2x+3\right)\left(1-2x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+3=0\\1-2x=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=-\frac{3}{2}\\x=\frac{1}{2}\end{matrix}\right.\)
Vậy \(x=\left\{-\frac{3}{2};\frac{1}{2}\right\}\)
c, 6x3 + x2 = 2x
\(\Leftrightarrow6x^3+x^2-2x=0\)
\(\Leftrightarrow x\left(6x^2+x-2\right)=0\)
\(\Leftrightarrow x\left(6x^2+4x-3x-2\right)=0\)
\(\Leftrightarrow x\left[2x\left(3x+2\right)-\left(3x+2\right)\right]=0\)
\(\Leftrightarrow x\left(3x+2\right)\left(2x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\3x+2=0\\2x-1=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-\frac{2}{3}\\x=\frac{1}{2}\end{matrix}\right.\)
Vậy \(x=\left\{0;-\frac{2}{3};\frac{1}{2}\right\}\)

Ít thôi -..-
a) ( 3x + 2 )( 2x + 9 ) - ( x + 3 )( 6x + 1 ) = ( x + 1 )2 - ( x + 2 )( x - 2 )
<=> 6x2 + 31x + 18 - ( 6x2 + 19x + 3 ) = x2 + 2x + 1 - ( x2 - 4 )
<=> 6x2 + 31x + 18 - 6x2 - 19x - 3 = x2 + 2x + 1 - x2 + 4
<=> 12x + 15 = 2x + 5
<=> 12x - 2x = 5 - 15
<=> 10x = -10
<=> x = -1
b) ( 2x + 3 )( x - 4 ) + ( x - 5 )( x - 2 ) = ( 3x - 5 )( x - 4 )
<=> 2x2 - 5x - 12 + x2 - 7x + 10 = 3x2 - 17x + 20
<=> 3x2 - 12x - 2 = 3x2 - 17x + 20
<=> 3x2 - 12x - 3x2 + 17x = 20 + 2
<=> 5x = 22
<=> x = 22/5
c) ( x + 2 )3 - ( x - 2 )3 - 12x( x - 1 ) = -8
<=> x3 + 6x2 + 12x + 8 - ( x3 - 6x2 + 12x - 8 ) - 12x2 + 12x = -8
<=> x3 + 6x2 + 12x + 8 - x3 + 6x2 - 12x + 8 - 12x2 + 12x = -8
<=> 12x + 16 = -8
<=> 12x = -24
<=> x = -2
d) ( 3x - 1 )2 - 5( x + 1 ) + 6x - 3.2x + 1 - ( x - 1 )2 = 16
<=> 9x2 - 6x + 1 - 5x - 5 + 6x - 6x + 1 - ( x2 - 2x + 1 ) = 16
<=> 9x2 - 11x - 3 - x2 + 2x - 1 = 16
<=> 8x2 - 9x - 4 = 16
<=> 8x2 - 9x - 4 - 16 = 0
<=> 8x2 - 9x - 20 = 0
( Đến đây bạn có hai sự lựa chọn : 1 là vô nghiệm
2 là nghiệm vô tỉ =) )
a) (3x + 2)(2x + 9) - (x + 3)(6x + 1) = (x + 1)2 - (x + 2)(x - 2)
=> 3x(2x + 9) + 2(2x + 9) - x(6x + 1) - 3(6x + 1) = x2 + 2x + 1 - x(x - 2) - 2(x - 2)
=> 6x2 + 27x + 4x + 18 - 6x2 - x - 18x - 3 = x2 + 2x + 1 - x2 + 2x - 2x + 4
=> (6x2 - 6x2) + (27x + 4x - x - 18x) + (18 - 3) = (x2 - x2) + (2x + 2x - 2x) + (1 + 4)
=> 12x + 15 = 2x + 5
=> 12x + 15 - 2x - 5 = 0
=> 10x + 10 = 0
=> 10x = -10 => x = -1
b) (2x + 3)(x - 4) + (x - 5)(x - 2) = (3x - 5)(x - 4)
=> 2x(x - 4) + 3(x - 4) + x(x - 2) - 5(x - 2) = 3x(x - 4) - 5(x - 4)
=> 2x2 - 8x + 3x - 12 + x2 - 2x - 5x + 10 = 3x2 - 12x - 5x + 20
=> (2x2 + x2) + (-8x + 3x - 2x - 5x) + (-12 + 10) = 3x2 - 17x + 20
=> 3x2 - 12x - 2 = 3x2 - 17x + 20
=> 3x2 - 12x - 2 - 3x2 + 17x - 20 = 0
=> (3x2 - 3x2) + (-12x + 17x) + (-2 - 20) = 0
=> 5x - 22 = 0
=> 5x = 22 => x = 22/5
c) (x + 2)3 - (x - 2)3 - 12x(x - 1) = -8
=> x3 + 6x2 + 12x + 8 - (x3 - 6x2 + 12x - 8) - 12x2 + 12x = -8
=> x3 + 6x2 + 12x + 8 -x3 + 6x2 - 12x + 8 - 12x2 + 12x = -8
=> (x3 - x3) + (6x2 + 6x2 - 12x2) + (12x - 12x + 12x) + (8 + 8) = -8
=> 12x + 16 = -8
=> 12x = -24
=> x = -2
Còn bài cuối làm nốt

1) \(2x-\left|6x-7\right|=-x+8\)
\(\Rightarrow\orbr{\begin{cases}2x-\left(6x-7\right)=-x+8\\2x-\left(-6x+7\right)=-x+8\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}-3x=1\\9x=15\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=-\frac{1}{3}\\x=\frac{5}{3}\end{cases}}\)
Thử lại đều không thỏa mãn.
Vậy phương trình vô nghiệm.
2) \(\frac{\left|x+2\right|}{2}-\frac{\left|x-1\right|}{3}=\frac{1}{4}+\frac{x+3}{6}\)(2)
Với \(x\ge1\): (2) tương đương với:
\(\frac{x+2}{2}-\frac{x-1}{3}=\frac{1}{4}+\frac{x+3}{6}\)
\(\Leftrightarrow0x=-\frac{7}{12}\)(phương trình vô nghiệm)
Với \(-2\le x< 1\): (2) tương đương với:
\(\frac{x+2}{2}-\frac{1-x}{3}=\frac{1}{4}+\frac{x+3}{6}\)
\(\Leftrightarrow\frac{2}{3}x=\frac{1}{12}\Leftrightarrow x=\frac{1}{8}\)(thỏa mãn)
Với \(x< -2\): (2) tương đương với:
\(\frac{-x-2}{2}-\frac{1-x}{3}=\frac{1}{4}+\frac{x+3}{6}\)
\(\Leftrightarrow\frac{-1}{3}x=\frac{25}{12}\Leftrightarrow x=-\frac{25}{4}\)(thỏa mãn)
3) \(\left|x^2-2x\right|=x\)
\(\Rightarrow\orbr{\begin{cases}x^2-2x=x\\x^2-2x=-x\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x^2-3x=0\\x^2-x=0\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=0,x=3\\x=0,x=1\end{cases}}\)
Thử lại đều thỏa mãn.
4) \(\left|x^2-4x+5\right|=x^2-1\)
\(\Leftrightarrow x^2-4x+5=x^2-1\)(vì \(x^2-4x+5=\left(x-2\right)^2+1>0\))
\(\Leftrightarrow-4x=-6\)
\(\Leftrightarrow x=\frac{3}{2}\)

(x+1)(6x2+2x)+(x-1)(6x2+2x)
<=> (6x2+2x)(x+1+x-1)
<=> 2x(3x+1)2x
<=> 4x2(3x+1)
<=> x2=0
3x+1=0
<=> x=0
x= -1/3 (-1 phần 3)

\(2x^2+6x-8=0\)
<=> \(2x^2-2x+8x-8=0\)
<=> \(2x\left(x-1\right)+8\left(x-1\right)=0\)
<=> \(\left(2x+8\right)\left(x-1\right)=0\)
<=> \(\hept{\begin{cases}2x+8=0\\x-1=0\end{cases}}\)
<=> \(\hept{\begin{cases}x=-4\\x=1\end{cases}}\)
\(2x^2-x-1=0\)
<=> \(2x^2-2x+x-1=0\)
<=> \(2x\left(x-1\right)+\left(x-1\right)=0\)
<=> \(\left(2x+1\right)\left(x-1\right)=0\)
<=> \(\hept{\begin{cases}2x+1=0\\x-1=0\end{cases}}\)
<=> \(\hept{\begin{cases}x=-\frac{1}{2}\\x=1\end{cases}}\)
\(4x^2-5x-9=0\)
<=> \(4x^2+4x-9x-9=0\)
<=> \(4x\left(x+1\right)-9\left(x+1\right)=0\)
<=> \(\left(4x-9\right)\left(x+1\right)=0\)
<=> \(\hept{\begin{cases}4x-9=0\\x+1=0\end{cases}}\)
<=> \(\hept{\begin{cases}x=\frac{3}{2}\\x=-1\end{cases}}\)
học tốt
\(2x^2+6x-8=0\)
\(< =>2x^2-2x+8x-8=0\)
\(\Leftrightarrow2x\left(x-1\right)+8\left(x-1\right)=0\)
\(\Leftrightarrow\left(2x+8\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left(2x+8\right)\left(x-1\right)=0\)
\(\Leftrightarrow2x+8=0\)hoặc \(x-1=0\)
\(\Leftrightarrow x=-4\)hoặc \(x=1\)

B1 a, x^3+1=0 <=> x^3 = -1
<=> x=-1
b, x^2=2x<=> x^2-2x = 0
<=> x.(x-2)=0 <=> x=0 hoặc x-2=0
<=> x=0 hoặc x=2
c, 3x^2-6x-24=0
<=> (3x^2+6x)-(12x+24) = 0
<=> (x+2) . (3x-12) = 0
<=> x+2=0 hoặc 3x-12=0
<=> x=-2 hoặc x=4
B2, a, Có 2012^2 = 2012.2012 = (2011+1).2012 = 2011.2012 + 2012
= 2011.2012+2011 + 1 = 2011.(2012+1) +1 = 2011.2013 +1 > 2011.2013
=> 2011.2013 < 2012^2
c, a+b+c = 0 <=> a+b=-c
<=> (a+b)^3 = -c^3
<=> a^3+b^3+3ab.(a+b) = -c^3
<=> a^3+b^3+c^3 + 3ab(a+b)=0
<=> a^3+b^3+c^3 = -3ab.(a+b) = -3ab.(-c) = 3abc => ĐPCM
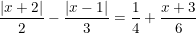


\(x\left(x-2\right)+x-2=0\\ \Leftrightarrow x\left(x-2\right)+\left(x-2\right)=0\\ \Leftrightarrow\left(x-2\right)\left(x+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-2=0\\x+1=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=2\\x=-1\end{matrix}\right.\)
\(x^2-2x+1=9\\ \Leftrightarrow\left(x-1\right)^2=9\\ \Leftrightarrow\left[{}\begin{matrix}x-1=-3\\x-1=3\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-2\\x=4\end{matrix}\right.\)
\(7x^2=2x\\ \Leftrightarrow7x^2-2x=0\\ \Leftrightarrow x\left(7x-2\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\7x-2=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{2}{7}\end{matrix}\right.\)
\(x^2-6x=8\\ \Leftrightarrow x^2-6x-8=0\\ \left(x^2-6x+9\right)-17=0\\ \Leftrightarrow\left(x-3\right)^2-\sqrt{17^2}=0\\ \Leftrightarrow\left(x-3-\sqrt{17}\right)\left(x-3+\sqrt{17}\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-3-\sqrt{17}=0\\x-3+\sqrt{17}=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=3+\sqrt{17}\\x=3-\sqrt{17}\end{matrix}\right.\)
Ơ sao làm mỗi 1 câu vậy bạn ?