Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: \(=2x^3:\dfrac{-3}{2}x+4x:\dfrac{3}{2}x-5:\dfrac{3}{2}\)
=-4/3x^2+8/3-10/3
=-4/3x^2-2/3
d: \(\dfrac{3x^3-5x+2}{x-3}=\dfrac{3x^3-9x^2+9x^2-27x+22x-66+68}{x-3}\)
\(=3x^2+9x+22+\dfrac{68}{x-3}\)

a) Ta có: \(5x^2-3x\left(x+2\right)\)
\(=5x^2-3x^2-6x\)
\(=2x^2-6x\)
b) Ta có: \(3x\left(x-5\right)-5x\left(x+7\right)\)
\(=3x^2-15x-5x^2-35x\)
\(=-2x^2-50x\)
c) Ta có: \(3x^2y\left(2x^2-y\right)-2x^2\left(2x^2y-y^2\right)\)
\(=3x^2y\left(2x^2-y\right)-2x^2y\left(2x^2-y\right)\)
\(=x^2y\left(2x^2-y\right)=2x^4y-x^2y^2\)
d) Ta có: \(3x^2\left(2y-1\right)-\left[2x^2\cdot\left(5y-3\right)-2x\left(x-1\right)\right]\)
\(=6x^2y-3x^2-\left[10x^2y-6x^2-2x^2+2x\right]\)
\(=6x^2y-3x^2-10x^2y+6x^2+2x^2-2x\)
\(=-4x^2y+5x^2-2x\)
e) Ta có: \(4x\left(x^3-4x^2\right)+2x\left(2x^3-x^2+7x\right)\)
\(=4x^4-16x^3+4x^4-2x^3+14x^2\)
\(=8x^4-18x^3+14x^2\)
f) Ta có: \(25x-4\left(3x-1\right)+7x\left(5-2x^2\right)\)
\(=25x-12x+4+35x-14x^3\)
\(=-14x^3+48x+4\)

Lời giải:
a.
$5x-[2x+1-(2x-3)-(4x+1)]=5x-(2x+1-2x+3-4x-1)$
$=5x-(-4x+3)=5x+4x-3=9x-3$
b.
$(-3x^2+2x-1)+(4x^2-2x+3)$
$=-3x^2+2x-1+4x^2-2x+3=x^2+2$

a.
\(3x^2\left(2x^3-x+5\right)=6x^5-3x^3+15x^2\)
\(\Rightarrow6x^5-3x^3+15x^2=6x^5-3x^3+15x^2\)
\(=6x^5-3x^2+15x^2-6x^5-3x^3+15x^2\)
= 0
b.
\(\left(4xy+3y-5x\right)x^2y=4x^3y^2+3x^2y^2-5x^3y\)
\(\Rightarrow4x^3y^2+3x^2y^2-5x^3y=4x^3y^2+3x^2y^2-5x^3y\)
= 0

a/ \(M=\left(-2x^4+x^2+5\right)-\left(5x^2-x^3+4x\right)\)
\(=-2x^4+x^2+5-5x^2+x^3-4x\)
\(=-2x^4+x^3-4x^2-4x+5\)
Vậy...
b/ \(M=-2x^4+x^2+5+5x^2-x^3+4x\)
\(=-2x^4-x^4+6x^2+4x+5\)
Vậy...
c/ \(M=\left(5x^2-x^3+4x\right)-\left(-2x^4+x^2+5\right)\)
\(=5x^2-x^3+4x+2x^4-x^2-5\)
\(=2x^4-x^3+4x^2-5\)
Vậy...
d/ \(M=-\left(5x^2-x^3+4x\right)\)
\(=x^4-5x^2-4x\)
Vậy..
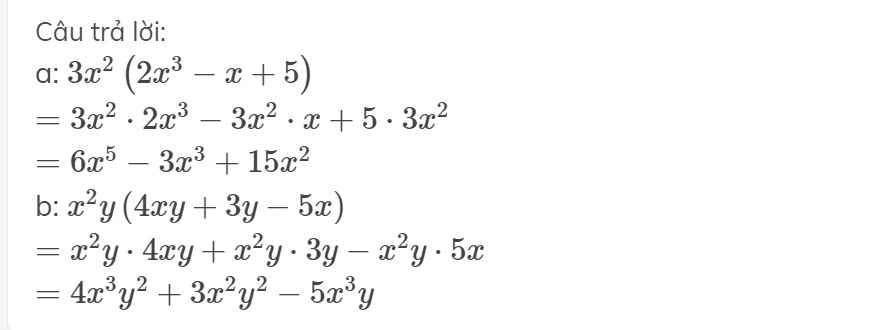
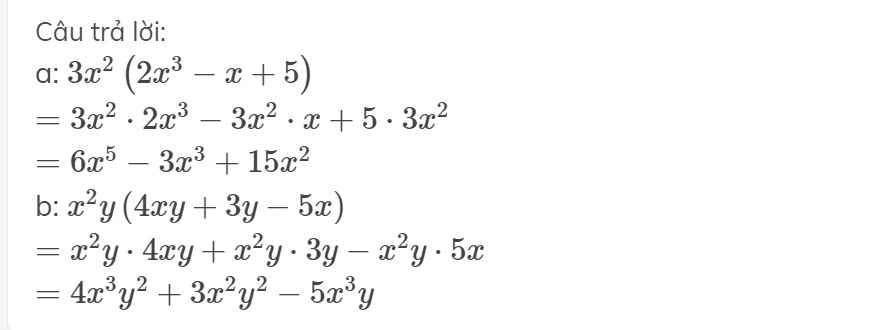
a) 4x²(x² - 5x + 2)
= 4x².x² - 4x².5x + 4x².2
= 4x⁴ - 20x³ + 8x²
b) (2x² - 5x + 3) : (2x - 3)
= (2x² - 3x - 2x + 3) : (2x - 3)
= [(2x² - 3x) - (2x - 3)] : (2x - 3)
= [x(2x - 3) - (2x - 3)] : (2x - 3)
= (2x - 3)(x - 1) : (2x - 3)
= x - 1
a, \(4x^2\left(x^2-5x+2\right)\\ =4x^4-20x^3+8x^2\)
b, \(\left(2x^2-5x+3\right):\left(2x-3\right)\\ =x-1\)