Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\dfrac{2x-3}{2}>\dfrac{1-3x}{6}\)
\(\Leftrightarrow\dfrac{3\left(2x-3\right)}{6}>\dfrac{1-3x}{6}\)
\(\Leftrightarrow6x-9>1-3x\)
\(\Leftrightarrow6x+3x>1+9\)
\(\Leftrightarrow9x>10\)
\(\Leftrightarrow x>\dfrac{10}{9}\)
Vậy BPT có nghiệm \(x>\dfrac{10}{9}\)
> 0 10/9 ( ///////////////////////

a/ \(\dfrac{2-x}{3}< \dfrac{3-2x}{5}\)
\(\Leftrightarrow5\left(2-x\right)< 3\left(3-2x\right)\)
\(\Leftrightarrow10-5x< 9-6x\)
\(\Leftrightarrow x< -1\)
Bpt có tập nghiệm: \(S=\left\{x|x>-1\right\}\)
Biểu diễn tập nghiệm trên trục số:
-1 0
b/ 8x + 3(x+1) > 5x - (2x - 6)
<=> 8x + 3x + 3 > 5x - 2x + 6
<=> 8x + 3x - 5x + 2x > 6 - 3
<=> 8x > 3 <=> x > \(\dfrac{8}{3}\)
Vậy bpt có tập nghiệm là: \(S=\left\{x|x>\dfrac{8}{3}\right\}\)
Biểu diễn........(tự biểu diễn nhé quá dễ r)
c/ \(\left|x-7\right|=-2x+3\) (*)
+) Nếu \(x-7\ge0\Leftrightarrow x\ge7\) thì
|x - 7| = x - 7
(*) => x - 7 = -2x + 3
<=> x + 2x = 3 + 7
<=> 3x = 10 <=> x = \(\dfrac{10}{3}\)(loại)
+) Nếu x - 7 < 0 <=> x < 7
thì |x - 7| = 2x - 3
(*) => x - 7 = 2x - 3
<=> x - 2x = -3 + 7
<=> -x = 4 <=> x = -4 (nhận)
Vậy pt có 1 nghiệm x = -4

\(\dfrac{x+1}{3}>\dfrac{2x-1}{6}-2\)
\(\Leftrightarrow2\left(x+1\right)>2x-1-12\)
\(\Leftrightarrow2x+2>2x-13\) \(\Leftrightarrow2x-2x>-13-2\)
\(\Leftrightarrow0x>-15\) ( luôn đúng)
Vậy bpt trên có vô số nghiệm
\(\Rightarrow\) k cần phải biểu diễn trên trục số

b) \(\dfrac{5\left(4x-1\right)}{15}-\dfrac{2-x}{15}-\dfrac{3\left(10x-3\right)}{15}\le0\)
\(\Leftrightarrow\dfrac{20x-5-2+x-30x+9}{15}\le0\)
\(\Rightarrow-9x+2\le0\)
\(\Leftrightarrow-9x\le-2\)
\(\Rightarrow-9x.\dfrac{-1}{9}\ge-2.\dfrac{-1}{9}\)
\(\Leftrightarrow x\ge\dfrac{2}{9}\)
câu a ,không hiểu đề

a) 2x - 3 > 3(x - 2)
<=> 2x - 3 > 3x - 6
<=> -x > -3
<=> x < 3
b) \(\frac{12x+1}{12}\le\frac{9x+1}{3}-\frac{8x+1}{4}\)
\(\Leftrightarrow\frac{12x+1}{12}\le\frac{4\left(9x+1\right)}{12}-\frac{3\left(8x+1\right)}{12}\)
\(\Leftrightarrow12x+1\le36x+4-24x-3\)
\(\Leftrightarrow0x\le0\)
=> bpt vô số nghiệm
(Bạn tự biểu diễn tập nghiệm nha)
a) Thay x = 3 vào bất phương trình ta được: 2.3 + 3 < 9 <=> 9 < 9 (khẳng định sai)
Vậy x = 3 không là nghiệm của bất phương trình2x + 3 < 9
b) Thay x = 3 vào bất phương trình ta có: -4.3 > 2.3 + 5 => -12 > 11 (khẳng định sai)
Vậy x = 3 không là nghiệm của bất phương trình -4x > 2x + 5
c) Thay x = 3 vào bất phương trình ta có: 5 - 3 > 3.3 -12 => 2 > -3 (khẳng định đúng)
Vậy x = 3 là nghiệm của bất phương trình 5 - x > 3x - 12

\(\dfrac{x-2}{2}+1\le\dfrac{x-1}{3}\)
\(\Leftrightarrow\dfrac{3\left(x-2\right)}{6}+\dfrac{1.6}{6}\le\dfrac{2\left(x-1\right)}{6}\)
`<=> 3x - 6 + 6 <= 2x-2`
`<=> 3x <= 2x-2`
`<=> 3x -2x <= -2`
`<=> x <= -2`
\(\dfrac{x-2}{2}\)+1≤\(\dfrac{x-1}{3}\)
<=>\(\dfrac{3x-6}{6}\)+\(\dfrac{6}{6}\)≤\(\dfrac{2x-1}{6}\)
<=>3x-6+6≤2x-1
<=>x<-1

a)\(\dfrac{x-5}{4}\ge\dfrac{3-2x}{5}\)
\(\Leftrightarrow\dfrac{5x-25}{20}\ge\dfrac{12-8x}{20}\)
\(\Leftrightarrow5x-25\ge12-8x\)
\(\Leftrightarrow5x+8x\ge12+25\)
\(\Leftrightarrow13x\ge37\)
\(\Leftrightarrow x\ge\dfrac{37}{13}\)
b)\(2x\left(6x-1\right)-3< 3x\left(4x+3\right)-5x\)
\(\Leftrightarrow12x^2-2x-3< 12x^2+9x-5x\)
\(\Leftrightarrow12x^2-12x^2-2x-9x+5x< 3\)
\(\Leftrightarrow-6x< 3\)
\(\Leftrightarrow x>-\dfrac{1}{2}\)
c)\(\left|x-4\right|=5-3x\)
\(\Leftrightarrow\left[{}\begin{matrix}5-3x=x-4\\5-3x=4-x\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}5+4=x+3x\\5-4=-x+3x\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4x=9\\2x=1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{9}{4}\\x=\dfrac{1}{2}\end{matrix}\right.\)
p/s: tui làm đúng đề
a.
\(\dfrac{x-5}{4}\ge\dfrac{3-2x}{5}\)
\(\Leftrightarrow5x-25\ge12-8x\)
\(\Leftrightarrow13x\ge37\)
\(\Leftrightarrow x\ge\dfrac{37}{13}\)
0 37 13
b.
\(2x\left(6x-1\right)-3< 3x\left(4x+3\right)-5x\)
\(\Leftrightarrow12x^2-2x-3< 12x^2+9x-5x\)
\(\Leftrightarrow-6x>3\)
\(\Leftrightarrow x< \dfrac{-1}{2}\)
0 -1 2
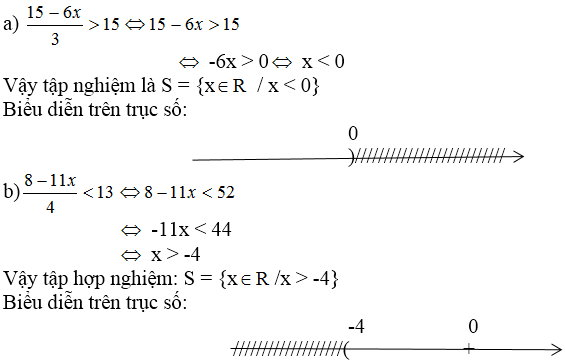
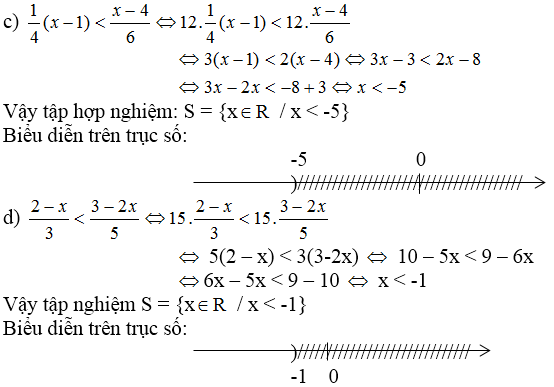
\(\Leftrightarrow3\left(1-2x\right)-2\left(x+1\right)< =6\)
=>3-6x-2x-2<=6
=>-8x+1<=6
=>-8x<=5
hay x>=5/8
dung ko ?