
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


A = 10,11 + 11,12 + 12,13 + . . .+ 98,99 + 99,10
Ta có :
10,11 = 10 + 0,11
11,12 = 11 + 0,12
12,13 = 12 + 0,13
. . . . . . . . . . . . . .
97,98 = 97 + 0,98
98,99 = 98 + 0,99
99,10 = 99 + 0,10
Đặt B = 10 + 11 + 12 + 13 + . .. +98 + 99
và C = 0,11 + 0,12 + 0,13 + . . . .+ 0,98 + 0,99 + 0,10
- - > 100C = 11 + 12 + 13 + . . .+ 98 + 99 + 10
Ta chỉ việc tính B là suy ra C !
B = 10 + 11 + 12 + 13 + . .. +98 + 99
B = (10+99)+(11+98)+(12+97)+. . . +(44+65) + (45 + 64)
Vì từ 10 đến 99 có tất cả 90 số . Ta sẽ có 90/2 = 45 cặp
Mỗi cặp có tổng là 10 + 99 = 11 + 98 = . .= 45 +64 = 109
Vậy ta có B = 45.109 = 4905
Với A = 4905 . Ta thấy 100C = 10 + 11 + 12 +. . + 98 + 99 =B
- - > 100C = 4905 . Hay C = 4905/100 = 49,05
Vậy A = B + C = 4905 + 49,05 = 4954,05

Giống nhau:
- Đều là các số tự nhiên
Khác nhau:
-số nguyên tố tự nhiên chỉ có hai ước là 1 và chính nó
-Hợp số là số tự nhiên có nhiều hơn hai ước
Tích của hai số nguyên tố là hợp số bởi ngoài ước là 1 ra nó còn có ước là hai số nguyên tố đó nữa.

Tuy có vẻ hơi muộn nhưng thôi ![]()
Nếu A là số tự nhiên ⇒ \(\dfrac{1}{10}\left(7^{2004}-3^{92^{94}}\right)\in N\)
\(\Rightarrow7^{2004}-3^{92^{94}}⋮10\)
Thật vậy, ta có :
72004 với lũy thừa là 2004 ⋮ 4
⇒ 72004 = ( .......... 9 )
392^94 với lũy thừa là 9294 mà 92 ⋮ 4 ⇒ 9294 ⋮ 4
⇒ 392^94 = ( .......... 9 )
⇒ 72004 - 392^94 = ( .......... 9 ) - ( ............ 9) = ( ........... 0 ) ⋮ 10
⇒ \(\dfrac{1}{10}\left(7^{2004}-3^{92^{94}}\right)\in N\)
A=1/10.(72004-392^94) là số tự nhiên.

Từ đề bài ta có:
\(T=\dfrac{1+2}{2}.\dfrac{1+3}{3}.\dfrac{1+4}{4}...\dfrac{1+98}{98}.\dfrac{1+99}{99}\)
\(=\dfrac{3}{2}.\dfrac{4}{3}.\dfrac{5}{4}...\dfrac{99}{98}.\dfrac{100}{99}\)
\(=\dfrac{100}{2}\)
\(=50\).
\(T=\left(\dfrac{1}{2}+1\right)\left(\dfrac{1}{3}+1\right)\left(\dfrac{1}{4}+1\right)...\left(\dfrac{1}{98}+1\right)\left(\dfrac{1}{99}+1\right)\)
\(T=\dfrac{3}{2}.\dfrac{4}{3}.\dfrac{5}{4}....\dfrac{99}{98}.\dfrac{100}{99}\)
\(T=\dfrac{3.4.5......99}{3.4.5......99}.\dfrac{100}{2}\)
\(T=50\)

Ta có: \(\left|x-y\right|+\left|x-1\right|\ge0\)
\(\Rightarrow A=\left|x-y\right|+\left|x-1\right|+2017\ge2017\)
Dấu " = " khi \(\left\{{}\begin{matrix}\left|x-y\right|=0\\\left|x-1\right|=0\end{matrix}\right.\Rightarrow x=y=1\)
Vậy \(MIN_A=2017\) khi x = y = 1

2-->8: 4CS
10-->98: 45.2=90CS
100-->998: 450.3=1350CS
1000--> ?: ?.4=?CS
Số cuối cùng của dãy là:
{[(2016-4-90-1350):4]-1}.2+1000=1284
=>CS thứ 2016 của dãy là 4

a) Vì tia Ot nằm giữa 2 tia Ox và Oy
\(\Rightarrow\) \(\widehat{xOt}+\widehat{yOt}=\widehat{xOy}\)
\(\Rightarrow\) 30o + 70o = \(\widehat{xOy}\)
\(\Rightarrow\) \(\widehat{xOy}\) = 100o
Vậy \(\widehat{xOy}\) = 100o
b) Vì tia Ot nằm giữa 2 tia Ox và Oy
\(\Rightarrow\) \(\widehat{xOt}+\widehat{yOt}=\widehat{xOy}\)
\(\Rightarrow\) \(\dfrac{1}{3}\widehat{yOt}+\widehat{yOt}=108^o\)
\(\Rightarrow\) \(\widehat{yOt}\left(\dfrac{1}{3}+1\right)\) = 108o
\(\Rightarrow\) \(\widehat{yOt}\dfrac{1}{4}\) = 108o
\(\Rightarrow\) \(\widehat{yOt}\)= 108o : \(\dfrac{4}{3}\) = 81o
\(\Rightarrow\) \(\widehat{xOt}\)= 81o : 3 = 27o
Vậy \(\widehat{yOt}\) = 81o và \(\widehat{xOt}\) = 27o
c) Vì tia Ot nằm giữa 2 tia Ox và Oy
\(\Rightarrow\) \(\widehat{yOt}+\widehat{xOt}=\widehat{xOy}\)
\(\Rightarrow\) \(\widehat{yOt}+\widehat{xOt}=80^o\)(1)
Theo bài ra, ta có: \(\widehat{yOt}-\widehat{xOt}=20^o\) (2)
Từ (1) và (2) suy ra:
\(\widehat{xOt}\) = (80o - 20o) : 2 = 30o
\(\Rightarrow\) \(\widehat{yOt}\) = 80o - 30o = 50o
Vậy \(\widehat{xOt}\) = 30o và \(\widehat{yOt}\) = 50o
c) Vì tia Ot nằm giưa 2 tia Ox và Oy
\(\Rightarrow\) \(\widehat{xOt}+\widehat{yOt}=\widehat{xOy}\)
\(\Rightarrow\) 50o + \(\widehat{yOt}\) = 100o
\(\Rightarrow\) \(\widehat{yOt}\) = 100o - 50o = 50o
Vậy \(\widehat{yOt}\) = 50o
d) Vì tia Ot nằm giữa 2 tia Ox và Oy
\(\Rightarrow\) \(\widehat{xOt}+\widehat{yOt}=\widehat{xOy}\)
\(\Rightarrow\) ao + bo = \(\widehat{xOy}\)
Vậy \(\widehat{xOy}\)= ao + bo (với 0 \(\le\) a,b \(\le\) 180)


Ta có :
\(A=\dfrac{1}{4}+\dfrac{1}{9}+\dfrac{1}{16}+.........................+\dfrac{1}{81}+\dfrac{1}{10^2}\)
\(A=\dfrac{1}{4}+\dfrac{1}{3^2}+\dfrac{1}{4^2}+.....................+\dfrac{1}{9^2}+\dfrac{1}{10^2}\)
Mà :
\(\dfrac{1}{3^2}>\dfrac{1}{3.4}\)
\(\dfrac{1}{4^2}>\dfrac{1}{4.5}\)
\(\dfrac{1}{5^2}>\dfrac{1}{5.6}\)
.........................................
\(\dfrac{1}{9^2}>\dfrac{1}{9.10}\)
\(\dfrac{1}{10^2}>\dfrac{1}{10.11}\)
\(\Rightarrow A>\dfrac{1}{4}+\dfrac{1}{3.4}+\dfrac{1}{4.5}+........................+\dfrac{1}{9.10}+\dfrac{1}{10^2}\)
\(\Rightarrow A>\dfrac{1}{4}+\dfrac{1}{3}-\dfrac{1}{4}+\dfrac{1}{4}-\dfrac{1}{5}+...................+\dfrac{1}{9}-\dfrac{1}{10}+\dfrac{1}{10}-\dfrac{1}{11}\)
\(\Rightarrow A>\dfrac{1}{4}+\dfrac{1}{3}-\dfrac{1}{11}\)
\(\Rightarrow A>\dfrac{7}{12}-\dfrac{1}{11}\)
\(\Rightarrow A>\dfrac{65}{132}\)\(\rightarrowđpcm\)
Ta có
A = \(\dfrac{1}{4}+\dfrac{1}{9}+\dfrac{1}{16}+...+\dfrac{1}{81}+\dfrac{1}{100}\)
A = \(\dfrac{1}{4}+\dfrac{1}{3.3}+\dfrac{1}{4.4}+...+\dfrac{1}{9.9}+\dfrac{1}{10.10}\)
Vì \(\dfrac{1}{3.3}>\dfrac{1}{3.4}\)
\(\dfrac{1}{4.4}>\dfrac{1}{4.5}\)
.................
\(\dfrac{1}{9.9}>\dfrac{1}{9.10}\)
\(\dfrac{1}{10.10}>\dfrac{1}{10.11}\)
=> A > \(\dfrac{1}{4}+\dfrac{1}{3.4}+\dfrac{1}{4.5}+...+\dfrac{1}{9.10}+\dfrac{1}{10.11}\)
A > \(\dfrac{1}{4}+\dfrac{1}{3}-\dfrac{1}{4}+\dfrac{1}{4}-\dfrac{1}{5}+...+\dfrac{1}{9}-\dfrac{1}{10}+\dfrac{1}{10}-\dfrac{1}{11}\)
A > \(\dfrac{1}{4}+\dfrac{1}{3}-\dfrac{1}{11}\)
A > \(\dfrac{7}{12}-\dfrac{1}{11}\)
A > \(\dfrac{65}{132}\)
Vậy A > \(\dfrac{65}{132}\) < đpcm)
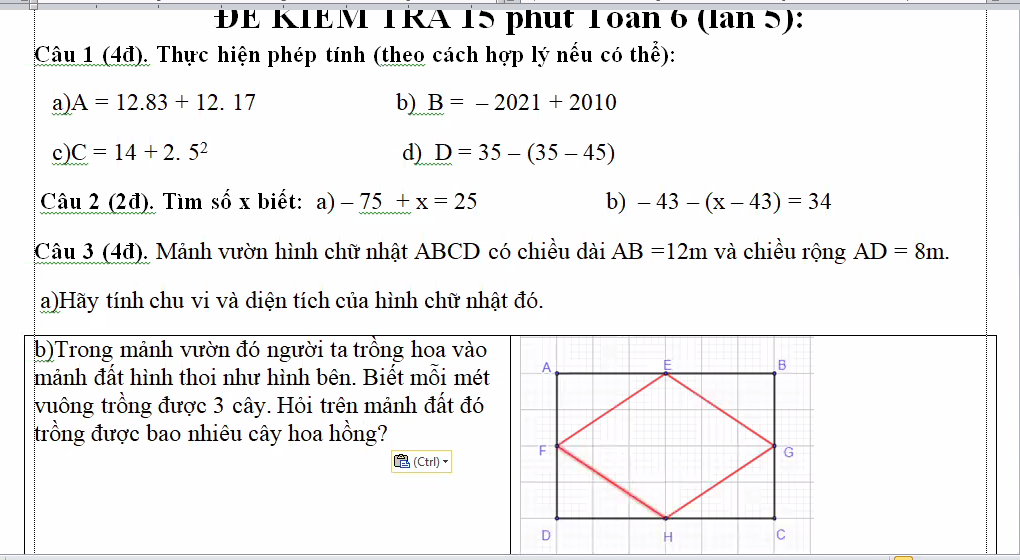

 giải giùm tớ nha
giải giùm tớ nha Giúp mk bài 32 với
Giúp mk bài 32 với làm giùm mình nha.cảm ơn
làm giùm mình nha.cảm ơn
a) \(A=12\left(83+17\right)=12.100=1200\)
b) \(B=-11\)
c) \(C=14+50=64\)
d) \(D=35-35+45=45\)
cảm ơn bn