
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


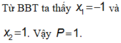

2) Ta có:
\(B=x^4+2x^3y-2x^3+x^2y^2-2x^2y-x\left(x+y\right)+2x+3\)
\(=x^4+x^3y-2x^3+x^3y+x^2y^2-2x^2y-x\left(x+y\right)+2x+3\)
\(=\left(x^4+x^3y-2x^3\right)+\left(x^3y+x^2y^2-2x^2y\right)-\left[x\left(x+y\right)-2x\right]+3\)
Do \(x+y-2=0\Rightarrow x+y=2\)
\(\Rightarrow B=\left(x^4+x^3y-2x^3\right)+\left(x^3y+x^2y^2-2x^2y\right)-\left[2x-2x\right]+3\)
\(=x^3.\left(x+y-2\right)+x^2y\left(x+y-2\right)-0+3\)
\(=0+0+3\)
\(=3\)
Vậy \(B=3\)
1) Ta có:
\(A=x^3+x^2y-2x^2-xy-y^2+3y+x-1\)
\(=\left(x^3+x^2y-2x^2\right)-\left(xy+y^2-2y\right)+y+x-1\)
\(=x^2\left(x+y-2\right)-y\left(x+y-2\right)+\left(x+y-2\right)+1\)
\(=0+0+0+1\)
\(=1\)
Vậy \(A=1\)

a) ĐK: x-1 khác 0 và x+1 khác 0
<=> x khác 1 và x khác -1
b) ĐK: x-2 khác 0
<=> x khác 2

Câu 1 :
Đk: \(x\ge1\)
\(\sqrt{x-1}+\sqrt{2x-1}=5\\ \Leftrightarrow x-1+2\sqrt{\left(x-1\right)\left(2x-1\right)}+2x-1=25\\ \Leftrightarrow2\sqrt{2x^2-3x+1}=27-3x\\ \)
\(\Leftrightarrow\begin{cases}27-3x\ge0\\4\left(2x^2-3x+1\right)=9x^2-162x+729\end{cases}\) \(\Leftrightarrow\begin{cases}x\le9\\x^2-150x+725=0\end{cases}\)
\(\Leftrightarrow\begin{cases}x\le9\\x=145hoặcx=5\end{cases}\)
với x= 5 thoản mãn điều kiện, x=145 loại
Vậy \(S=\left\{5\right\}\)

a) -2x+14=0
<=>-2x= - 14
<=>x = 7
Vậy phương trình có tập nghiệm x={7}
b)(4x-10) (x+5)=0
<=>4x-10=0 <=>4x=10 <=>x=5/2
<=>x+5=0 <=>x=-5
Vậy phương trình có tập nghiệm x={5/2;- 5}
c)\(\frac{1-x}{x+1}\) + 3=\(\frac{2x+3}{x+1}\)
ĐKXD: x+1 #0<=>x#-1(# là khác)
\(\frac{1-x}{x+1}\)+3=\(\frac{2x+3}{x+1}\)
<=>\(\frac{1-x}{x+1}\)+\(\frac{3.\left(x+1\right)}{x+1}\)=\(\frac{2x+3}{x+1}\)
<=>\(\frac{1-x}{x+1}\)+\(\frac{3x+3}{x+1}\)=\(\frac{2x+3}{x+1}\)
=>1-x+3x+3=2x+3
<=>-x+3x-2x=-1-3+3
<=>0x = -1 (vô nghiệm)
Vâyj phương trình vô nghiệm
d) 1,2-(x-0,8)=-2(0,9+x)
<=> 1,2-x+0,8=-1,8-2x
<=>-x+2x=-1,2-0,8-1,8
<=>x=-4
Vậy phương trình có tập nghiệm x={-4}

a) Vì \(\left|x\left(x^2-3\right)\right|\ge0\) nên \(x\ge0\)
Ta có : |x(x2 - 3)| = x
<=> x(x2 - 3) = x <=> x2 - 3 = x : x = 1 <=> x2 = 4
Vì x \(\ge\) 0 nên x = 2

Câu 1.
a). 2A = 8 + 2 3 + 2 4 + . . . + 2 21.
=> 2A – A = 2 21 +8 – ( 4 + 2 2 ) + (2 3 – 2 3) +. . . + (2 20 – 2 20). = 2 21.
b). (x + 1) + ( x + 2 ) + . . . . . . . . + (x + 100) = 5750
=> x + 1 + x + 2 + x + 3 + . . . . . . .. . .. . . . + x + 100 = 5750
=> ( 1 + 2 + 3 + . . . + 100) + ( x + x + x . . . . . . . + x ) = 5750
=> 101 . 50 + 100 x = 5750
100 x + 5050 = 5750
100 x = 5750 – 5050
100 x = 700
x = 7
101 . 50 + 100 x = 5750
100 x + 5050 = 5750
100 x = 5750 – 5050
100 x = 700
x = 7
Câu 1. a). 2A = 8 + 2 3 + 2 4 + . . . + 2 21.
=> 2A – A = 2 21 +8 – ( 4 + 2 2 ) + (2 3 – 2 3) +. . . + (2 20 – 2 20). = 2 21.
b). (x + 1) + ( x + 2 ) + . . . . . . . . + (x + 100) = 5750
=> x + 1 + x + 2 + x + 3 + . . . . . . .. . .. . . . + x + 100 = 5750
=> ( 1 + 2 + 3 + . . . + 100) + ( x + x + x . . . . . . . + x ) = 5750
=> 101 . 50 + 100 x = 5750
100 x + 5050 = 5750
100 x = 5750 – 5050
100 x = 700
x = 7