Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
a) Ta có, theo quy tắc ba điểm của phép trừ:
=
–
(1)
Mặt khác, =
(2)
Từ (1) và (2) suy ra:
=
–
.
b) Ta có : =
–
(1)
=
(2)
Từ (1) và (2) cho ta:
=
–
.
c) Ta có :
–
=
(1)
–
=
(2)
=
(3)
Từ (1), (2), (3) suy ra đpcm.
d) –
+
= (
–
) +
=
+
=
+
( vì
=
) =
a) Gọi theo thứ tự ∆1, ∆2, ∆3 là giá của các vectơ ,
,
cùng phương với
=> ∆1 //∆3 ( hoặc ∆1 = ∆3 ) (1)
cùng phương với
=> ∆2 // ∆3 ( hoặc ∆2 = ∆3 ) (2)
Từ (1), (2) suy ra ∆1 // ∆2 ( hoặc ∆1 = ∆2 ), theo định nghĩa hai vectơ ,
cùng phương.
Vậy
a) đúng.
b) Đúng.
Gọi G là giao điểm của AK, BM thì G là trọng tâm của tam giác.
Ta có =
=>
=
= –
= –
= –
Theo quy tắc 3 điểm đối với tổng vec tơ:
=
+
=>
=
–
=
(
–
).
AK là trung tuyến thuộc cạnh BC nên
+
= 2
=>
–
+
= 2
Từ đây ta có =
+
=>
= –
–
.
BM là trung tuyến thuộc đỉnh B nên
+
= 2
=> –
+
= 2
=> =
+
.
Trước hết ta có
= 3
=>
= 3 (
+
)
=> = 3
+ 3
=> – = 3
=> =
mà =
–
nên
=
(
–
)
Theo quy tắc 3 điểm, ta có
=
+
=>
=
+
–
=> = –
+
hay
= –
+
Áp dụng quy tắc 3 điểm đối với phép cộng vectơ:
=
+
=
+
=> +
=
+
+ (
+
)
ABCD là hình bình hành, hi vec tơ và
là hai vec tơ đối nhau nên:
+
=
Suy ra +
=
+
.
Mình có cách khác :
Áp dụng quy tắc 3 điểm đối với phép trừ vec tơ
=
–
=
–
=> +
= (
+
) – (
+
).
ABCD là hình bình hành nên và
là hai vec tơ đối nhau, cho ta:
+
=
Suy ra: +
=
+
.
Ta xét tổng:
+
+
+
+
+
=
=
(1)
Mặt khác, ta có ABIJ, BCPQ và CARS là các hình bình hành nên:
=
=
=
=> +
+
=
+
+
=
=
(2)
Từ (1) và (2) suy ra : +
+
=
(dpcm)
Ta có +
=
=
= a
Ta có: –
=
+
.
Trên tia CB, ta dựng =
=> –
=
+
=
Tam giác EAC vuông tại A và có : AC = a, CE = 2a , suy ra AE = a√3
Vậy =
= a√3
a) Ta có = 2
= 2
+ 0
suy ra
= (2;0)
b) = (0; -3)
c) = (3; -4)
d) = (0,2; – √ 3)
N là trung điểm của CD:
2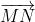
=
+ 
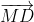(1)
Theo quy tắc 3 điểm, ta có:
Từ (1), (2), (3) ta có: 2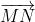
= 
+
+
+
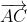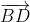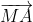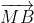
vì M là trung điểm của Ab nên: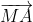
+
= 
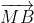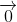
Suy ra : 2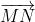
=
+
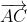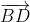
Chứng minh tương tự, ta có 2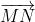
= 
+
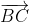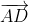
Chú ý: Sau khi chứng minh 2 C =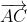
+
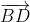ta chỉ cần chứng minh thêm 
+
= 
+
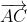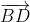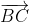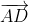cũng được
Ta có: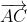
+
= 
+
+
+
=
+
+
+
= 
+
+
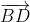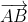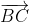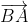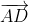
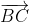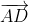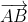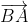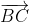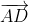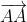
Vì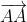
= 
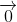nên ta có: 
+
=
+
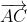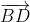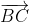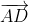
và 2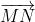
= 
+
= 
+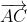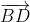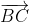