
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



Giải:
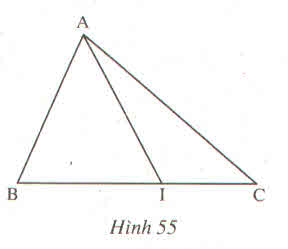
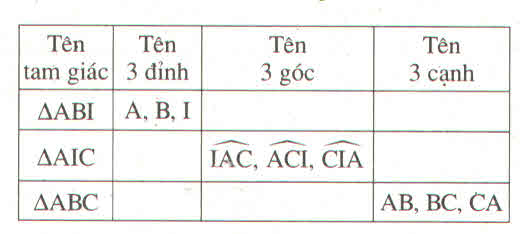
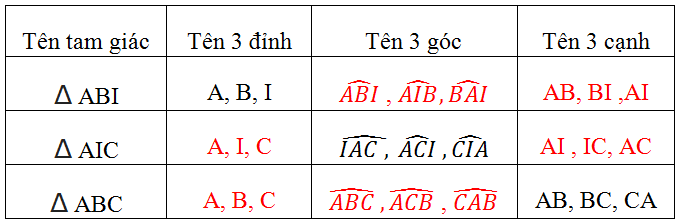
|
Tên tam giác |
Tên 3 đỉnh |
Tên 3 góc |
Tên 3 cạnh |
|
|
A,B,I |
|
AB, BI, IA |
|
|
A,I,C |
|
AI, IC, CA |
|
|
A,B,C |
|
AB, BC, CA |

Giải:
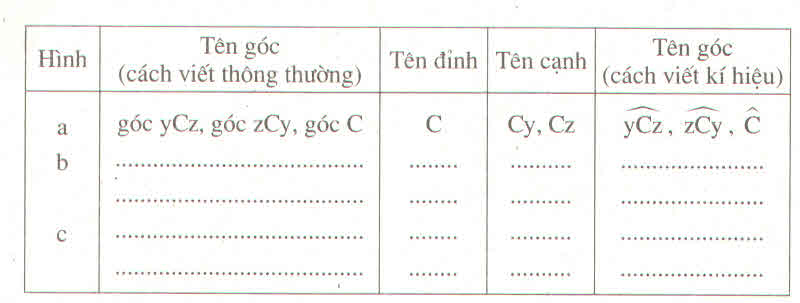
|
Hình |
Tên góc (cách viết thông thường) |
Tên đỉnh |
Tên cạnh |
Tên góc (Cách viết kí hiệu) |
|
a |
Góc yCz, góc zCy, góc C |
C |
Cy,Cz |
|
|
b |
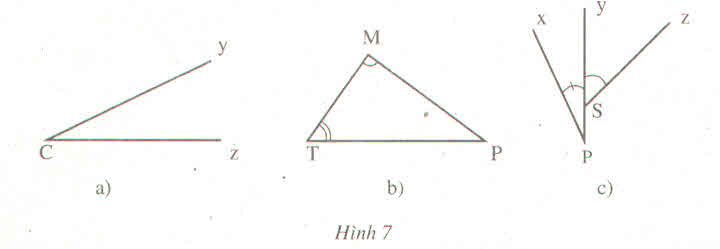
Góc MTP, PTM, T Góc TMP, PMT,M Góc TPM, MPT,P |
T M P |
TM,TP MT,MP PT,PM |
|
|
c |
Góc xPy,yPx,P Góc ySz,zSy |
P S |
Px, Py Sy, Sz |
|


Số nguyên biểu thị điểm:
- Điểm N: 2
- Điểm P: -3
- Điểm Q: -5
Số nguyên biểu thị điểm:
- Điểm N : 2
- Điểm P : -3
- Điểm Q : -5

Vẽ đường thẳng x'x . Lấy O bất kì lm gốc chung cho 2 tia đối nhau Ox , Ox' . Lấy 1 độ dài lm đơn vị . Trên mỗi tia , kể từ gốc vẽ liên tiếp các đoạn thẳng có độ dài bằng đơn vị đã chọn . Trên tia Ox , ghi các mốc liên tiếp bằng các số 0,1,2,3,... Số 0 ứng vs điểm O. Trên tia Ox' ghi các mốc liên tiếp bằng số -1 , -2 , -3 , ....


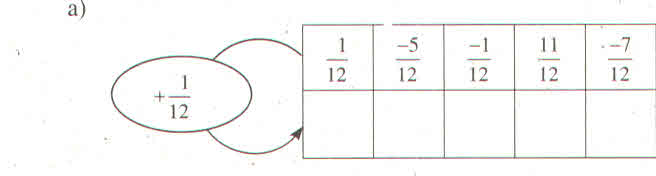
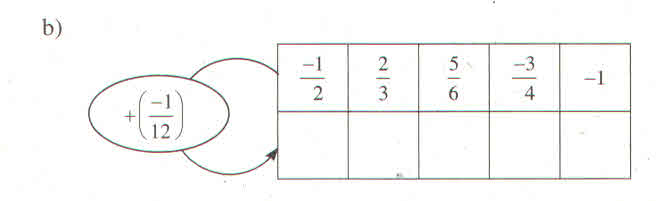


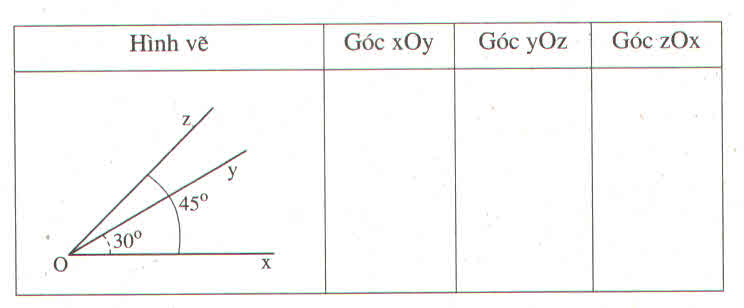
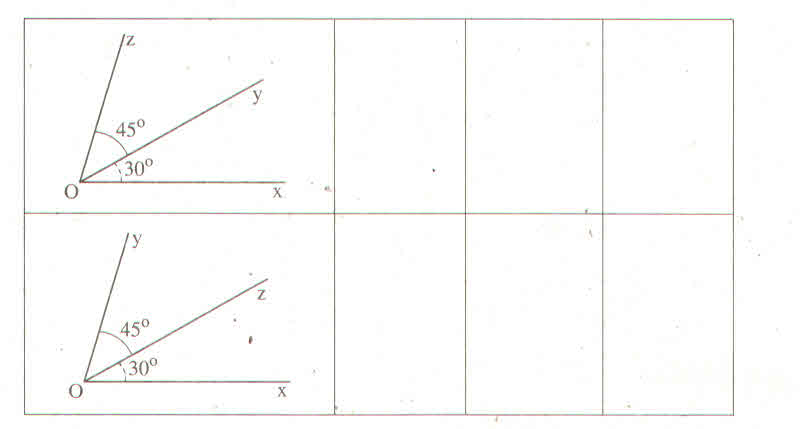
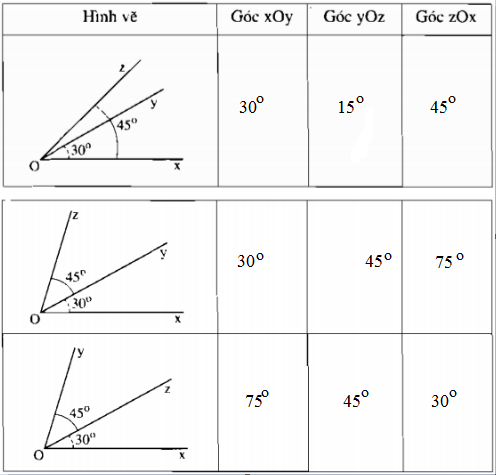
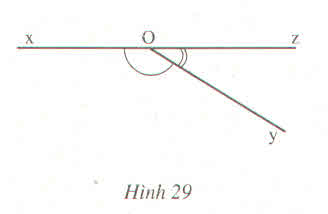




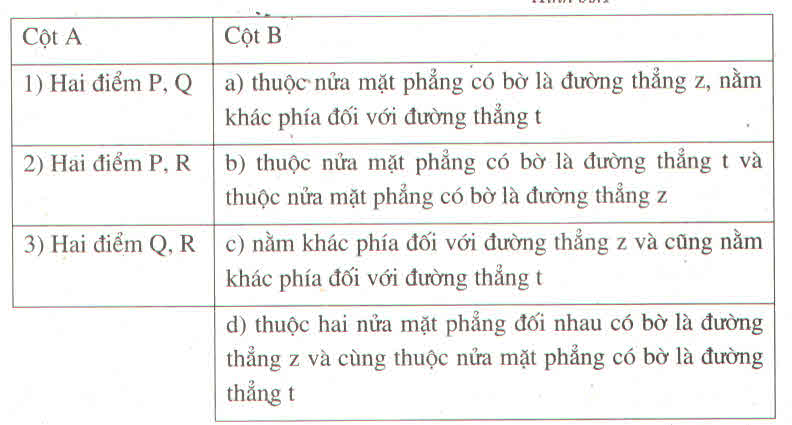


Câu 16:
a) Ta có: \(\widehat{xOz}=\dfrac{2}{5}\cdot\widehat{xOy}\)
nên \(\widehat{xOz}=\dfrac{2}{5}\cdot150^0\)
hay \(\widehat{xOz}=60^0\)
Trên cùng một nửa mặt phẳng bờ chứa tia Ox, ta có: \(\widehat{xOz}< \widehat{xOy}\left(60^0< 150^0\right)\)
nên tia Oz nằm giữa hai tia Ox và Oy
\(\Leftrightarrow\widehat{xOz}+\widehat{yOz}=\widehat{xOy}\)
\(\Leftrightarrow\widehat{yOz}+60^0=150^0\)
hay \(\widehat{yOz}=90^0\)
Vậy: \(\widehat{xOz}=60^0\); \(\widehat{yOz}=90^0\)
Câu 16:
b) Ta có: Om là tia phân giác của \(\widehat{xOz}\)(gt)
nên \(\widehat{xOm}=\dfrac{\widehat{xOz}}{2}=\dfrac{60^0}{2}\)
hay \(\widehat{xOm}=30^0\)
Trên cùng một nửa mặt phẳng bờ chứa tia Ox, ta có: \(\widehat{xOm}< \widehat{xOy}\left(30^0< 150^0\right)\)
nên tia Om nằm giữa hai tia Ox và Oy
\(\Leftrightarrow\widehat{xOm}+\widehat{yOm}=\widehat{xOy}\)
\(\Leftrightarrow\widehat{yOm}+30^0=150^0\)
hay \(\widehat{yOm}=120^0\)
Vậy: \(\widehat{xOm}=30^0\); \(\widehat{yOm}=120^0\)