
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Ta có \(\widehat{S}+\widehat{SGQ}+\widehat{Q}=180^0\Rightarrow\widehat{S}+\widehat{Q}=180^0-\widehat{SGQ}\)
Mà \(\widehat{S}-\widehat{Q}=12^0\Rightarrow\left\{{}\begin{matrix}\widehat{S}=\dfrac{180^0-\widehat{SGQ}+12^0}{2}=96^0-\dfrac{\widehat{SGQ}}{2}\\\widehat{Q}=\dfrac{180^0-\widehat{SGQ}-12^0}{2}=84^0-\dfrac{\widehat{SGQ}}{2}\end{matrix}\right.\)
Mà GP là p/g nên \(\widehat{QGP}=\widehat{PGS}=\dfrac{\widehat{SGQ}}{2}\)
\(\Rightarrow\widehat{Q}=84^0-\widehat{QGP}\)
Ta có \(\widehat{GPS}=\widehat{Q}+\widehat{QGP}=84^0-\widehat{QGP}+\widehat{QGP}=84^0\) (tc góc ngoài)

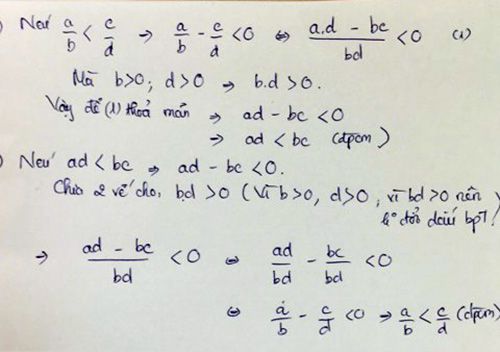
Làm nhắn gọn hơn thì
1
a/b < c/d
=> ad/bd < cb/db
=> ad < cb
2
ad < cb
=>ad /bd < cb/bd
Chúc pn hc tốt

hhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhh

Vế phải = 0 ?
\(\Leftrightarrow2x^2-2x-5x+5=0\)
\(\Leftrightarrow2x\left(x-1\right)-5\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(2x-5\right)=0\)
\(\Leftrightarrow\hept{\begin{cases}2x-5=0\\x-1=0\end{cases}}\)
Rồi giải nốt đi

bạn giúp mình bài 7 trang 10 toán 7 ki 1 nhé
bạn có sdt ko cho mình xin với

gọi độ dài 2 cạnh góc vuông lần lượt là a,b
theo đề bài ta có a/7=b/24
đặt a/7 =b/24=k
=>a=k7 va b=k24
=>k7.k24:2=366
=> k2=61/14
=>
bạn ơi mình giải ra số số căn bậc liệu có đúng đề ko

x^2+5.x<0
=> x.(x+5)<0
=>x<0 hoặc x+5<0
x+5<0=> x<-5
vậy chỉ cần x<-5 là x cũng bé hơn 0
x^2+5.x<0
=>(x+5)<0
=>x<0 hoặc x+5<0
x+5<0=>x<-5
Vậy x-<-5 là x <0
\(\dfrac{7-y}{3}=\dfrac{6-2y}{2}\)
\(\Rightarrow3\left(6-2y\right)=2\left(7-y\right)\)
\(\Rightarrow18-6y=14-2y\Rightarrow4y=4\Rightarrow y=1\)
Thanks