
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



Bài 3 :
A B S M C P N x y 1 2 z 1 2
a) Kéo dài tia NM và NM cắt BC tại S
Khi đó ta có :
\(\hept{\begin{cases}\widehat{ABC}=\widehat{BSM}\left(\text{ 2 góc so le trong }\right)\\\widehat{MNP}=\widehat{BSM}\left(\text{ 2 góc so le trong }\right)\end{cases}}\Rightarrow\widehat{ABC}=\widehat{MNP}\Rightarrow\widehat{MNP}=40^o\)
b) Vẽ \(\hept{\begin{cases}\text{Bx là tia phân giác của }\widehat{ABC}\\\text{Ny là tia phân giác của }\widehat{MNP}\end{cases}}\)
\(\Rightarrow\widehat{B_1}=B_2=\widehat{N_1}=\widehat{N_2}=\frac{\widehat{ABC}}{2}=\frac{\widehat{MNP}}{2}=\frac{40^o}{2}=20^o\left(\text{do }\widehat{ABC}=\widehat{MNP}\right)\)
Vẽ Sz // Bx => \(\widehat{B_2}=\widehat{S_1}\)
Lại có \(\widehat{BSN}=\widehat{MSP}\Rightarrow\frac{\widehat{BSN}}{2}=\frac{\widehat{MSP}}{2}\Rightarrow\widehat{S_2}=\widehat{N_1}\)mà \(\widehat{S_2}\text{ và }\widehat{N_1}\)là 2 góc so le trong
=> Sz // Ny mà Sz // Bx => Bx // Ny hay tia phân giác của 2 góc \(\widehat{ABC}\text{ và }\widehat{MNP}\)song song nhau

Bài 1:
x y m B A C 1 1 2 1
Qua B, vẽ tia Bm sao cho Bm // Ax
Bm // Ax ( cách vẽ ) => góc A1 + góc B1 = 180o ( trong cùng phía )
Mà góc A1 = 140o ( giả thiết ) => góc B1 = 40o
Ta có: góc B1 + góc B2 = góc ABC
Mà góc ABC = 70o ( giả thiết ); góc B1 = 40o ( chứng minh trên )
=> góc B2 = 30o
Ta có: góc B2 + góc C1 = 30o + 150o = 180o
Mà hai góc này ở vị trí trong cùng phía
=> Bm // Cy ( dấu hiệu nhận biết 2 đường thẳng song song )
Ta lại có:
Ax // Bm ( cách vẽ ); Cy // Bm ( chứng minh trên )
=> Ax // Cy ( tính chất 3 quan hệ từ vuông góc đến song song ) ( đpcm )
Bài 3:
A B C F E G N M H 1 2
a) Chứng minh AH < \(\dfrac{1}{2}\) ( AB + AC )
+) Vì AH vuông góc với BC ( giả thiết )
=> AH < AB ( quan hệ giữa đường vuông góc và đường xiên ) ( 1 )
+) Vì AH vuông góc với BC ( giả thiết )
=> AH < AC ( quan hệ giữa đường vuông góc và đường xiên ) ( 2 )
+) Từ ( 1 ) và ( 2 ) => AH + AH < AB + AC
=> 2 . AH < AB + AC
=> AH < \(\dfrac{1}{2}\) ( AB + AC ) ( đpcm )
b) Chứng minh EF = BC
+) Vì BM là đường trung tuyến của tam giác ABC ( giả thiết )
=> \(\dfrac{BG}{BM}=\dfrac{2}{3}\)
=> \(\dfrac{MG}{BG}=\dfrac{1}{2}\)
=> 2 . MG = BG
Mà EM = MG ( do BM là đường trung tuyến của tam giác ABC )
=> EM + MG = BG => EG = BG
+) Vì CN là đường trung tuyến của tam giác ABC ( giả thiết )
=> \(\dfrac{CG}{CN}=\dfrac{2}{3}\)
=> \(\dfrac{GN}{CG}=\dfrac{1}{2}\)
=> 2 . GN = CG
Mà FN = GN ( do CN là đường trung tuyến của tam giác ABC )
=> FN + GN = CG => FG = CG
Góc G1 = góc G2 ( đối đỉnh )
Xét tam giác FEG và tam giác CBG có:
FG = CG ( chứng minh trên )
EG = BG ( chứng minh trên )
Góc G1 = góc G2 ( chứng minh trên )
=> tam giác FEG = tam giác CBG ( c.g.c )
=> EF = BC ( 2 cạnh tương ứng ) ( đpcm )


Giải:
Do \(\left(2016a+13b-1\right)\left(2016^a+2016a+b\right)\) \(=2015\)
Nên \(2016a+13b-1\) và \(2016^a+2016a+b\) là 2 số lẻ \((*)\)
Ta xét 2 trường hợp:
Trường hợp 1: Nếu \(a\ne0\) thì \(2016^a+2016a\) là số chẵn
Do \(2016^a+2016a+b\) lẻ \(\Rightarrow b\) lẻ
Với \(b\) lẻ \(\Rightarrow13b-1\) chẵn do đó \(2016a+13b-1\) chẵn (trái với \((*)\))
Trường hợp 2: Nếu \(a=0\) thì:
\(\left(2016.0+13b-1\right)\left(2016^0+2016.0+b\right)\) \(=2015\)
\(\Leftrightarrow\left(13b-1\right)\left(b+1\right)=2015=1.5.13.31\)
Do \(b\in N\Rightarrow\left(13b-1\right)\left(b+1\right)=5.403=13.155\) \(=31.65\)
Và \(13b-1>b+1\)
\(*)\) Nếu \(b+1=5\Rightarrow b=4\Rightarrow13b-1=51\) (loại)
\(*)\) Nếu \(b+1=13\Rightarrow b=12\Rightarrow13b-1=155\) (chọn)
\(*)\) Nếu \(b+1=31\Rightarrow b=30\Rightarrow13b-1=389\) (loại)
Vậy \(\left(a,b\right)=\left(0;12\right)\)



3. Xét tam giác ADM và tam giác AEM có :
góc ADM = góc AEM = 90 độ
Góc BAM = góc CAM (gt)
AM chung
=>Tam giác ADM = tam giác AEm (c.huyền - g.nhọn)
=>MD = ME (cặp cạnh t/ứng )
AD = AE (cặp cạnh t/ứng )
Xét tam giác MDB và tam giác MEC có :
MB = MC (gt)
góc MDB = góc MEC = 90 độ
MD = ME ( câu a)
=>Tam giác MDB = Tam giác MEC (c.huyền-c.g.vuông)
Vì AD + DB = AB
AE + EC = AC
Mà AD = AE
DB = EC
=>AB = AC
Xét tam giác ABM và tam giác ACM có
AM chung
góc BAM = góc CAM (gt)
AB = AC (CMT)
=>Tam giác ABM = Tam giác ACM (c.huyền-g.nhon)
Vậy có 3 cặp tam giác bằng nhau

1/ Ta có hình vẽ:
A C B I E F H
a/ Ta có: BE và CF là các đường cao của tam giác ABC.
Mà BE cắt CF tại H
=> H là trực tâm của tam giác
H thuộc AI
=> AI cũng là đường cao của tam giác ABC.
Vì tam giác ABC cân tại A
=> AI cũng là trung tuyến của tam giác
=> I là trung điểm của BC.
b/ Xét hai tam giác vuông ABE và ACF có:
A: góc chung
AB = AC (t/g ABC cân)
=> tam giác ABE = tam giác ACF
=> AE = AF.
Ta có: AB = AC (GT)
==> AB - AF = AC - AE
hay BF = CE
Xét tam giác BFI và tam giác CEI có:
góc B = góc C (t/g ABC cân)
BI = IC (I là trung điểm BC)
BF = CE (cmt)
=> tam giác BFI = tam giác CEI
=> IF = IE
Vậy tam giác IEF cân tại I
Bài 1:
Ta có BE, CF là đường cao TG ABC
=> AI là đường cao thứ 3 của TG ABC
Mà TG ABC cân AI cũng là đường phân giác
=> BAI = CAI
Xét TG ABI và TG ACI
AIB= AIC = 90 độ
AB = AC( TG ABC cân tại A)
BAI= CAI( cmt)
=> TG ABI= TG ACI
=> BI= IC
=> I là trung điểm BC
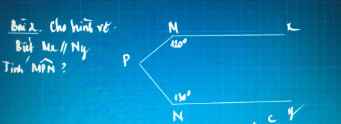










 M.n giải hộ em vs đg cần gấp ạ
M.n giải hộ em vs đg cần gấp ạ
Kẻ Pz//Mx//Ny
\(\Rightarrow\left\{{}\begin{matrix}\widehat{MPz}+\widehat{PMx}=180^0\\\widehat{NPz}+\widehat{PNy}=180^0\end{matrix}\right.\)(trong cùng phía)
\(\Rightarrow\left\{{}\begin{matrix}\widehat{MPz}=180^0-\widehat{PMx}=180^0-120^0=60^0\\\widehat{NPz}=180^0-\widehat{PNy}=180^0-130^0=50^0\end{matrix}\right.\)
\(\Rightarrow\widehat{MPN}=\widehat{MPz}+\widehat{NPz}=60^0+50^0=110^0\)
Kẻ Pz//Mx//Ny
\(\Rightarrow\left\{{}\begin{matrix}\widehat{xMP}+\widehat{MPz}=180^0\\\widehat{yNP}+\widehat{NPz}=180^0\end{matrix}\right.\left(trong.cùng.phía\right)\\ \Rightarrow\left\{{}\begin{matrix}\widehat{MPz}=180^0-120^0=60^0\\\widehat{NPz}=180^0-150^0=30^0\end{matrix}\right.\\ \Rightarrow\widehat{MPN}=\widehat{MPz}+\widehat{NPz}=90^0\)