
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a: CH=16^2/24=256/24=32/3(cm)
BC=24+32/3=104/3cm
AC=căn 32/3*104/3=16/3*căn 13(cm)
b: BC=12^2/6=144/6=24cm
CH=24-6=18cm
AC=căn 18*24=12*căn 3(cm)

g: \(=\dfrac{x^2+2x-x^2-4x-2x+4}{x\left(x-2\right)\left(x+2\right)}=\dfrac{-4x+4}{x\left(x-2\right)\left(x+2\right)}\)
h: \(=\dfrac{2x^2+1-x^2+1-x^2+x-1}{\left(x+1\right)\left(x^2-x+1\right)}\)
\(=\dfrac{x+1}{\left(x+1\right)\left(x^2-x+1\right)}=\dfrac{1}{x^2-x+1}\)
\(e,=\dfrac{1}{x-1}-\dfrac{2x}{\left(x^2+1\right)\left(x-1\right)}=\dfrac{x^2-2x+1}{\left(x^2+1\right)\left(x-1\right)}=\dfrac{\left(x-1\right)^2}{\left(x^2+1\right)\left(x-1\right)}=\dfrac{x-1}{x^2+1}\\ f,=\dfrac{3x-1}{2\left(3x+1\right)}+\dfrac{3x+1}{2\left(3x-1\right)}-\dfrac{6x}{\left(3x-1\right)\left(3x+1\right)}\\ =\dfrac{9x^2-6x+1+9x^2+6x+1-12x}{2\left(3x-1\right)\left(3x+1\right)}=\dfrac{2\left(3x-1\right)^2}{2\left(3x-1\right)\left(3x+1\right)}=\dfrac{3x-1}{3x+1}\)
\(g,=\dfrac{x}{x\left(x-2\right)}-\dfrac{x^2+4x}{x\left(x-2\right)\left(x+2\right)}-\dfrac{2}{x\left(x+2\right)}\\ =\dfrac{x^2+2x-x^2-4x-2x+4}{x\left(x-2\right)\left(x+2\right)}=\dfrac{-4x+4}{x\left(x-2\right)\left(x+2\right)}\\ h,=\dfrac{2x^2+1-x^2+1-x^2+x-1}{\left(x+1\right)\left(x^2-x+1\right)}=\dfrac{x+1}{\left(x+1\right)\left(x^2-x+1\right)}=\dfrac{1}{x^2-x+1}\)

Bài 1:
\(a,\dfrac{25}{14x^2y}=\dfrac{75y^4}{42x^2y^5};\dfrac{14}{21xy^5}=\dfrac{28x}{42x^2y^5}\\ b,\dfrac{3x+1}{12xy^4}=\dfrac{3x\left(3x+1\right)}{36x^2y^4};\dfrac{y-2}{9x^2y^3}=\dfrac{4y\left(y-2\right)}{36x^2y^4}\\ c,\dfrac{1}{6x^3y^2}=\dfrac{6y^2}{36x^3y^4};\dfrac{x+1}{9x^2y^4}=\dfrac{4x\left(x+1\right)}{36x^3y^4};\dfrac{x-1}{4xy^3}=\dfrac{9x^2y\left(x-1\right)}{36x^3y^4}\\ d,\dfrac{3+2x}{10x^4y}=\dfrac{12y^4\left(3+2x\right)}{120x^4y^5};\dfrac{5}{8x^2y^2}=\dfrac{75x^2y^3}{120x^4y^5};\dfrac{2}{3xy^5}=\dfrac{80x^3}{120x^4y^5}\)

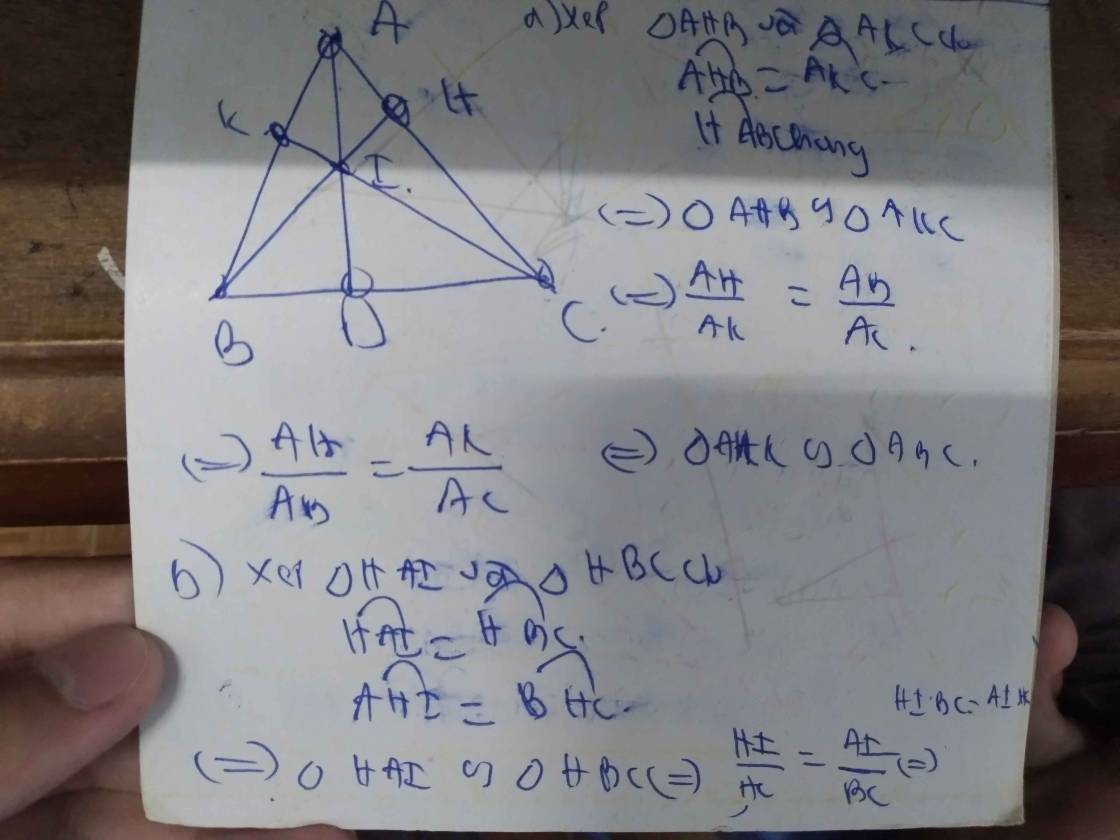
b: Sửa đề: AO vuông góc BI
Gọi M là trung điểm của IC
Xét ΔIHC có IO/IH=IM/IC
nên OM//HC và OM=1/2HC
=>OM vuông góc AH
Xet ΔAHM có
MO,HI là đường cao
MO cắt HI tại O
=>O là trực tâm
=>AO vuông góc HM
=>AO vuông góc BI

Bài I
\(1,=x\left(x-y\right)\\ 2,=x\left(y+1\right)+y+1=\left(x+1\right)\left(y+1\right)\\ 3,=x\left(x^2-2x-5x+10\right)=x\left(x-2\right)\left(x-5\right)\)
Bài II
\(1,=x-x^2+x^2-x-2=-2\\ 2,\Leftrightarrow x^2+6x+9-x^2=45\\ \Leftrightarrow6x=36\Leftrightarrow x=6\)
Bài III
\(1,A=\dfrac{4-9}{3\left(2+5\right)}=\dfrac{-5}{3\cdot7}=-\dfrac{5}{21}\\ 2,B=\dfrac{x^2-3x+2x^2+6x-3x^2-9}{\left(x-3\right)\left(x+3\right)}=\dfrac{3\left(x-3\right)}{\left(x-3\right)\left(x+3\right)}=\dfrac{3}{x+3}\\ 3,P=AB=\dfrac{\left(x-3\right)\left(x+3\right)}{3\left(x+5\right)}\cdot\dfrac{3}{x+3}=\dfrac{x-3}{x+5}=1-\dfrac{8}{x+5}\in Z\\ \Leftrightarrow x+5\inƯ\left(8\right)=\left\{-8;-4;-2;-1;1;2;4;8\right\}\\ \Leftrightarrow x\in\left\{-13;-9;-7;-6;-4;-1;3\right\}\)

\(e,\left(x^3+3x^2y-2xy^2\right)\left(-3x^2y\right)\)
\(=\left(-3x^2.x^3.y\right)+\left(-3x^2y.3x^2y\right)+\left(-3x^2y.\left(-2xy^2\right)\right)\)
\(=-3x^5y-9x^4y^2+6x^3y^3\)
\(f,\left(4x^2-\dfrac{3}{2}xy+\dfrac{1}{4}\right)\left(-10xy^2\right)\)
\(=\left(-10xy^2.4x^2\right)+\left(-10xy^2.\left(-\dfrac{3}{2}xy\right)\right)+\left(-10xy^2.\dfrac{1}{4}\right)\)
\(=-40x^3y^2+15x^2y^3-\dfrac{5}{2}xy^2\)



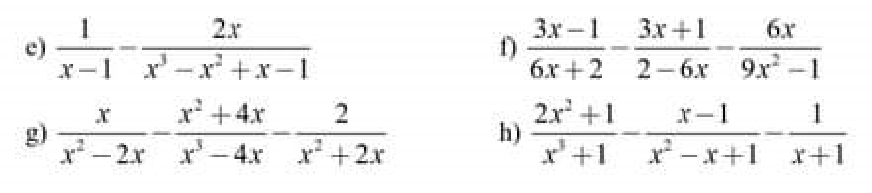







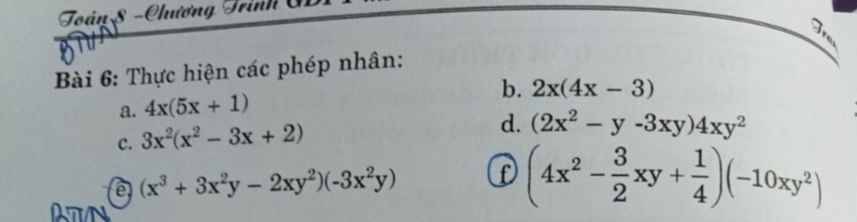
a: BE/BC=4/12=1/3
BF/BA=3/9=1/3
=>BE/BC=BF/BA
b: BF/BA=BE/BC
=>BF/BE=BA/BC
=>ΔBFA đồng dạng với ΔBEC