VT
3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
BT
1
5 tháng 2 2023
\(\Leftrightarrow\left(\dfrac{x+1}{2022}+1\right)+\left(\dfrac{x+3}{2020}+1\right)+\left(\dfrac{x+5}{2018}+1\right)+\left(\dfrac{x+7}{2016}+1\right)=0\)
=>x+2023=0
=>x=-2023
20 tháng 4 2020
\(\frac{x-2}{2017}+\frac{x-3}{2018}=\frac{x-4}{2019}+\frac{x-5}{2020}\)
<=> \(\frac{x-2}{2017}+1+\frac{x-3}{2018}+1=\frac{x-4}{2019}+1+\frac{x-5}{2020}+1\)
<=> \(\frac{x+2015}{2017}+\frac{x+2015}{2018}-\frac{x+2015}{2019}-\frac{x+2015}{2020}=0\)
<=> \(\left(x+2015\right)\left(\frac{1}{2017}+\frac{1}{2018}-\frac{1}{2019}-\frac{1}{2020}\right)=0\)
<=> x + 2015 = 0 ( vì \(\frac{1}{2017}+\frac{1}{2018}-\frac{1}{2019}-\frac{1}{2020}\ne0\))
<=> x = - 2015
Vậy x = -2015.
20 tháng 4 2020
Giải phương trình :
\(\frac{x-2}{2017}+\frac{x-3}{2018}=\frac{x-4}{2019}+\frac{x-5}{2020}\)
\(\Rightarrow\frac{x-2}{2017}+1+\frac{x-3}{2018}+1=\frac{x-4}{2019}+1+\frac{x-5}{2020}+1\)
\(\Rightarrow\frac{x+2015}{2017}+\frac{x+2015}{2018}-\frac{x+2015}{2019}-\frac{x+2015}{2020}=0\)
\(\Rightarrow\left(x+2015\right)\left(\frac{1}{2017}+\frac{1}{2018}-\frac{1}{2019}-\frac{1}{2020}\right)=0\)
Mà \(\left(\frac{1}{2017}+\frac{1}{2018}-\frac{1}{2019}-\frac{1}{2020}\right)>0\)
\(\Rightarrow x+2015=0\)
\(\Rightarrow x=-2015\)
24 tháng 5 2022
Nhận thấy vế trái luôn dương nên \(x-2020\ge0\Leftrightarrow x\ge2020\)
Với \(x\ge2020\Rightarrow\left\{{}\begin{matrix}x-2017\ge0\\2x-2018\ge0\\3x-2019\ge0\end{matrix}\right.\)
PT trở thành: \(x-2017+2x-2018+3x-2019=x-2020\)
Hay kết hợp với điều kiện \(x=\dfrac{4034}{5}\) suy ra PT đã cho vô nghiệm
P
0
TT
1 tháng 3 2020
1) Phương trình ban đầu tương đương :
\(\left(2021x-2020\right)^3=\left(2x-2\right)^3+\left(2019x-2018\right)^3\)
Đặt \(a=2x-2,b=2019x-2018\)
\(\Rightarrow a+b=2021x-2020\)
Khi đó phương trình có dạng :
\(\left(a+b\right)^3=a^3+b^3\)
\(\Leftrightarrow3ab\left(a+b\right)=0\)
\(\Leftrightarrow3\cdot\left(2x-2\right)\cdot\left(2019x-2018\right)\cdot\left(2021x-2002\right)=0\)
\(\Leftrightarrow\)Hoặc \(2x-2=0\)
Hoặc \(2019x-2018=0\)
Hoặc \(2021x-2020=0\)
\(\Rightarrow x\in\left\{1,\frac{2018}{2019},\frac{2020}{2021}\right\}\) (thỏa mãn)
Vậy : phương trình đã cho có tập nghiệm \(S=\left\{1,\frac{2018}{2019},\frac{2020}{2021}\right\}\)
KN
1 tháng 3 2020
\(x\left(2x-3\right)+x\left(x-m\right)=3x^2+x-m\)
\(\Leftrightarrow2x^2-3x+x^2-xm=3x^2+x-m\)
\(\Leftrightarrow-3x-xm=x-m\)
\(\Leftrightarrow4x+xm=m\Leftrightarrow x\left(4+m\right)=m\)
\(\Leftrightarrow x=\frac{m}{m+4}\)
Phương trình có nghiệm không âm \(\Leftrightarrow x\ge0\)
\(\Rightarrow\frac{m}{m+4}\ge0\)
Mà \(m+4>m\)nên \(\orbr{\begin{cases}m\ge0\\m+4\le0\end{cases}}\Leftrightarrow\orbr{\begin{cases}m\ge0\\m\le-4\end{cases}}\)
NN
2
2 tháng 2 2022
\(\Rightarrow x+1+2x-2020=3x-2019\Leftrightarrow3x-2019=3x-2019\)
Vậy pt có vô số nghiệm
16 tháng 4 2020
Ta có:\(\frac{x-2}{2017}+1+\frac{x-3}{2018}+1=\frac{x-4}{2019}+1+\frac{x-5}{2020}+1\)
\(\Rightarrow\frac{x+2015}{2017}+\frac{x+2015}{2018}-\frac{x+2015}{2019}-\frac{x+2015}{2020}=0\)
\(\Rightarrow\left(x+2015\right)\left(\frac{1}{2017}+\frac{1}{2018}-\frac{1}{2019}-\frac{1}{2020}\right)=0\)
Mà \(\frac{1}{2017}+\frac{1}{2018}-\frac{1}{2019}-\frac{1}{2020}>0\)
\(\Rightarrow x+2015=0\Rightarrow x=-2015\)
\(S=\left\{-2015\right\}\)
H9
HT.Phong (9A5)
CTVHS
12 tháng 3 2023
a) \(3\left(2x-x\right)=5x+1\)
\(\Leftrightarrow6x-3x=5x+1\)
\(\Leftrightarrow6x-3x-5x=1\)
\(\Leftrightarrow-2x=1\)
\(\Leftrightarrow x=\dfrac{1}{-2}=-\dfrac{1}{2}\)
b) \(\dfrac{x+1}{2021}+\dfrac{x+2}{2020}+\dfrac{x+3}{2019}+\dfrac{x+4}{2018}=0\)
\(\Leftrightarrow\dfrac{x+1}{2021}+1+\dfrac{x+2}{2020}+1=\dfrac{x+3}{2019}+1+\dfrac{x+4}{2018}+1\)
\(\Leftrightarrow\dfrac{x+2022}{2021}+\dfrac{x+2022}{2020}=\dfrac{x+2022}{2019}+\dfrac{x+2022}{2018}\)
\(\Leftrightarrow\left(x+2022\right)\left(\dfrac{1}{2021}+\dfrac{1}{2020}+\dfrac{1}{2019}+\dfrac{1}{2018}\right)\)
\(\Leftrightarrow x+2022=0\)
\(\Leftrightarrow x=-2022\)
HH
1
TT
15 tháng 5 2021
\(\dfrac{x+1}{2021}+\dfrac{x+2}{2020}=\dfrac{x+3}{2019}+\dfrac{x+4}{2018}\)
=>\(\dfrac{x+1}{2021}+1+\dfrac{x+2}{2020}+1=\dfrac{x+3}{2019}+1+\dfrac{x+4}{2018}+1\)
=>\(\dfrac{x+2022}{2021}+\dfrac{x+2022}{2020}=\dfrac{x+2022}{2019}+\dfrac{x+2022}{2018}\)
=> (x+2022)(\(\dfrac{1}{2021}+\dfrac{1}{2020}-\dfrac{1}{2019}-\dfrac{1}{2018}\))=0
=>x+2022=0
=> x=-2022
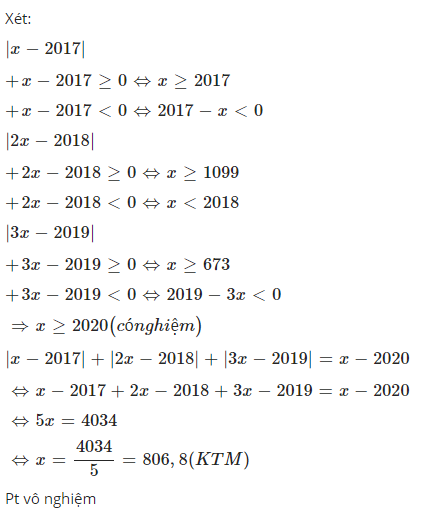
\(\left(x-2018\right)^3+\left(x-2020\right)^3=\left(2x-4038\right)\)
\(\Leftrightarrow\left(x-2018\right)^3+\left(x-2020\right)^3+\left(4038-2x\right)^3=0^{^{\left(1\right)}}\)
Áp dụng bđt \(a+b+c=0\Leftrightarrow a^3+b^3+c^3=3abc\)
Ta có \(\left(x-2018\right)+\left(x-2020\right)+\left(4038-2x\right)=0\)
\(\Leftrightarrow\left(x-2018\right)^3+\left(x-2020\right)^3+\left(4038-2x\right)^3=3\left(x-2018\right)\left(x-2020\right)\left(4038-2x\right)\)
Do đó (1) \(\Leftrightarrow3\left(x-2018\right)\left(x-2020\right)\left(4038-x\right)=0\)
<=> x-2018 =0 hoặc x-2020 = 0 hoặc 4038 -2x =0
<=> x=2018 hoặc x=2020 hoặc x=2019
Vậy phương trình đã cho có nghiệm S={2018;2020;2019}
\(\left(x-2018\right)^3+\left(x-2020\right)^3=\left(2x-4038\right)^3\)
\(\Leftrightarrow\left(x-2018\right)^3+\left(x-2020\right)^3+\left(4038-2x\right)^3=0\)
ta có \(\left(x-2018\right)+\left(x-2020\right)+\left(4038-2x\right)=0\)
nên đặt \(\left(x-2018\right)=a;\left(x-2020\right)=b;\left(4038-2x\right)=c\Leftrightarrow a+b+c=0\)
Khi đó a3 + b3+c3 = 0 ( 1)
mà a+b+c=0 \(\Leftrightarrow\)a+b=-c
\(\Leftrightarrow\)(a+b)3 = -c3
\(\Leftrightarrow\)a3+b3+c3 = 3abc (2)
Từ (1) và (2) \(\Leftrightarrow\)abc=0
\(\Leftrightarrow\)\(\left(x-2018\right)=0hoặc\left(x-2020\right)=0hoặc\left(4038-2x\right)=0\)
\(\Leftrightarrow\)\(x=2018hoặcx=2020hoặcx=2019\)
Vậy tập nghiệm của PT là S={2018;2019;2020}