
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


(2,3x – 6,9).(0,1x + 2) = 0
⇔ 2,3x – 6,9 = 0 hoặc 0,1x + 2 = 0
+ 2,3x – 6,9 = 0 ⇔ 2,3x = 6,9 ⇔ x = 3.
+ 0,1x + 2 = 0 ⇔ 0,1x = -2 ⇔ x = -20.
Vậy phương trình có tập nghiệm S = {3; -20}.
c) (4x + 2)(x2 + 1) = 0
⇔ 4x + 2 = 0 hoặc x2 + 1 = 0
+ 4x + 2 = 0 ⇔ 4x = -2 ⇔ x = 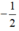
+ x2 + 1 = 0 ⇔ x2 = -1 (Phương trình vô nghiệm vì x2 ≥ 0 với mọi x ).
Vậy phương trình có tập nghiệm 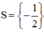

a) (3x - 2)(4x + 5) = 0
\(\Leftrightarrow\orbr{\begin{cases}3x-2=0\\4x+5=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=\frac{2}{3}\\x=-\frac{5}{4}\end{cases}}}\)
Vậy \(S=\left\{-\frac{5}{4};\frac{2}{3}\right\}\)
b) (2,3x - 6,9)(0,1x + 2) = 0
\(\Leftrightarrow\orbr{\begin{cases}2,3x-6,9=0\\0,1x+2=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=3\\x=-20\end{cases}}}\)
Vậy \(S=\left\{-20;3\right\}\)

(2,3x-6,9)(0,1x+2)=0
<=>2,3x-6,9=0
=>2,3x=6,9
=>x=3
<=>0,1x+2=0
=>0,1x=-2
=>x=-20
kl..................

a) (3x - 2)(4x + 5) = 0
⇔ 3x - 2 = 0 hoặc 4x + 5 = 0
1) 3x - 2 = 0 ⇔ 3x = 2 ⇔ x = 2323
2) 4x + 5 = 0 ⇔ 4x = -5 ⇔ x = −54−54
Vậy phương trình có tập nghiệm S = {23;−54}{23;−54}.
b) (2,3x - 6,9)(0,1x + 2) = 0
⇔ 2,3x - 6,9 = 0 hoặc 0,1x + 2 = 0
1) 2,3x - 6,9 = 0 ⇔ 2,3x = 6,9 ⇔ x = 3
2) 0,1x + 2 = 0 ⇔ 0,1x = -2 ⇔ x = -20.
Vậy phương trình có tập hợp nghiệm S = {3;-20}
c) (4x + 2)(x2 + 1) = 0 ⇔ 4x + 2 = 0 hoặc x2 + 1 = 0
1) 4x + 2 = 0 ⇔ 4x = -2 ⇔ x = −12−12
2) x2 + 1 = 0 ⇔ x2 = -1 (vô lí vì x2 ≥ 0)
Vậy phương trình có tập hợp nghiệm S = {−12}{−12}.
d) (2x + 7)(x - 5)(5x + 1) = 0
⇔ 2x + 7 = 0 hoặc x - 5 = 0 hoặc 5x + 1 = 0
1) 2x + 7 = 0 ⇔ 2x = -7 ⇔ x = −72−72
2) x - 5 = 0 ⇔ x = 5
3) 5x + 1 = 0 ⇔ 5x = -1 ⇔ x = −15−15.
Vậy phương trình có tập nghiệm là S = {−72;5;−15}{−72;5;−15}
a) (3x - 2)(4x + 5) = 0
⇔ 3x - 2 = 0 hoặc 4x + 5 = 0
1) 3x - 2 = 0 ⇔ 3x = 2 ⇔ x = 2/3
2) 4x + 5 = 0 ⇔ 4x = -5 ⇔ x = -5/4
Vậy phương trình có tập nghiệm S = {2/3;−5/4}
b) (2,3x - 6,9)(0,1x + 2) = 0
⇔ 2,3x - 6,9 = 0 hoặc 0,1x + 2 = 0
1) 2,3x - 6,9 = 0 ⇔ 2,3x = 6,9 ⇔ x = 3
2) 0,1x + 2 = 0 ⇔ 0,1x = -2 ⇔ x = -20.
Vậy phương trình có tập hợp nghiệm S = {3;-20}
c) (4x + 2)(x2 + 1) = 0 ⇔ 4x + 2 = 0 hoặc x2 + 1 = 0
1) 4x + 2 = 0 ⇔ 4x = -2 ⇔ x = −1/2
2) x2 + 1 = 0 ⇔ x2 = -1 (vô lí vì x2 ≥ 0)
Vậy phương trình có tập hợp nghiệm S = {−1/2}
d) (2x + 7)(x - 5)(5x + 1) = 0
⇔ 2x + 7 = 0 hoặc x - 5 = 0 hoặc 5x + 1 = 0
1) 2x + 7 = 0 ⇔ 2x = -7 ⇔ x = −7/2
2) x - 5 = 0 ⇔ x = 5
3) 5x + 1 = 0 ⇔ 5x = -1 ⇔ x = −1/5
Vậy phương trình có tập nghiệm là S = {−7/2;5;−1/5}

\(a,\left(x-1\right)\left(x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x+2=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-2\end{matrix}\right.\)
\(b,\left(x-2\right)\left(x-5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-2=0\\x-5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=5\end{matrix}\right.\)
\(c,\left(x+3\right)\left(x-5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\x-5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=5\end{matrix}\right.\)
\(d,\left(x+\dfrac{1}{2}\right)\left(4x+4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{1}{2}=0\\4x+4=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{1}{2}=0\\4\left(x+1\right)=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{1}{2}\\x=-1\end{matrix}\right.\)
\(e,\left(x-4\right)\left(5x-10\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-4=0\\5x-10=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=4\\x=2\end{matrix}\right.\)
\(f,\left(2x-1\right)\left(3x+6\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-1=0\\3x+6=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=-2\end{matrix}\right.\)
`a,(x-1)(x+2)=0`
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x+2=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-2\end{matrix}\right.\)
`b,(x -2)(x -5)=0`
\(\Leftrightarrow\left[{}\begin{matrix}x-2=0\\x-5=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=5\end{matrix}\right.\)
`c,(x +3)(x -5)=0`
\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\x-5=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=5\end{matrix}\right.\)
`d,(x + 1/2)(4x + 4)=0`
\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{1}{2}=0\\4x+4=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{1}{2}\\4x=-4\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{1}{2}\\x=-1\end{matrix}\right.\)
`e,(x -4)(5x -10)=0`
\(\Leftrightarrow\left[{}\begin{matrix}x-4=0\\5x-10=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=4\\5x=10\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=4\\x=2\end{matrix}\right.\)
`f,(2x -1)(3x +6)=0`
\(\Leftrightarrow\left[{}\begin{matrix}2x-1=0\\3x+6=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=1\\3x=-6\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=-2\end{matrix}\right.\)
`g,(2,3x -6,9)(0,1x -2)=0`
\(\Leftrightarrow\left[{}\begin{matrix}2,3x-6,9=0\\0,1x-2=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2,3x=6,9\\0,1x=2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=20\end{matrix}\right.\)

a: (3x-2)(4x+5)=0
=>3x-2=0 hoặc 4x+5=0
=>x=2/3 hoặc x=-5/4
b: (2,3x-6,9)(0,1x+2)=0
=>2,3x-6,9=0 hoặc 0,1x+2=0
=>x=3 hoặc x=-20
c: =>(x-3)(2x+5)=0
=>x-3=0 hoặc 2x+5=0
=>x=3 hoặc x=-5/2

a) (3x - 2)(4x + 5) = 0
⇔ 3x - 2 = 0 hoặc 4x + 5 = 0
1) 3x - 2 = 0 ⇔ 3x = 2 ⇔ x = 2/3
2) 4x + 5 = 0 ⇔ 4x = -5 ⇔ x = -5/4
Vậy phương trình có tập nghiệm S = {2/3;−5/4}
b) (2,3x - 6,9)(0,1x + 2) = 0
⇔ 2,3x - 6,9 = 0 hoặc 0,1x + 2 = 0
1) 2,3x - 6,9 = 0 ⇔ 2,3x = 6,9 ⇔ x = 3
2) 0,1x + 2 = 0 ⇔ 0,1x = -2 ⇔ x = -20.
Vậy phương trình có tập hợp nghiệm S = {3;-20}
c) (4x + 2)(x2 + 1) = 0 ⇔ 4x + 2 = 0 hoặc x2 + 1 = 0
1) 4x + 2 = 0 ⇔ 4x = -2 ⇔ x = −1/2
2) x2 + 1 = 0 ⇔ x2 = -1 (vô lí vì x2 ≥ 0)
Vậy phương trình có tập hợp nghiệm S = {−1/2}
d) (2x + 7)(x - 5)(5x + 1) = 0
⇔ 2x + 7 = 0 hoặc x - 5 = 0 hoặc 5x + 1 = 0
1) 2x + 7 = 0 ⇔ 2x = -7 ⇔ x = −7/2
2) x - 5 = 0 ⇔ x = 5
3) 5x + 1 = 0 ⇔ 5x = -1 ⇔ x = −1/5
Vậy phương trình có tập nghiệm là S = {−7/2;5;−1/5}

a) \(\left(3x-2\right)\left(4x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}3x-2=0\\4x+5=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{2}{3}\\x=-\dfrac{5}{4}\end{matrix}\right.\)
Vậy: \(S=\left\{\dfrac{2}{3};-\dfrac{5}{4}\right\}\)
b) \(\left(2,3x-6,9\right)\left(0,1x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2,3x-6,9=0\\0,1x+2=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=-20\end{matrix}\right.\)
c) \(\left(4x+2\right)\left(x^2+1\right)=0\)
Vì \(x^2+1\ge1>0\forall x\)
\(\Rightarrow4x+2=0\)
\(\Leftrightarrow x=-\dfrac{1}{2}\)
Vậy: \(S=\left\{-\dfrac{1}{2}\right\}\)
d) \(\left(2x+7\right)\left(x-5\right)\left(5x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+7=0\\x-5=0\\5x+1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{7}{2}\\x=5\\x=-\dfrac{1}{5}\end{matrix}\right.\)
Vậy: \(S=\left\{-\dfrac{7}{2};5;-\dfrac{1}{5}\right\}\)
e) \(\left(x-1\right)\left(2x+7\right)\left(x^2+2\right)=0\)
Vì \(x^2+2\ge2>0\forall x\)
\(\Rightarrow\left(x-1\right)\left(2x+7\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\2x+7=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-\dfrac{7}{2}\end{matrix}\right.\)
f) \(\left(3x+2\right)\left(x^2-1\right)=\left(9x^2-4\right)\left(x+1\right)\)
\(\Leftrightarrow\left(3x+2\right)\left(x-1\right)\left(x+1\right)-\left(3x-2\right)\left(3x+2\right)\left(x+1\right)=0\)
\(\Leftrightarrow\left[\left(3x+2\right)\left(x+1\right)\right].\left(x-1-3x+2\right)=0\)
\(\Leftrightarrow\left(3x^2+5x+2\right)\left(-2x+1\right)=0\)
\(\Leftrightarrow\left(3x^2+3x+2x+2\right)\left(-2x+1\right)=0\)
\(\Leftrightarrow\left[3x\left(x+1\right)+2\left(x+1\right)\right]\left(-2x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(3x+2\right)\left(-2x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+1=0\\3x+2=0\\-2x+1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=-\dfrac{2}{3}\\x=\dfrac{1}{2}\end{matrix}\right.\)
Vậy: \(S=\left\{-1;-\dfrac{2}{3};\dfrac{1}{2}\right\}\)


(2,3x - 6,9)(0,1x + 2) = 0
⇔ 2,3x - 6,9 = 0 hoặc 0,1x + 2 = 0
1) 2,3x - 6,9 = 0 ⇔ 2,3x = 6,9 ⇔ x = 3
2) 0,1x + 2 = 0 ⇔ 0,1x = -2 ⇔ x = -20.
Vậy phương trình có tập hợp nghiệm S = {3;-20}
Giải :
b) (2,3x - 6,9)(0,1x + 2) = 0 <=> 2,3x - 6,9 = 0 hoặc 0,1x + 2 = 0
2,3x - 6,9 = 0 <=> x = 6,9/2,3 = 3
0,1x + 2 = 0 <=> x = -2/0,1 = - 20
Vậy tập nghiệm của phương trình là S = {3 ; -20}