
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Cách 1:
(x + 1)2 = 4(x2 – 2x + 1)
⇔ (x + 1)2 - 4(x2 – 2x + 1) = 0
⇔ (x + 1)2 - 22. (x -1)2 = 0
⇔ (x + 1)2 – [ 2(x – 1)]2 =0
⇔ [(x+ 1) + 2( x- 1)]. [(x+ 1) - 2( x- 1)]= 0
⇔ ( x+1+ 2x -2) . (x+1 – 2x + 2) =0
⇔ ( 3x- 1).( 3- x) = 0
⇔ 3x – 1 = 0 hoặc 3 – x= 0
+) 3x – 1 = 0 ⇔ 3x = 1 ⇔ x = 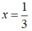
+) 3 – x = 0 ⇔ x= 3
Vậy tập nghiệm của phương trình đã cho là:
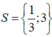
* Cách 2: Ta có:
(x + 1)2 = 4(x2 – 2x + 1)
⇔ (x + 1)2 - 4(x2 – 2x + 1) = 0
⇔ x2 + 2x +1- 4x2 + 8x – 4 = 0
⇔ - 3x2 + 10x – 3 = 0
⇔ (- 3x2 + 9x) + (x – 3) = 0
⇔ -3x (x – 3)+ ( x- 3) = 0
⇔ ( x- 3). ( - 3x + 1) = 0
⇔ x - 3 = 0 hoặc -3x + 1= 0
+) x - 3 = 0 x = 3
+) - 3x + 1 = 0 - 3x = - 1 ⇔ x = 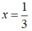
Vậy tập nghiệm của phương trình đã cho là:
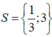
\(\left(x+1\right)^2=4\left(x^2-2x+1\right)\)
\(< =>\left(x+1\right)^2=\left(2x-2\right)^2\)
\(< =>\left(x+1-2x+2\right)\left(x+1+2x-2\right)=0\)
\(< =>\orbr{\begin{cases}-x+3=0\\3x-1=0\end{cases}}\)
\(< =>\orbr{\begin{cases}x=3\\x=\frac{1}{3}\end{cases}}\)

\(a,2x\left(x-5\right)+4\left(x-5\right)=0\\ \Leftrightarrow\left(x-5\right)\left(2x+4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-5=0\\2x+4=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=5\\2x=-4\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=5\\x=-2\end{matrix}\right.\)
Vậy \(x\in\left\{5;-2\right\}\)
\(b,3x-15=2x\left(x-5\right)\\ \Leftrightarrow3\left(x-5\right)-2x\left(x-5\right)=0\\ \Leftrightarrow\left(x-5\right)\left(-2x+3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-5=0\\-2x+3=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=5\\2x=3\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=5\\x=\dfrac{3}{2}\end{matrix}\right.\)
Vậy \(x\in\left\{5;\dfrac{3}{2}\right\}\)
\(c,\left(2x+1\right)\left(3x-2\right)=\left(5x-8\right)\left(2x+1\right)\\ \Leftrightarrow\left(2x+1\right)\left(3x-2\right)-\left(5x-8\right)\left(2x+1\right)=0\\ \Leftrightarrow\left(2x+1\right)\left(3x-2-5x+8\right)=0\\ \Leftrightarrow\left(2x+1\right)\left(-2x+6\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}2x+1=0\\-2x+6=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}2x=-1\\2x=6\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{1}{2}\\x=3\end{matrix}\right.\)
Vậy \(x\in\left\{-\dfrac{1}{2};3\right\}\)
Câu d xem lại đề

Bài 3:
b: \(\Leftrightarrow x^2\left(x+1\right)^2=0\)
hay \(x\in\left\{0;-1\right\}\)
c: \(\Leftrightarrow\left(x-1\right)\left(x^2+x+1\right)=0\)
=>x-1=0
hay x=1
d: \(\Leftrightarrow6x^2-3x-4x+2=0\)
\(\Leftrightarrow\left(2x-1\right)\left(3x-2\right)=0\)
hay \(x\in\left\{\dfrac{1}{2};\dfrac{2}{3}\right\}\)

(x + 1) ^ 2 + |x - 1| = x ^ 2 + 4
ó/x-1/=x^2+4-(x+1)^2
ó/x-1/=x^2+4-x^2-2x-1
ó/x-1/=-2x+3
Nếu x-1≥0 óx≥1 thì /x-1/=x-1
Ta có pt : x-1=-2x+3
óx+2x=3+1
ó3x=4
óx=4/3(t/m)
Nếu x-1 <0 óx<1 thì /x-1/=1-x
Ta có pt :1-x=-2x+3
ó-x+2x=3-1
óx=2(loại)
Vậy pt trình có nghiệm là x=4/3
bn oi "ó " là dấu khi và chỉ khi ("<=>") , do tải lên bị lỗi nên nó như zậy . sorry nhé !


<=>x2-3x-4=4-x2
<=>2x2-3x-8=0
\(\Delta=\left(-3\right)^2-4\cdot\left(-8\right)\cdot2=73>0\)
=> Pt đã cho có 2 nghiệm phân biệt \(x_1=\frac{3+\sqrt{73}}{4}\\ x_2=\frac{3-\sqrt{73}}{4}\)

Mình khuyên bạn thế này :
Bạn nên tách những câu hỏi ra
Như vậy các bạn sẽ dễ giúp
Và cũng có nhiều bạn giúp hơn !
Bài 1.
a) ( x - 3 )( x + 7 ) = 0
<=> x - 3 = 0 hoặc x + 7 = 0
<=> x = 3 hoặc x = -7
Vậy S = { 3 ; -7 }
b) ( x - 2 )2 + ( x - 2 )( x - 3 ) = 0
<=> ( x - 2 )( x - 2 + x - 3 ) = 0
<=> ( x - 2 )( 2x - 5 ) = 0
<=> x - 2 = 0 hoặc 2x - 5 = 0
<=> x = 2 hoặc x = 5/2
Vậy S = { 2 ; 5/2 }
c) x2 - 5x + 6 = 0
<=> x2 - 2x - 3x + 6 = 0
<=> x( x - 2 ) - 3( x - 2 ) = 0
<=> ( x - 2 )( x - 3 ) = 0
<=> x - 2 = 0 hoặc x - 3 = 0
<=> x = 2 hoặc x = 3

| x - 2 |.( x - 1 ).( x + 1 ).( x + 2 ) = 4
Bỏ dấu tuyệt đối => 2 TH xảy ra
TH1:| x - 2 |.( x - 1 ).( x + 1 ).( x + 2 ) = 4
<=>(x-2).(x-1).(x+1).(x+2)=4
<=> (x-2).(x+2).(x-1)(x+1)=4
<=> (x2- 4).(x2- 1)=4
<=>x4- x2 - 4x2 + 4 =4
<=> x4 - 5x2 +4-4=0
<=> x4 - 5x2= 0
<=>x2 ( x2 - 5 ) =0
<=> 2 TH
*x2=0=> x=0
*x2- 5 =0 => x2= \(\pm\sqrt{5}\)=> x=\(\sqrt{5}\) hoặc x=\(-\sqrt{5}\)
Vậy x=0 hoặc x=\(\sqrt{5}\); x=-\(\sqrt{5}\)
TH2:| x - 2 |.( x - 1 ).( x + 1 ).( x + 2 ) = 4
<=>(2-x).(x-1).(x+1).(x+2)=4 ( TH này là dấu - đằng trc)
<=>(2-x).(2+x).(x-1)(x+1)=4
<=>(4 - x2). (x2 - 1) =4
<=> 4x2 - 4 - x4 + x2 - 4 =0
<=> 5x2 - x4 - 8 =0
<=> 5x2 - x4 = 8
Đặt x2 = t
-t2+5t-8 = -(t2 - 5t + 8)
Ta có: (t2 - 5t + 8)
=t2 - 5t +\(\frac{25}{4}+\frac{7}{4}\)
=(t2 - 5t + \(\left(\frac{5}{2}\right)^2\)) + \(\frac{7}{4}\)
= (t+\(\frac{5}{2}\))2 + \(\frac{7}{4}\)
Vì: (t+\(\frac{5}{2}\))2 > 0 với mọi t
=> (t+\(\frac{5}{2}\))2 + \(\frac{7}{4}\) > 0 với mọi t
=> t2 - 5t + 8 > 0 với mọi t
=>-(t2 - 5t + 8) < 0 với mọi t
=> o có gt nào tm t => PT vô nghiệm
Loại TH 2
Vậy \(\Leftrightarrow\hept{\begin{cases}x=0\\x=\sqrt{5};x=-\sqrt{5}\end{cases}}\)
4x2-9=(2x+3)(3x-5)
Ta có:
(X+1)2 =4\(\left(x^2-2x+1\right)^2\)
\(\Leftrightarrow\)\(\left(x+1\right)^2=2^2\left(\left(x-1\right)^2\right)^2\)
\(\Leftrightarrow\)\(\left(x+1\right)^2=\left(2\left(x-1\right)^2\right)^2\)
\(\Leftrightarrow\)\(x+1=2\left(x-1\right)^2\)
\(\Leftrightarrow\)\(x+1=2x^2-4x+2\)
\(\Leftrightarrow\)\(2x^2-4x+2-x-1=0\)
\(\Leftrightarrow\)\(2x^2-3x+1=0\)
\(\Leftrightarrow\)\(2x^2-2x-x+1=0\)
\(\Leftrightarrow\)\(2x\left(x-1\right)-\left(x-1\right)=0\)
\(\Leftrightarrow\)\(\left(2x-1\right)\left(x-1\right)=0\)
\(\Leftrightarrow\)\(x=\frac{1}{2}\)hoặc \(x=1\)