Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(Q_{\left(x\right)}=x^{14}-10x^{13}+10x^{12}-10x^{11}+...+10x^2-10x+10\)
\(=x^{14}-\left(x+1\right)x^{13}+\left(x+1\right)x^{12}-\left(x+1\right)x^{11}+..+\left(x+1\right)x^2-\left(x+1\right)x+x+1\)
\(=x^{14}-x^{14}-x^{13}+x^{13}+x^{12}-x^{12}-x^{11}+...+x^3+x^2-x^2-x+x+1\)
\(=1\)
\(a.P(x)=x^7-80x^6+80x^5-80x^4+....+80x+15\)
\(=x^7-79x^6-x^6+79x^5+x^5-79x^4-....-x^2+79x+x+15\)
\(=x^6(x-79)-x^5(x-79)+x^4(x-79)-....-x(x-79)+x+15\)
\(=(x-79)(x^6-x^5+x^4-....-x)+x+15\)
Thay x = 79 vào biểu thức trên , ta có
\(P(79)=(79-79)(79^6-79^5+79^4-...-79)+79+15\)
\(=0+79+15\)
\(=94\)
Vậy \(P(x)=94\)khi x = 79
\(b.Q(x)=x^{14}-10x^{13}+10x^{12}-.....+10x^2-10x+10\)
\(=x^{14}-9x^{13}-x^{13}+9x^{12}+.....-x^3+9x^2+x^2-9x-x+10\)
\(=x^{13}(x-9)-x^{12}(x-9)+.....-x^2(x-9)+x(x-9)-x+10\)
\(=(x-9)(x^{13}-x^{12}+.....-x^2+x)-x+10\)
Thay x = 9 vào biểu thức trên , ta có
\(Q(9)=(9-9)(9^{13}-9^{12}+.....-9^2+9)-9+10\)
\(=0-9+10\)
\(=1\)
Vậy \(Q(x)=1\)khi x = 9
\(c.R(x)=x^4-17x^3+17x^2-17x+20\)
\(=x^4-16x^3-x^3+16x^2+x^2-16x-x+20\)
\(=x^3(x-16)-x^2(x-16)+x(x-16)-x+20\)
\(=(x-16)(x^3-x^2+x)-x+20\)
Thay x = 16 vào biểu thức trên , ta có
\(R(16)=(16-16)(16^3-16^2+16)-16+20\)
\(=0-16+20\)
\(=4\)
Vậy \(R(x)=4\)khi x = 16
\(d.S(x)=x^{10}-13x^9+13x^8-13x^7+.....+13x^2-13x+10\)
\(=x^{10}-12x^9-x^9+12x^8+.....+x^2-12x-x+10\)
\(=x^9(x-12)-x^8(x-12)+....+x(x-12)-x+10\)
\(=(x-12)(x^9-x^8+....+x)-x+10\)
Thay x = 12 vào biểu thức trên , ta có
\(S(12)=(12-12)(12^9-12^8+....+12)-12+10\)
\(=0-12+10\)
\(=-2\)
Vậy \(S(x)=-2\)khi x = 12
Hình như đây là toán lớp 7 có trong phần trắc nghiệm của thi HSG huyện
Chúc bạn học tốt , nhớ kết bạn với mình

b) Thay x+1=10 ta được:
Q(x) = \(x^{14}-\left(x+1\right)x^{13}+\left(x+1\right)x^{12}-\left(x+1\right)x^{11}+...+\left(x+1\right)x^2-\left(x+1\right)x+\left(x+1\right)\) \(=x^{14}-x^{14}-x^{13}+x^{13}+x^{12}-x^{12}-x^{11}+...+x^3+x^2-x^2-x+x+1=1\)
d) Thay x+1=13, ta được:
S(x) = \(x^{10}-\left(x+1\right)x^9+\left(x+1\right)x^8-\left(x+1\right)x^7+...+\left(x+1\right)x^2-\left(x+1\right)x+10\)
\(=x^{10}-x^{10}-x^9+x^9+x^8-x^8-x^7+...+x^3+x^2-x^2-x+10=-12+10=-2\)

áp dụng đl ta-lét vào tam giác có:
\(\dfrac{BC}{CA}=\dfrac{DE}{EA}=\dfrac{BC}{5}=\dfrac{3}{8}=>BC=\dfrac{3}{8}.5=\dfrac{15}{8}=1,875\)
X = BC + CA = 1,875 + 5 = 6,875

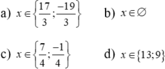
lần sau bạn ghi rõ đề bài nhé
a, \(A=x^2+10x+39=x^2+2.5.x+25+14=\left(x+5\right)^2+14\ge14\)
Dấu ''='' xảy ra khi \(x=-5\)
Vậy GTNN A là 14 khi x = -5
b, \(B=25x^2-70x+1000=\left(5x\right)^2-2.5x.7+49+951\)
\(=\left(5x-7\right)^2+951\ge951\)
Dấu ''='' xảy ra khi \(x=\frac{7}{5}\)
Vậy GTNN B là 951 khi x = 7/5
c, \(C=49x^2+64x+100=\left(7x\right)^2+2.7x.\frac{32}{7}+\frac{1024}{49}+\frac{3876}{49}\)
\(=\left(7x+\frac{32}{7}\right)^2+\frac{3876}{49}\ge\frac{3876}{49}\)
Dấu ''='' xảy ra khi \(x=-\frac{32}{49}\)
Vậy GTNN C là 3876/49 khi x = -32/49
d, \(D=5x^2+13x+41=5\left(x^2+\frac{13}{5}x+\frac{41}{5}\right)\)
\(=5\left(x^2+2.\frac{6,5}{5}.x+\frac{169}{100}+\frac{651}{100}\right)=5\left(x+\frac{6,5}{5}\right)^2+\frac{651}{20}\ge\frac{651}{20}\)
Dấu ''='' xảy ra khi \(x=-\frac{6,5}{2}\)
Vậy GTNN D là 651/20 khi x = -6,5/2