DP
giải hộ mình với các bạn
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
VB
1
các bạn giải hộ mình bài này với ạ
26 tháng 3 2022
a: Xét ΔABD và ΔACE có
AB=AC
\(\widehat{BAD}\) chung
AD=AE
Do đó: ΔABD=ΔACE
Suy ra: BD=CE
b: Xét ΔEBC và ΔDCB có
EB=DC
BC chung
CE=BD
Do đó: ΔEBC=ΔDCB
Suy ra: \(\widehat{GCB}=\widehat{GBC}\)
hay ΔGBC cân tại G
VV
5
AH
29 tháng 10 2016
\(9^{x-1}=\frac{1}{9}\)
\(9^{x-1}=9^{-1}\)
=> x - 1 = - 1
x = -1
x = 0
29 tháng 10 2016
9x-1= \(\frac{1}{9}\)
9x-1= 9-1
=> x-1=-1
x= -1-1
x= 0
tk nhé
ZS
1
UI
8 tháng 9 2019
\(\sqrt{6+\sqrt{6}+\sqrt{6}}>\sqrt{6+\sqrt{4}+\sqrt{1}}=\sqrt{6+2+1}=\sqrt{9}=3\)
Vay \(\sqrt{6+\sqrt{6}+\sqrt{6}}>3\)
Chuc ban hoc tot
9 tháng 7 2017
1) Ta có: |x+3| \(\ge\)0; |2x+y-4| \(\ge\)0
\(\Rightarrow\) |x + 3| + |2x + y - 4| \(\ge\) 0
Dấu = xảy ra khi x+3=0 và 2x+y-4 = 0 \(\Rightarrow\)x=-3; y=10
9 tháng 7 2017
1) |x + 3| + |2x + y - 4| = 0
\(\Leftrightarrow\hept{\begin{cases}x+3=0\\2x+y-4=0\end{cases}}\Leftrightarrow\hept{\begin{cases}x=-3\\-6+y-4=0\end{cases}}\Leftrightarrow\hept{\begin{cases}x=-3\\y=10\end{cases}}\)
PT
1
YD
29 tháng 12 2018
2\(^x\)+ 2\(^y\)= 2\(^{x+y}\)
=>2\(^{x+y}\)- 2\(^x\)- 2\(^y\)=0
<=>2\(^x\). 2\(^y\) - 2\(^x\)- 2\(^y\)=0
<=>2\(^x\) (2\(^y\) -1)-2y +1 =1
<=>2\(^x\) (2\(^y\) -1)-1(2y -1)=1
<=>(2\(^x\)-1)(2\(^y\) -1)=1
=>(2\(^x\)-1) và (2\(^y\)-1) bằng 1 hoặc -1
(2\(^x\)-1) = (2\(^y\)-1)=1=> x=y=1
(2\(^x\)-1) = (2\(^y\)-1)=-1 => Không tồn tại x và y
Vậy x=y=1
H
2
KV
9 tháng 10 2023
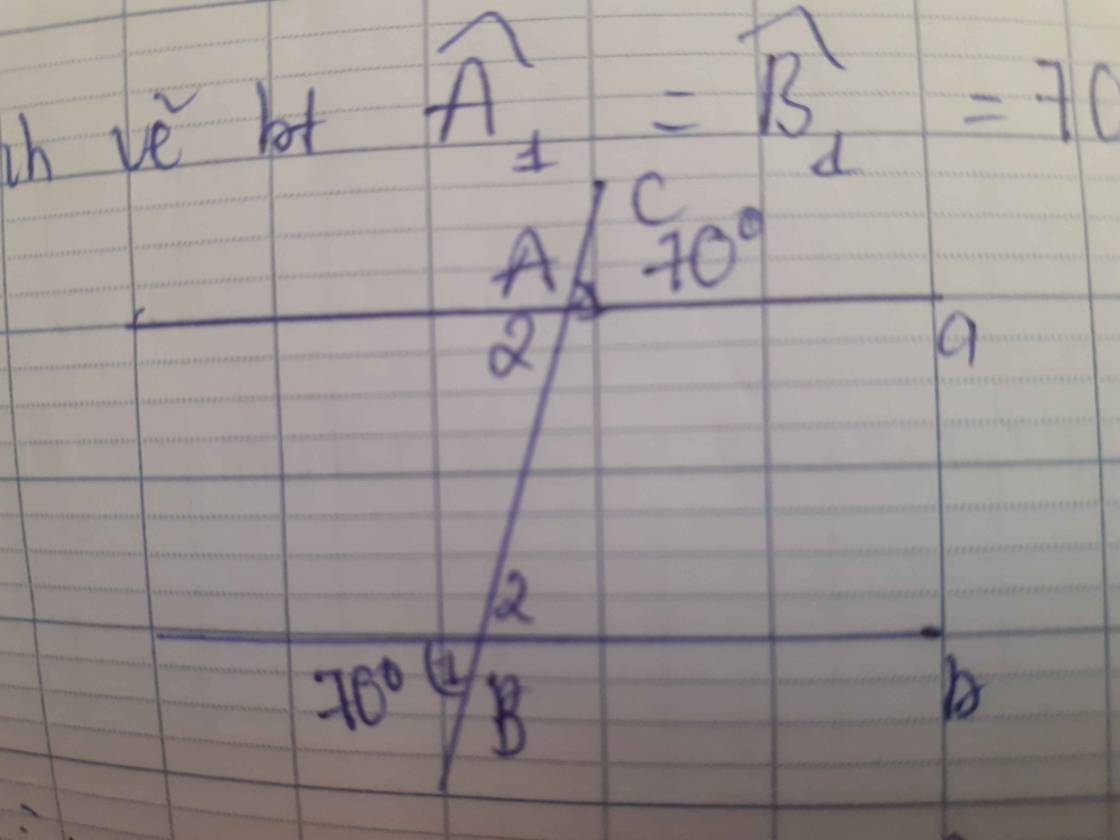
Ta có:
∠B₂ = ∠B₁ = 70⁰ (đối đỉnh)
⇒ ∠B₂ = ∠A₁ = 70⁰
Mà ∠B₂ và ∠A₁ là hai góc đồng vị
⇒ a // b
\(A=4x-18=0\)
\(4x=18\)
\(x=18\div4\)
\(x=4,5\)
Vậy đa thức A có nghiệm là x = 4,5.
\(B=3+15x=0\)
\(15x=-3\)
\(x=-3\div15\)
\(x=-0,2\)
Vậy đa thức B có nghiệm là x = -0,2.
\(C=x^2+5x+6=0\)
\(\Rightarrow x^2+2x+3x+6=0\)
\(\Rightarrow x\left(x+2\right)+3\left(x+2\right)=0\)
\(\Rightarrow\left(x+2\right)\left(x+3\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x+2=0\\x+3=0\end{cases}}\Rightarrow\orbr{\begin{cases}x=-2\\x=-3\end{cases}}\)
cảm ơn bạn nha