Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
\(\left(x-1\right)^3+x^3+\left(x+1\right)^3=\left(x+2\right)^3\)
\(\Leftrightarrow x^3-3x^2+3x-1+x^3+x^3+3x^2+3x+1-x^3-6x^2-12x-8=0\)
\(\Leftrightarrow2x^3-6x^2-6x-8=0\)
\(\Leftrightarrow2.\left(x^3-3x^2-3x-4\right)=0\)
\(\Leftrightarrow x^3-4x^2+x^2-4x+x-4=0\)
\(\Leftrightarrow x^2.\left(x-4\right)+x.\left(x-4\right)+\left(x-4\right)=0\)
\(\Leftrightarrow\left(x-4\right).\left(x^2+x+1\right)=0\)
Mà \(x^2+x+1=\left(x+\dfrac{1}{2}\right)^2+\dfrac{3}{4}>0\)
\(\Rightarrow x-4=0\Leftrightarrow x=4\)
a: =>3x+3=4x-4
=>-x=-7
hay x=7(nhận)
b: (x-1)(x-3)=0
=>x-1=0 hoặc x-3=0
=>x=1 hoặc x=3
c: 2(x-1)+x=0
=>2x-2+x=0
=>3x-2=0
hay x=2/3
a, ĐKXĐ : x ≠ 1 ; x ≠ -1
\(\Rightarrow3\left(x+1\right)=4\left(x-1\right)\)
\(\Leftrightarrow3x+3=4x-4\)
\(\Leftrightarrow-x=-7\)
\(\Leftrightarrow x=7\left(N\right)\)
b,
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=3\end{matrix}\right.\)
c,
\(\Leftrightarrow2x-2+x=0\)
\(\Leftrightarrow3x=2\)
\(\Leftrightarrow x=\dfrac{2}{3}\)
\(\dfrac{360}{x}-\dfrac{400}{x+1}=1\) (ĐK: \(x\ne0,x\ne-1\))
\(\Leftrightarrow\dfrac{360\left(x+1\right)}{x\left(x+1\right)}-\dfrac{400x}{x\left(x+1\right)}=\dfrac{x\left(x+1\right)}{x\left(x+1\right)}\)
\(\Leftrightarrow360\left(x+1\right)-400x=x\left(x+1\right)\)
\(\Leftrightarrow360x+360-400x=x^2+x\)
\(\Leftrightarrow-40x+360=x^2+x\)
\(\Leftrightarrow x^2+40x+x-360=0\)
\(\Leftrightarrow x^2+41x-360=0\)
\(\Rightarrow\Delta=41^2-4\cdot1\cdot\left(-360\right)=3121>0\)
\(\Rightarrow\left[{}\begin{matrix}x_1=\dfrac{-41+\sqrt{3121}}{2\cdot1}\approx7\left(tm\right)\\x_2=\dfrac{-41-\sqrt{3121}}{2\cdot1}\approx-48\left(tm\right)\end{matrix}\right.\)
\(\dfrac{360}{x}-\dfrac{400}{x+1}=1\)
Điều kiện: \(x\ne0;x\ne-1\)
PT \(\Leftrightarrow\dfrac{360\left(x+1\right)-400x}{x\left(x+1\right)}=1\)
\(\Rightarrow-40x+360=x\left(x+1\right)\)
\(\Leftrightarrow-40x+360=x^2+x\)
\(\Leftrightarrow x^2+41x-360=0\)
\(\Leftrightarrow x^2+2.\dfrac{41}{2}.x+\dfrac{1681}{4}=\dfrac{3121}{4}\)
\(\Leftrightarrow\left(x+\dfrac{41}{2}\right)^2=\left(\dfrac{\sqrt{3121}}{2}\right)^2\)
\(\Leftrightarrow x+\dfrac{41}{2}=\dfrac{\sqrt{3121}}{2}\) hoặc \(x+\dfrac{41}{2}=-\dfrac{\sqrt{3121}}{2}\)
\(\Leftrightarrow x=\dfrac{\sqrt{3121}}{2}-\dfrac{41}{2}\) hoặc \(x=-\dfrac{\sqrt{3121}}{2}-\dfrac{41}{2}\)
Vậy...
https://lazi.vn/edu/exercise/giai-phuong-trinh-x-1-x-22-x-1-x-4-32x-4-x-42-0-1
chỉ tiềm thấy cái này thôi ~ vì mk k thể giải đc nên nhờ mạng nên thông cảm cho nha
`1.` Với `0=0(` luôn đúng `)` `->` Kết luận: Vậy `S={x|x\inRR}`
`2.` Với `-1>0(` vô lý `)` `->` Kết luận: Vậy `S=∅`
Bài 4 :
24 phút = \(\dfrac{24}{60} = \dfrac{2}{5}\) giờ
Gọi thời gian dự định đi từ A đến B là x(giờ) ; x > 0
Suy ra quãng đường AB là 36x(km)
Khi vận tốc sau khi giảm là 36 -6 = 30(km/h)
Vì giảm vận tốc nên thời gian đi hết AB là x + \(\dfrac{2}{5}\)(giờ)
Ta có phương trình:
\(36x = 30(x + \dfrac{2}{5})\\ \Leftrightarrow x = 2\)
Vậy quãng đường AB dài 36.2 = 72(km)
\(\left(x^2-2x+3\right)\left(\frac{1}{2x}-5\right)\)
\(=\frac{x^2}{2x}-5x^2-\frac{2x}{2x}+10x+\frac{3}{2x}-15\)
\(=\frac{x^2}{2x}-5x^2-16+10x+\frac{3}{2x}\)
\(=-5x^2+\frac{x^2}{2x}+\frac{20x^2}{2x}+\frac{3}{2x}-16\)
\(=-5x^2+\frac{x^2+20x+3}{2x}-16\)
học tốt
(x^2-2x+3)(1/2x-5)=1/2x^3-5x^2-x^2+10x+3/2x-15=1/2x^3-6x^2+11,5x-15
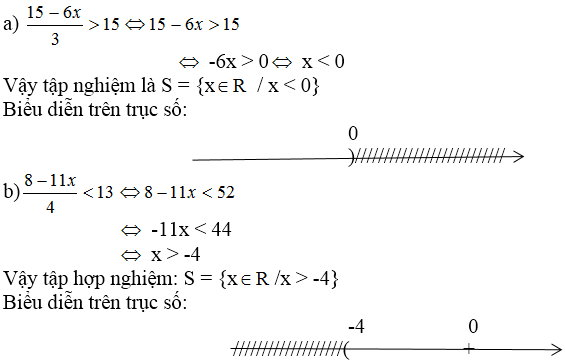
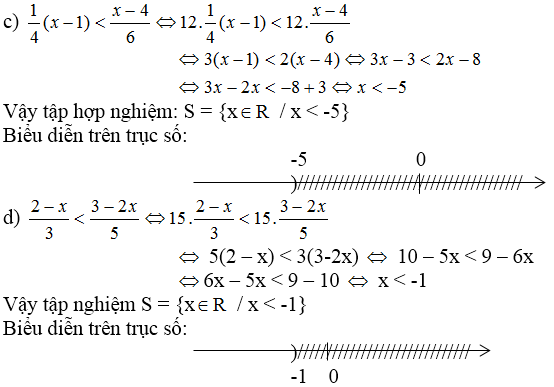
\(\dfrac{x-3}{2+x}< 1\\ \Leftrightarrow\dfrac{x-3}{2+x}-1< 0\\ \Leftrightarrow\dfrac{x-3}{2+x}-\dfrac{2+x}{2+x}< 0\\ \Leftrightarrow\dfrac{x-3-2-x}{2+x}< 0\\ \Leftrightarrow\dfrac{-5}{2+x}< 0\)
Vì \(-5< 0\)
\(\Rightarrow2+x>0\\ \Rightarrow x>-2\)
Vậy \(x>-2\)