
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Vì P,Q là trung điểm HI,HK nên PQ là đtb tg HIK
Do đó \(PQ=\dfrac{1}{2}IK=9\left(cm\right)\)
xét \(\Delta HIK\) có:
\(HP=IP\)\(\left(gt\right)\)
\(HQ=QK\left(gt\right)\)
\(\Rightarrow PQ\) là đường trung bình
\(\Rightarrow PQ=\dfrac{IK}{2}=\dfrac{18}{2}=9\left(cm\right)\)

6: \(=x^3\left(x-2\right)-\left(x-2\right)\)
\(=\left(x-2\right)\left(x-1\right)\left(x^2+x+1\right)\)
7: =(x-4)(x+2)
2/
\(2x^3-8x=2x\left(x^2-4\right)=2x\left(x-2\right)\left(x+2\right)\)
3/
\(9x^2-\left(x-1\right)^2=\left(3x\right)^2-\left(x-1\right)^2=\left(3x-x+1\right)\left(3x+x-1\right)\)
4/
\(x^2-3x+6y-4y^2=x^2-4y^2-3x+6y=\left(x^2-4y^2\right)-\left(3x-6y\right)\)
\(=\left(x-2y\right)\left(x+2y\right)-3\left(x-2y\right)=\left(x-2y\right)\left(x+2y-3\right)\)

7: =(x-4)(x+2)
4: \(=\left(x-2y\right)\left(x+2y\right)-3\left(x-2y\right)\)
\(=\left(x-2y\right)\left(x+2y-3\right)\)

\(1,=5x\left(1-4x+4x^2\right)=5x\left(2x-1\right)^2\\ 2,=x\left(x-2y\right)-3\left(x-2y\right)=\left(x-3\right)\left(x-2y\right)\\ 3,=4x^2-\left(y+3\right)^2=\left(2x+y+3\right)\left(2x-y-3\right)\)

Bài 4:
\(P=\dfrac{4x^2-2x+7}{2x-1}=\dfrac{2x\left(2x-1\right)+7}{2x-1}=2x+\dfrac{7}{2x-1}\in Z\\ \Leftrightarrow2x-1\inƯ\left(7\right)=\left\{-7;-1;1;7\right\}\\ \Leftrightarrow x\in\left\{-3;0;1;4\right\}\\ Q=\dfrac{4x^2-2x+3}{2x-1}=\dfrac{2x\left(2x-1\right)+3}{2x-1}=2x+\dfrac{3}{2x-1}\in Z\\ \Leftrightarrow2x-1\inƯ\left(3\right)=\left\{-3;-1;1;3\right\}\\ \Leftrightarrow x\in\left\{-1;0;1;2\right\}\)
Bài 5:
\(M=\dfrac{\left(5x-1\right)\left(5x+1\right)}{1-5x}+\dfrac{\left(y-3\right)\left(5x+1\right)}{y-3}=-\left(5x+1\right)+5x+1=0\)
Bài 6:
\(VT=\dfrac{a\left(a+3b\right)}{\left(a+3b\right)\left(a-3b\right)}-\dfrac{\left(2a+b\right)\left(a-3b\right)}{\left(a-3b\right)^2}=\dfrac{a}{a-3b}-\dfrac{2a+b}{a-3b}=\dfrac{-a-b}{a-3b}\)
\(VP=\dfrac{\left(a+b\right)\left(a+c\right)}{\left(a+c\right)\left(3b-a\right)}=\dfrac{a+b}{3b-a}=\dfrac{-a-b}{a-3b}\)
Vậy ta đc đpcm

\(1,\\ a,\dfrac{8x}{2xy}=\dfrac{4x}{y}\\ b,\dfrac{2xy}{6y}=\dfrac{x}{3}\\ c,\dfrac{3\left(x+2\right)}{2x}=\dfrac{6\left(x+2\right)}{4x}\\ d,\dfrac{4\left(x-2\right)}{3\left(x+1\right)}=\dfrac{8\left(x-2\right)x}{6\left(x+1\right)x}\\ 2,\\ \dfrac{x^2+3x+2}{x^2+x}=\dfrac{x^2+x+2x+2}{x\left(x+1\right)}=\dfrac{\left(x+1\right)\left(x+2\right)}{x\left(x+1\right)}=\dfrac{x+2}{x}\\ 3,\\ \dfrac{x^2-3x}{x^2-9}=\dfrac{x}{x+3}\)

Bài 3:
Ta có: \(x^2-2x+4=\left(x-1\right)^2+3\ge3\forall x\)
\(\Leftrightarrow P=\dfrac{15}{x^2-2x+4}=\dfrac{15}{\left(x-1\right)^2+3}\le5\forall x\)
Dấu '=' xảy ra khi x=1

\(18,\\ \dfrac{1-9x^2}{x^2+4x}:\dfrac{2-6x}{3x}\left(x\ne0;x\ne-4;x\ne\dfrac{1}{3}\right)\\ =\dfrac{\left(1-3x\right)\left(1+3x\right)}{x\left(x+4\right)}\cdot\dfrac{3x}{2\left(1-3x\right)}=\dfrac{3\left(1+3x\right)}{2\left(x+4\right)}\\ \dfrac{27-x^3}{5x+10}:\dfrac{x-3}{3x+6}\left(x\ne-2;x\ne3\right)=\dfrac{\left(3-x\right)\left(x^2+3x+9\right)}{5\left(x+2\right)}:\dfrac{3\left(x+2\right)}{-\left(3-x\right)}\\ =\dfrac{-3\left(x^2+3x+9\right)}{5}\)
\(19,\\ \dfrac{4x^2}{25y^2}:\dfrac{6x}{5y}:\dfrac{2x}{9y}\left(x,y\ne0\right)=\dfrac{4x^2\cdot5y\cdot9y}{25y^2\cdot6x\cdot2x}=\dfrac{3}{5}\)

1: \(P=\dfrac{2x-5}{x\left(x-1\right)}-\dfrac{x+5}{x}+\dfrac{2x+5}{x-1}\)
\(=\dfrac{2x-5-\left(x+5\right)\left(x-1\right)+x\left(2x+5\right)}{x\left(x-1\right)}\)
\(=\dfrac{2x-5-\left(x^2+4x-5\right)+2x^2+5x}{x\left(x-1\right)}\)
\(=\dfrac{2x^2+7x-5-x^2-4x+5}{x\left(x-1\right)}\)
\(=\dfrac{x^2+3x}{x\left(x-1\right)}=\dfrac{x\left(x+3\right)}{x\left(x-1\right)}=\dfrac{x+3}{x-1}\)
2: \(x^2-1=0\)
=>\(x^2=1\)
=>\(\left[{}\begin{matrix}x=1\left(loại\right)\\x=-1\left(nhận\right)\end{matrix}\right.\)
Thay x=-1 vào P, ta được:
\(P=\dfrac{-1+3}{-1-1}=\dfrac{2}{-2}=-1\)
3: P<1
=>P-1<0
=>\(\dfrac{x+3}{x-1}-1< 0\)
=>\(\dfrac{x+3-x+1}{x-1}< 0\)
=>\(\dfrac{4}{x-1}< 0\)
=>x-1<0
=>x<1
Kết hợp ĐKXĐ, ta được: \(\left\{{}\begin{matrix}x< 1\\x\ne0\end{matrix}\right.\)
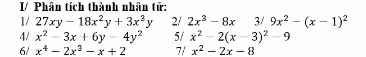



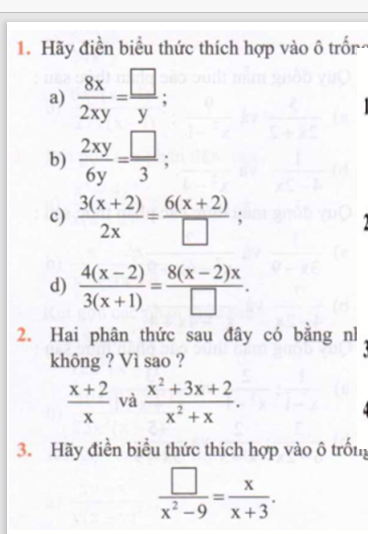 Mọi người giải giúp em với ạ em đang cần gấp ạ
Mọi người giải giúp em với ạ em đang cần gấp ạ Mọi người giải giúp em với ạ em đang cần gấp ạ
Mọi người giải giúp em với ạ em đang cần gấp ạ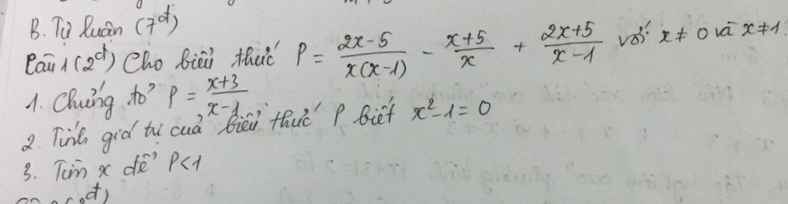 mọi người giúp em với ạ , em đang cần gấp :))
mọi người giúp em với ạ , em đang cần gấp :))
\(\left(x-2\right)^3+6x^2+8=x^3-6x^2+12x-8+6x^2+8=x^3+12x\)