Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Ta có:
(sin α+cos α)^2
=sin^2α + 2sin α cos α + cos^2 α
=1+2sin α cos α
Nên A đúng
(sin α−cos α)^2
=sin^2 α−2sin α cos α+cos^2α
=(sin^2α+cos^2α)−2sin α cos α
=1−2sin α cos α
Nên B đúng
cos^4 α−sin^4 α
=(cos^2 α−sin^2 α)(cos^2 α+sin^2 α)
=(cos^2 α−sin^2 α).1
=cos^2 α−sin^2 α
Nên C đúng
cos^4 α+sin^4 α
=(sin^2 α+cos^2 α )^2−2sin^2 α cos^2 α
=1−2 sin^2 α cos^2 α.
Nên D sai chọn D
ko bít có đúng ko nx
Bạn ơi! Toán từ lớp 10 trở lên bạn vào hoc 24 để gửi câu hỏi nhé!
Bài này câu D sai.
Bạn thay \(\alpha=\frac{\pi}{2}\) vào thử nhé!

Chọn B.
Ta có: 1 + cos2α = 2cos2α và sin2α = 2sinα.cosα.
Mà tanα = 2 nên cot α = 1/2
Suy ra:
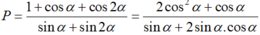
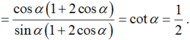

Chọn C.
Ta có :
P = sin(π + α).cos(π - α) = -sin α.(-cos α) = sin α.cos α.
Và ![]() = cos α.(-sin α) = -sin α.cos α.
= cos α.(-sin α) = -sin α.cos α.
Do đó; P + Q = 0.

Chọn D.
Xét biểu thức (sin α - cosα ) 2 + (sin α + cosα ) 2 ta có:
(sin α - cosα ) 2 + (sin α + cosα ) 2
= sin 2 α - 2sin α.cosα + cos 2 α + sin 2 α + 2 sin α.cosα + cos 2 α
= 2( sin 2 α + cos 2 α ) =2
⇒ (sin α - cosα ) 2 = 2 - (sin α + cosα ) 2
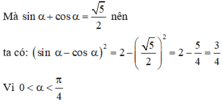
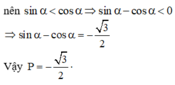

Chọn A.
Áp dụng công thức biến đổi tổng thành tích và công thức nhân đôi; ta có
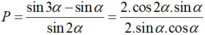

Chọn D
D