Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Có: \(3\overrightarrow{MA}+4\overrightarrow{MB}=0\Leftrightarrow3\overrightarrow{MA}+4\overrightarrow{MB}+3\overrightarrow{MC}=3\overrightarrow{MC}\)
\(\Leftrightarrow3\overrightarrow{MG}+\overrightarrow{MB}=3\overrightarrow{MC}\)
\(\Leftrightarrow3\overrightarrow{MG}+\overrightarrow{MC}+\overrightarrow{CB}=3\overrightarrow{MC}\)
\(\Leftrightarrow3\overrightarrow{MG}+2\overrightarrow{CM}-2\overrightarrow{CN}=0\)
\(\Leftrightarrow3\overrightarrow{MG}+2\overrightarrow{NM}=0\)
Vậy 3 điểm M, N, G thẳng hàng.
b, theo như mình biết thì không có thương hai vec tơ.

Gọi M là trung điểm AB \(\Rightarrow\overrightarrow{CG}=\dfrac{2}{3}\overrightarrow{CM}\)
Mà \(\overrightarrow{CM}=\dfrac{1}{2}\left(\overrightarrow{CA}+\overrightarrow{CB}\right)\) \(\Rightarrow\overrightarrow{CG}=\dfrac{1}{3}\overrightarrow{CA}+\dfrac{1}{3}\overrightarrow{CB}\)
Do I là trung điểm AG:
\(\overrightarrow{CI}=\dfrac{1}{2}\overrightarrow{CG}+\dfrac{1}{2}\overrightarrow{CA}=\dfrac{1}{2}\left(\dfrac{1}{3}\overrightarrow{CA}+\dfrac{1}{3}\overrightarrow{CB}\right)+\dfrac{1}{2}\overrightarrow{CA}=\dfrac{2}{3}\overrightarrow{CA}+\dfrac{1}{6}\overrightarrow{CB}\)
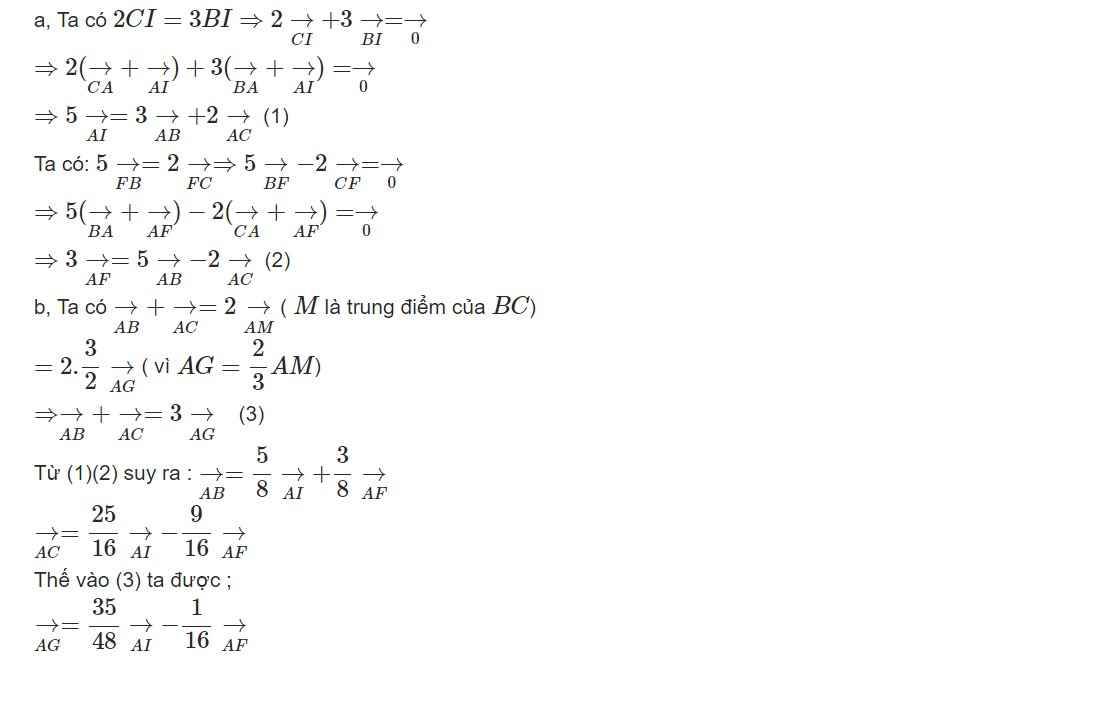
Lời giải:
a) Kéo dài $AG$ cắt $BC$ tại trung điểm $M$. Hiển nhiên $\overrightarrow{BM}, \overrightarrow{CM}$ là vecto đối nên tổng bằng vecto không.
Theo tính chất trọng tâm ta có:
$\overrightarrow{AI}=\frac{1}{2}\overrightarrow{AG}=\frac{1}{2}.\frac{2}{3}\overrightarrow{AM}=\frac{1}{3}\overrightarrow{AM}$
$=\frac{1}{6}(\overrightarrow{AM}+\overrightarrow{AM})=\frac{1}{6}(\overrightarrow{AB}+\overrightarrow{BM}+\overrightarrow{AC}+\overrightarrow{CM})$
$=\frac{1}{6}(\overrightarrow{AB}+\overrightarrow{AC})$
$=\frac{1}{6}(\overrightarrow{AC}+\overrightarrow{CB}+\overrightarrow{AC})$
$=\frac{1}{6}(2\overrightarrow{AC}+\overrightarrow{CB})$
$=\frac{-1}{3}\overrightarrow{CA}+\frac{1}{6}\overrightarrow{CB}$
$=\frac{-1}{3}\overrightarrow{a}+\frac{1}{6}\overrightarrow{b}$
----------------------
$\overrightarrow{AK}=\frac{1}{5}\overrightarrow{AB}=\frac{1}{5}(\overrightarrow{AC}+\overrightarrow{CB})=\frac{1}{5}(-\overrightarrow{CA}+\overrightarrow{CB})$
$=\frac{-1}{5}\overrightarrow{a}+\frac{1}{5}\overrightarrow{b}$
------------------
$\overrightarrow{CI}=\overrightarrow{CA}+\overrightarrow{AI}=\overrightarrow{a}-\frac{1}{3}\overrightarrow{a}+\frac{1}{6}\overrightarrow{b}$
$=\frac{2}{3}\overrightarrow{a}+\frac{1}{6}\overrightarrow{b}$
-------------------
$\overrightarrow{CK}=\overrightarrow{CA}+\overrightarrow{AK}=\overrightarrow{a}-\frac{1}{5}\overrightarrow{a}+\frac{1}{5}\overrightarrow{b}=\frac{4}{5}\overrightarrow{a}+\frac{1}{5}\overrightarrow{b}$
b)
Từ phần a ta thấy: $\overrightarrow{CI}=\frac{5}{6}\overrightarrrow{CK}$ nên $C,I,K$ thẳng hàng.
Hình vẽ:
