

Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



a) Hàm số f(x) = 2x3 + 6x + 1 là hàm đa thức nên liên tục trên R.
Mặt khác vì f(0).f(1) = 1.(-3) < 0 nên phương trình có nghiệm trong khoảng (1; 2).
Vậy phương trình f(x) = 0 có ít nhất hai nghiệm.
b) Hàm số g(x) = cosx – x xác định trên R nên liên tục trên R.
Mặt khác, ta có g(0).g(π/2) = 1. (-π/2) < 0 nên phương trình đã cho có nghiệm trong khoảng (0; π/2).
a) Hàm số f(x) = 2x3 + 6x + 1 là hàm đa thức nên liên tục trên R.
Mặt khác vì f(0).f(1) = 1.(-3) < 0 nên phương trình có nghiệm trong khoảng (1; 2).
Vậy phương trình f(x) = 0 có ít nhất hai nghiệm.
b) Hàm số g(x) = cosx - x xác định trên R nên liên tục trên R.
Mặt khác, ta có g(0).g() = 1. (-
) < 0 nên phương trình đã cho có nghiệm trong khoảng (0;
).

Chứng minh rằng phương trình sau có ít nhất hai nghiệm :
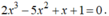
- Xét hàm số: f ( x ) = 2 x 3 - 5 x 2 + x + 1 là hàm đa thức.
⇒ Hàm số f liên tục trên R.
- Ta có:
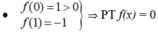 có ít nhất một nghiệm c1 ∈ (0;1).
có ít nhất một nghiệm c1 ∈ (0;1).
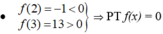 có ít nhất một nghiệm c2 ∈ (2;3).
có ít nhất một nghiệm c2 ∈ (2;3).
- Mà c ≠ c 2 nên PT f(x) = 0 có ít nhất 2 nghiệm.

gợi ý: a)chia 2 vế cho căn 5
đặt \(\frac{1}{\sqrt{5}}=cosa\Rightarrow\frac{2}{\sqrt{5}}=sina\)
khi đó pt <=>sin(x-a)=\(\frac{3}{\sqrt{5}}>1\)
->vô nghiệm
bn giải thích cho mk chỗ này được ko : \(\frac{1}{\sqrt{5}}=\cos a\Rightarrow\frac{2}{\sqrt{5}}=\sin a\)

Đặt f(x) = x5 – 3x4 + 5x – 2, ta có:
⎧⎪ ⎪ ⎪ ⎪ ⎪ ⎪ ⎪ ⎪⎨⎪ ⎪ ⎪ ⎪ ⎪ ⎪ ⎪ ⎪⎩f(−2)=(−2)5−3(−2)4+5(−2)−2<0f(0)=−2<0f(1)=1−3+5−2=1>0f(2)=25−3.24+5.2−2=−8<0f(2)=35−3.34+5.3−2=13<0⇒⎧⎪⎨⎪⎩f(0).f(1)<0(1)f(1).f(2)<0(2)f(2).f(3)<0(3){f(−2)=(−2)5−3(−2)4+5(−2)−2<0f(0)=−2<0f(1)=1−3+5−2=1>0f(2)=25−3.24+5.2−2=−8<0f(2)=35−3.34+5.3−2=13<0⇒{f(0).f(1)<0(1)f(1).f(2)<0(2)f(2).f(3)<0(3)
_ Hàm số f(x) là hàm số đa thức liên tục trên R.
⇒ Hàm số f(x) liên tục trên các đoạn [0, 1], [1, 2], [2, 3] (4)
Từ (1), (2), (3) và (4) ⇒ phương trình x5 – 3x4 + 5x – 2 = 0 có ít nhất một nghiệm trên mỗi khoảng (0, 1), (1, 2), (2, 3).
Vậy phương trình x5 – 3x4 + 5x – 2 = 0 có ít nhất ba nghiệm trên khoảng (-2, 5) (đpcm)
Đặt \(f\left(x\right)=x^5-3x^4+5x-2\).
\(f\left(-2\right)=\left(-2\right)^5-3.\left(-2\right)^4+5.\left(-2\right)-2=-56< 0\).
\(f\left(0\right)=-2< 0\).
\(f\left(1\right)=1^5-3.1^4+5.1-2=1>0\).
\(f\left(2\right)=2^5-3.2^4+5.2-2=-8< 0\).
\(f\left(3\right)=3^5-3.3^4+5.3-2=13>0\).
\(\Rightarrow\left\{{}\begin{matrix}f\left(0\right).f\left(1\right)< 0\\f\left(1\right).f\left(2\right)< 0\\f\left(2\right).f\left(3\right)< 0\end{matrix}\right.\).
Hàm số đã cho là hàm đa thức nên liên tục trên R.
Suy ra hàm số liên tục trên các đoạn: \(\left[0;1\right];\left[1;2\right];\left[2;3\right]\) nên phương trình \(x^5-3x^4+5x-2=0\) có ít nhất một nghiệm trên các khoảng \(\left(0;1\right);\left(1;2\right);\left(2;3\right)\).

Đặt \(f\left(x\right)=sin^3x+sinx-1\)
Hàm \(f\left(x\right)\) liên tục trên R
Ta có: \(f\left(0\right)=-1\) ; \(f\left(\dfrac{\pi}{2}\right)=1\)
\(\Rightarrow f\left(0\right).f\left(\dfrac{\pi}{2}\right)< 0\)
\(\Rightarrow\) Phương trình \(f\left(x\right)=0\) luôn có ít nhất 1 nghiệm thuộc \(\left(0;\dfrac{\pi}{2}\right)\) (đpcm)