
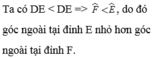
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

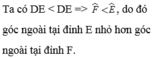

\(\Delta DEF\) cho ta \(\widehat{D}+\widehat{E}+\widehat{F}=180^0\)
\(\Rightarrow\widehat{D}=180^0-\left(\widehat{E}+\widehat{F}\right)\)
\(\Rightarrow\widehat{D}=180^0-\left(70^0+60^0\right)=180^0-130^0=50^0\)
\(Xét\) \(\Delta ABCvà\Delta DEFcó\)
\(\widehat{A}=\widehat{D}\left(=50^0\right)\)
AB=DE
AC=DF
\(\Rightarrow\Delta ABC=\Delta DEF\left(c-g-c\right)\)
Vậy \(\Delta ABC=\Delta DEF\)

a: góc MDH=90 độ-góc DMH
=90 độ-2*góc MDF
=90 độ-2*góc E
=góc F+góc E-2*góc E
=góc F-gócE
b: (EF+DH)^2-(DF+DE)^2
=EF^2+2*EF*DH+DH^2-DF^2-DE^2-2*DF*DE
=DH^2>0
=>EF+DH>DF+DE
=>EF-DE>DF-DH

a) Có DE < DF( 5cm < 12cm)
->góc F< góc E
b) áp dụng đl pytago:
EF^2=DE^2+DF^2=5^2+12^2=169
= > EF=13 (cm)
tam giác DEF có DM là trung tuyến(M là trung điểm của EF) ứng với cạnh huyền
=> DM=EM=MF=EF/2=13/2=6,5cm

1.
Ta có:
\(\widehat{A}+\widehat{B}+\widehat{C}=180^0\)
Mà \(\widehat{B}=\widehat{C}\)
\(\Rightarrow\widehat{A}+\widehat{C}+\widehat{C}=180^0\)
\(\widehat{A}=180^0-2.65^0\)
\(\widehat{A}=50^0\)
2.
Áp dụng định lý pitago, ta có:
\(DF^2=DE^2+EF^2\)
\(\Rightarrow EF=\sqrt{DF^2-DE^2}=\sqrt{17^2-8^2}=\sqrt{225}=15cm\)
Ta có:
\(DF>EF>DE\)
\(\Rightarrow\widehat{E}>\widehat{D}>\widehat{F}\)

a:
\(\widehat{HDE}+\widehat{E}=90^0\)(ΔHDE vuông tại H)
\(\widehat{E}+\widehat{F}=90^0\)(ΔEDF vuông tại D)
Do đó: \(\widehat{HDE}=\widehat{F}\)
ΔDEF vuông tại D
mà DM là đường trung tuyến
nên MD=MF
=>\(\widehat{MDF}=\widehat{MFD}=\widehat{F}\)
\(\widehat{EDH}+\widehat{MDH}+\widehat{FDM}=\widehat{EDF}=90^0\)
=>\(\widehat{F}+\widehat{MDH}+\widehat{F}=90^0\)
=>\(\widehat{MDH}+2\cdot\widehat{F}=\widehat{E}+\widehat{F}\)
=>\(\widehat{MDH}=\widehat{E}+\widehat{F}-2\cdot\widehat{F}=\widehat{E}-\widehat{F}\)
b:
Xét ΔDEF vuông tại D có DH là đường cao
nên \(DE\cdot DF=DH\cdot EF\)
ΔDEF vuông tại D
=>\(DE^2+DF^2=EF^2\)
\(\left(EF+DH\right)^2=EF^2+2\cdot EF\cdot DH+DH^2\)
\(=EF^2+2\cdot DE\cdot DF+DH^2\)
\(\left(DF+DE\right)^2=DF^2+2\cdot DF\cdot DE+DE^2\)
\(=\left(DF^2+DE^2\right)+2\cdot DF\cdot DE\)
\(=EF^2+2\cdot DH\cdot EF\)
\(\left(EF+DH\right)^2-\left(DF+DE\right)^2\)
\(=EF^2+2\cdot DH\cdot EF+DH^2-EF^2-2\cdot DH\cdot EF\)
\(=DH^2>0\)
=>EF+DH>DF+DE
=>EF-DE>DF-DH