Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a. Pt(1) có 2 nghiệm phân biệt \(\Leftrightarrow\Delta=4\left(m-1\right)^2-4.m^2=4\left(m^2-2m+1\right)-4m^2=-8m+4>0\)
\(\Rightarrow m< \frac{1}{2}\)
b. Theo hệ thức Viet ta có \(\hept{\begin{cases}x_1+x_2=2\left(m-1\right)\\x_1.x_2=m^2\end{cases}}\)
Từ \(x_1^2+x_2^2-3.x_1.x_2+3=0\Rightarrow\left(x_1+x_2\right)^2-5.x_1.x_2+3=0\)
\(\Rightarrow4\left(m^2-2m+1\right)-5m^2+3=0\Rightarrow-m^2-8m+7=0\)
\(\Rightarrow\orbr{\begin{cases}m=-4-\sqrt{23}\\m=-4+\sqrt{23}\left(l\right)\end{cases}}\)
Vậy \(m=-4-\sqrt{23}\)

\(\left(m+1\right)x^2-2\left(m-1\right)x+m-3=0\) (1)
a) Phương trình (1) có 2 nghiệm phân biệt khi và chỉ khi:
\(\Delta'=\left(m-1\right)^2-\left(m+1\right)\left(m-3\right)>0\)
\(\Leftrightarrow\left(m^2-2m+1\right)-\left(m^2-2m-3\right)>0\)
\(\Leftrightarrow4>0\)(luôn đúng)
Vậy phương trình có 2 nghiệm phân biệt với mọi m.
b) Để t nghĩ tí

a) pt có 2 nghiệm dương <=> \(\Delta\ge0;\int^{x1+x2>0}_{x1.x2>0}\Leftrightarrow4\left(m+1\right)^2-4\left(m-4\right)\ge0;\int^{2m+2>0}_{m-4>0}\Leftrightarrow4m^2+4m+4+16\ge0;\int^{m>-1}_{m>4}\)
=> m>4. (cái kí hiệu ngoặc kia là kí hiệu và nha. tại trên này không có nên dùng tạm cái ý)
b) áp dụng hệ thức vi ét ta có: x1+x2=2m+2; x1.x2=m-4
\(M=\frac{\left(x1+x2\right)^2-2x1x2}{x1-x1.x2+x2-x1.x2}=\frac{\left(2m+2\right)^2-2\left(m-4\right)}{2m+2-2\left(m-4\right)}=\frac{4m^2+6m+12}{10}=\frac{\left(4m^2+6m+\frac{9}{4}\right)+\frac{39}{4}}{10}=\frac{\left(2m+\frac{3}{2}\right)^2+\frac{39}{4}}{10}\)
ta có: \(\left(2m+\frac{3}{2}\right)^2\ge0\Leftrightarrow\left(2m+\frac{3}{2}\right)^2+\frac{39}{4}\ge\frac{39}{4}\Leftrightarrow\frac{\left(2m+\frac{3}{2}\right)^2+\frac{39}{4}}{10}\ge\frac{39}{40}\)=> Min M=39/40 <=>m=-3/4

\(a,\Delta=m^2-4m+4=\left(m-2\right)^2\ge0\forall m\)
Nên pt đã cho luôn có 2 nghiệm phân biệt với mọi m
b, Theo Vi-ét \(\hept{\begin{cases}x_1+x_2=m\\x_1x_2=m-1\end{cases}}\)
Ta có \(B=\frac{2x_1x_2+3}{x_1^2+x_2^2+2\left(1+x_1x_2\right)}=1\)
\(\Leftrightarrow\frac{2x_1x_2+3}{\left(x_1+x_2\right)^2+2}=1\)
\(\Leftrightarrow\frac{2\left(m-1\right)+3}{m^2+2}=1\)
\(\Leftrightarrow\frac{2m+1}{m^2+2}=1\)
\(\Leftrightarrow2m+1=m^2+2\)
\(\Leftrightarrow m^2-2m+1=0\)
\(\Leftrightarrow\left(m-1\right)^2=0\)
\(\Leftrightarrow m=1\)

Để PT có 2 nghiệm thì:
∆' = (m - 1)2 - (m - 5) > 0
<=> m2 - 3m + 6 > 0
Đúng với mọi m.
Theo vi et ta có: \(\hept{\begin{cases}x_1+x_2=2m-2\\x_1.x_2=m-5\end{cases}}\)
Theo đề ta có:
(2x1 - 1)(2x2 - 1) = 3
<=> 4x1x2 - 2(x1 + x2) = 2
<=> 4(m - 5) - 2(2m - 2) = 2
<=> 0m = 18
Vậy không tồn tại n thỏa mãn

\(A=\left(\frac{x_1}{x_2}+\frac{x_2}{x_1}\right)^2-2=\left[\frac{x_1^2+x^2_2}{x_1x_2}\right]^2-2=\left[\frac{\left(x_1+x_2\right)^2-2x_1x_2}{x_1x_2}\right]^2-2\)
\(=\left[\frac{\left(2m-2\right)^2}{2m-5}-2\right]^2-2\)\(=\left(\frac{4m^2-8m+4}{2m-5}-2\right)^2-2=\left(2m-1+\frac{9}{2m-5}\right)^2-2\)
A nguyên khi \(\left(2m-1+\frac{9}{2m-5}\right)^2\in Z\)
\(\Leftrightarrow B=2m-1+\frac{9}{2m-5}=\frac{8m^2-12m+14}{2m-5}\)\(=\sqrt{k}\) với k là một số nguyên dương.
\(\Rightarrow8m^2-12m+14=\sqrt{k}\left(2m-5\right)\)\(\Leftrightarrow8m^2-2\left(6+\sqrt{k}\right)m+14+5\sqrt{k}=0\text{ (1)}\)
(1) có nghiệm m khi \(\Delta'=\left(\sqrt{k}+6\right)^2-8\left(14+5\sqrt{k}\right)\ge0\)
\(\Leftrightarrow k-28\sqrt{k}-76\ge0\Leftrightarrow\sqrt{k}\le14-4\sqrt{17}<0\text{ (loại) hoặc }\sqrt{k}\ge14+4\sqrt{17}\)
\(\Leftrightarrow k\ge\left(14+4\sqrt{17}\right)^2\approx929,78\Rightarrow k\ge930\)
Vậy \(m=\frac{6+\sqrt{k}+\sqrt{k-28\sqrt{k}-76}}{8}\text{ hoặc }m=\frac{6+\sqrt{k}-\sqrt{k-28\sqrt{k}-76}}{8}\) với k là một số nguyên lớn hợn hoặc bằng 930.
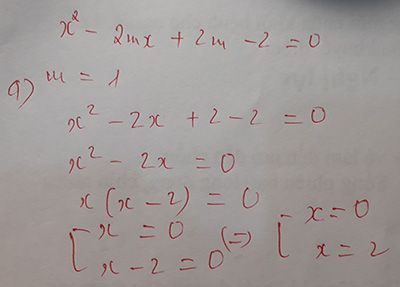
Câu 2:
\(\Delta'=\left(m-1\right)^2-m+3=m^2-3m+4=\left(m-\frac{3}{2}\right)^2+\frac{7}{4}>0;\forall m\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m-1\right)\\x_1x_2=m-3\end{matrix}\right.\)
\(P=x_1^2+x_2^2=\left(x_1+x_2\right)^2-2x_1x_2\)
\(=4\left(m-1\right)^2-2\left(m-3\right)\)
\(=4m^2-10m+10=4\left(m-\frac{5}{4}\right)^2+\frac{15}{4}\ge\frac{15}{4}\)
\(\Rightarrow P_{min}=\frac{15}{4}\) khi \(m=\frac{5}{4}\)
Câu 1:
Để pt có 2 nghiệm \(\left\{{}\begin{matrix}m\ne0\\\Delta'=\left(m-2\right)^2-m\left(m-3\right)\ge0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m\ne0\\-m+4\ge0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}m\ne0\\m\le4\end{matrix}\right.\)
Theo Viet: \(\left\{{}\begin{matrix}x_1+x_2=-\frac{2\left(m-2\right)}{m}\\x_1x_2=\frac{m-3}{m}\end{matrix}\right.\)
\(A=x_1^2+x_2^2=\left(x_1+x_2\right)^2-2x_1x_2\)
\(=\frac{4\left(m-2\right)^2}{m^2}-\frac{2\left(m-3\right)}{m}=\frac{4m^2-8m+4}{m^2}-\frac{2m-6}{m}\)
\(=4-\frac{8}{m}+\frac{4}{m^2}-2+\frac{6}{m}=\frac{4}{m^2}-\frac{2}{m}+2\)
\(=4\left(\frac{1}{m}-\frac{1}{4}\right)^2+\frac{7}{4}\ge\frac{7}{4}\)
\(A_{min}=\frac{7}{4}\) khi \(\frac{1}{m}=\frac{1}{4}\Leftrightarrow m=4\)