Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Lời giải:
Để pt có 2 nghiê pb thì:
$\Delta'=1-(m-3)>0\Leftrightarrow m< 4$
Áp dụng định lý Viet: \(\left\{\begin{matrix} x_1+x_2=2\\ x_1x_2=m-3\end{matrix}\right.\)
Khi đó:
\(x_1^2-2x_2+x_1x_2=-12\)
\(\Leftrightarrow x_1^2-2(2-x_1)+x_1(2-x_1)=-12\)
\(\Leftrightarrow x_1=-2\Leftrightarrow x_2=2-x_1=4\)
$m-3=x_1x_2=(-2).4=-8$
$\Leftrightarrow m=-5$ (tm)

\(x^2-x+1-m=0\left(1\right)\\ \text{PT có 2 nghiệm }x_1,x_2\\ \Leftrightarrow\Delta=1-4\left(1-m\right)\ge0\\ \Leftrightarrow4m-3\ge0\Leftrightarrow m\ge\dfrac{3}{4}\\ \text{Vi-ét: }\left\{{}\begin{matrix}x_1+x_2=1\\x_1x_2=1-m\end{matrix}\right.\\ \text{Ta có }5\left(\dfrac{1}{x_1}+\dfrac{1}{x_2}\right)-x_1x_2+4=0\\ \Leftrightarrow5\cdot\dfrac{x_1+x_2}{x_1x_2}-x_1x_2+4=0\\ \Leftrightarrow\dfrac{5}{1-m}+m-1+4=0\\ \Leftrightarrow\dfrac{5}{1-m}+m+3=0\\ \Leftrightarrow5+\left(1-m\right)\left(m+3\right)=0\\ \Leftrightarrow m^2+2m-8=0\\ \Leftrightarrow m^2-2m+4m-8=0\\ \Leftrightarrow\left(m-2\right)\left(m+4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}m=2\left(n\right)\\m=-4\left(l\right)\end{matrix}\right.\)
Vậy $m=2$

Mình nghĩ với pt tổng quát: \(ax^2+bx+c=0\) có \(\Delta=b^2-4ac\)
Nếu như vậy thì: \(1.x^2+6x+m\) có \(\Delta=6^2-4m\)chứ?
Riêng mình thì bài này mình dùng delta phẩy cho lẹ:
Lời giải
Để pt \(x^2+6x+m=0\) có 2 nghiệm phân biệt thì:
\(\Delta'=\left(\frac{b}{2}\right)^2-ac=3^2-m>0\)
\(\Leftrightarrow m< 9\)

a: Δ=(-2m)^2-4(m-2)
=4m^2-4m+8=(2m-1)^2+7>=7>0
=>PT luôn có hai nghiệm phân biệt
b: x1^2+x2^2-6x1x2
=(x1+x2)^2-8x1x2
=(2m)^2-8(m-2)
=4m^2-8m+16=(2m-2)^2+8>=8
=>24/(2m-2)^2+8<=3
=>M>=-3
Dấu = xảy ra khi m=1

\(a,\Delta'=\left(-1\right)^2-\left(m-3\right)=1-m+3=4-m\)
Để pt trên có nghiệm thì \(4-m\ge0\Leftrightarrow m\le4\)
b, Theo Vi-ét:\(\left\{{}\begin{matrix}x_1+x_2=2\\x_1x_2=m-3\end{matrix}\right.\)
\(\left(x_1+x_2\right)^2=16+2x_1x_2\\ \Leftrightarrow2^2=16+2\left(m-3\right)\\ \Leftrightarrow2m-6+16-4=0\\ \Leftrightarrow2m+6=0\\ \Leftrightarrow m=-3\left(tm\right)\)

Ta có: \(\Delta'=2m^2+4>0\forall m\)
Theo Vi-ét, ta có: \(\left\{{}\begin{matrix}x_1+x_2=2m\\x_1x_2=-m^2-4\end{matrix}\right.\)
Mặt khác: \(x_1^2+x_2^2=20\)
\(\Rightarrow\left(x_1+x_2\right)^2-2x_1x_2=20\)
\(\Rightarrow4m^2+2m^2-12=0\) \(\Leftrightarrow\left[{}\begin{matrix}m=-2\\m=\dfrac{3}{2}\end{matrix}\right.\)
Vậy ...

Xét \(\Delta=\text{}\)\(\left(-4m\right)^2-4\left(3m^2-3\right)\)\(=4m^2+12>0\forall m\)
=> Pt luôn có hai nghiệm pb
Theo viet \(\left\{{}\begin{matrix}x_1+x_2=4m\\x_1x_2=3m^2-3\end{matrix}\right.\)
\(P=\dfrac{2019}{\left|x_1-x_2\right|}\)\(\Leftrightarrow P^2=\dfrac{2019^2}{\left(x_1-x_2\right)^2}\)\(=\dfrac{2019^2}{\left(x_1+x_2\right)^2-4x_1x_2}\)\(=\dfrac{2019^2}{16m^2-4\left(3m^2-3\right)}\)
\(=\dfrac{2019^2}{4m^2+12}\le\dfrac{2019^2}{12}\)
\(\Rightarrow P\le\dfrac{2019}{\sqrt{12}}\)
\(\Rightarrow P_{max}=\dfrac{2019\sqrt{12}}{12}\Leftrightarrow m=0\)
Vậy m=0

Làm câu b)
Để phương trình có hai nghiệm phân biệt:
\(\Delta'\ge0\Leftrightarrow3^2-\left(m+1\right)\ge0\Leftrightarrow m\le8\)
Áp dụng định lí Vi-ét ta có:
\(\hept{\begin{cases}x_1+x_2=6\\x_1.x_2=m+1\end{cases}}\)(1)
Xét: \(x^2_1+x^2_2=3\left(x_1+x_2\right)\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=3\left(x_1+x_2\right)\)(2)
Từ 1, 2 ta có:
\(6^2-2\left(m+1\right)=3.6\Leftrightarrow m=8\)(tm)
Vậy ...
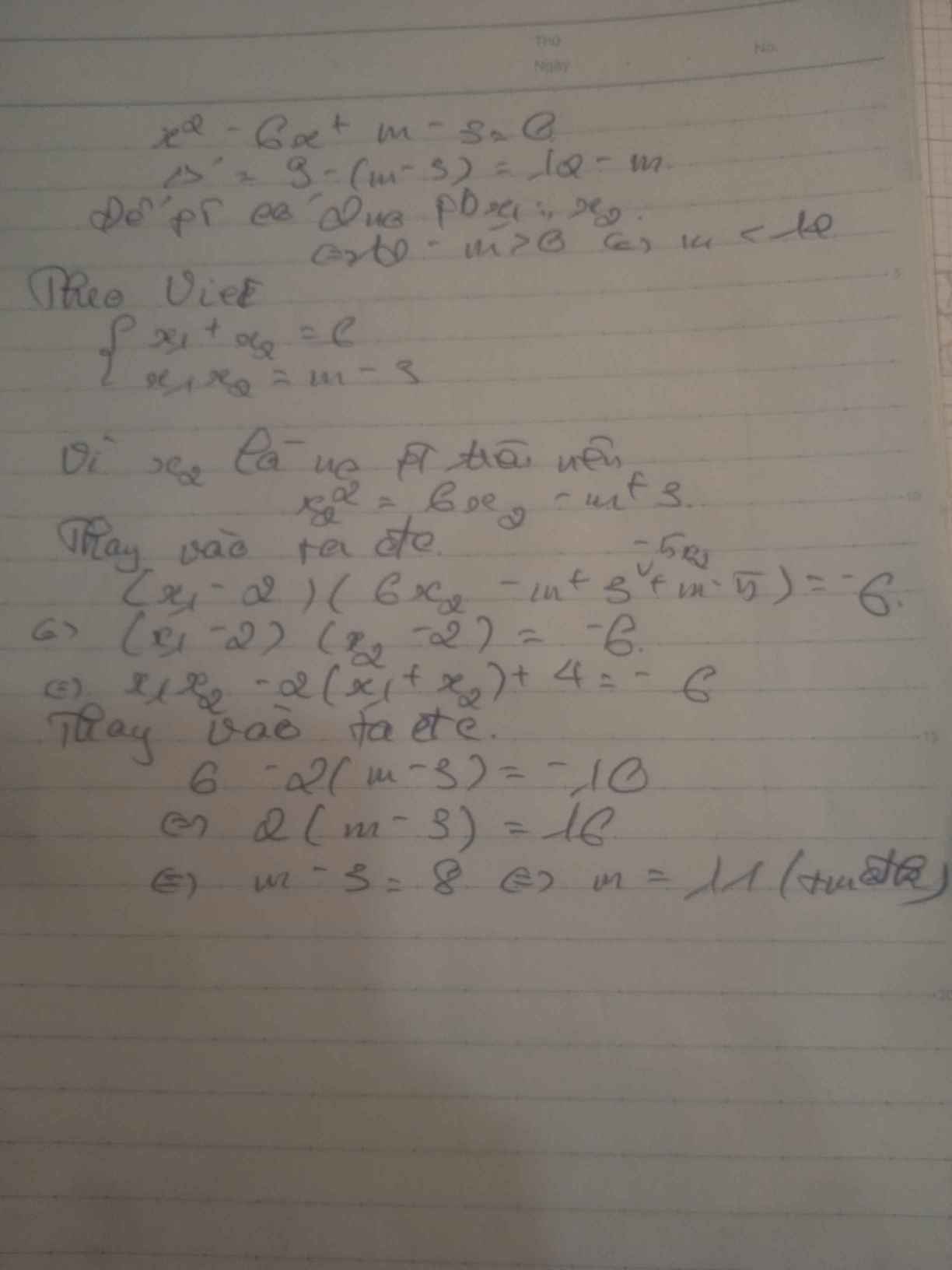
\(\text{Δ}=\left(-6\right)^2-4\cdot1\cdot\left(-m\right)=4m+36\)
Để phương trình có hai nghiệm phân biệt thì Δ>0
=>4m+36>0
=>m>-9
Theo Vi-et, ta có:
\(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=6\\x_1x_2=\dfrac{c}{a}=-m\end{matrix}\right.\)
\(\left(x_1-x_2\right)^2=\left(x_1+x_2\right)^2-4x_1x_2=36+4m\)
=>\(x_1-x_2=\pm2\sqrt{m+9}\)
\(x_1^2-x_2^2=24\)
=>\(\left(x_1+x_2\right)\left(x_1-x_2\right)=24\)
=>\(\pm2\sqrt{m+9}=4\)
=>\(\pm\sqrt{m+9}=2\)
=>\(\left[{}\begin{matrix}\sqrt{m+9}=2\left(nhận\right)\\\sqrt{m+9}=-2\left(loại\right)\end{matrix}\right.\Leftrightarrow m+9=4\)
=>m=-5(nhận)