Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Phương trình 2 nghiệm phân biệt khi
\(\Delta=\left(1-m\right)^2-4\left(-m\right).1=\left(m+1\right)^2>0\)
\(\Leftrightarrow m\ne-1\)
Hệ thức Vière : \(\hept{\begin{cases}x_1+x_2=m-1\\x_1.x_2=-m\end{cases}}\)
Khi đó \(x_1\left(5-x_2\right)\ge5\left(3-x_2\right)-36\)
<=> \(-x_1x_2+5\left(x_1+x_2\right)\ge-21\)
<=> \(-\left(-m\right)+5\left(m-1\right)\ge-21\)
\(\Leftrightarrow6m\ge-16\Leftrightarrow m\ge-\frac{8}{3}\)
Kết hợp điều kiện => \(\hept{\begin{cases}m\ge-\frac{8}{3}\\m\ne-1\end{cases}}\)thì thỏa mãn bài toán
\(\Delta=\left(1-m\right)^2+4m=\left(m+1\right)^2>0\Rightarrow m\ne-1\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=m-1\\x_1x_2=-m\end{matrix}\right.\)
\(x_1\left(5-x_2\right)\ge5\left(3-x_2\right)-36\)
\(\Leftrightarrow5\left(x_1+x_2\right)-x_1x_2\ge-21\)
\(\Leftrightarrow5\left(m-1\right)+m\ge-21\)
\(\Leftrightarrow m\ge-\dfrac{8}{3}\)
Kết hợp điều kiện ban đầu ta được: \(\left\{{}\begin{matrix}m\ne-1\\m\ge-\dfrac{8}{3}\end{matrix}\right.\)

Đặt \(t=\sqrt{x}\left(t\ge0\right)\Rightarrow t^2-\sqrt{6}t-3+2m=0\left(1\right)\)
Giả sử phương trình $(1)$ có nghiệm $t_1;t_2$ thì \(t_1+t_2=\sqrt{6}\) và \(t_1.t_2=2m-3\)
\(t_1=\sqrt{x_1}\left(t_1\ge0\right)\Rightarrow x_1=t_1^2\) và \(t_2=\sqrt{x_2}\left(t_2\ge0\right)\Rightarrow x_2=t_2^2\)
Ta có: \(\dfrac{{{x_1} + {x_2}}}{{\sqrt {{x_1}} + \sqrt {{x_2}} }} = \dfrac{{\sqrt {24} }}{3}\)
\(\Leftrightarrow \dfrac{{t_1^2 + t_2^2}}{{{t_1} + {t_2}}} = \dfrac{{\sqrt {24} }}{3}\\ \Leftrightarrow \dfrac{{{{\left( {{t_1} + {t_2}} \right)}^2} - 2{t_1}{t_2}}}{{{t_1} + {t_2}}} = \dfrac{{\sqrt {24} }}{3}\\ \Leftrightarrow \dfrac{{6 + 6 - 4m}}{{\sqrt 6 }} = \dfrac{{\sqrt {24} }}{3} \Leftrightarrow m = 2\left( {tm} \right)\)

pt có 2 nghiệm pb dương
<=> {delta=25-4m>0
{ x1+x2=5>0
{x1..x2=m>0
<=> 0<m <25/4
( x1canx2+x2canx1)2=36
x1^2..x2 +x1 ..x2^2 +2 (x1×x2)can (x1×x2)=36
sau đó sử ddụng viet và thay vào
mn cho mk hỏi
nếu đđặt câu hỏi trên OLM này thì khi có người giải đáp cho mk thì có thông báo k z
Lập \(\Delta=25-4m\)
Phương trình có 2 nghiệm \(x_1;x_2\)khi \(\Delta\ge0\)hay \(m\le\frac{25}{4}\)
Áp dụng hệ thức Vi-et ta có \(\hept{\begin{cases}x_1+x_2=5\\x_1x_2=m\end{cases}}\)
2 nghiệm \(x_1;x_2\)dương khi \(\hept{\begin{cases}x_1+x_2>0\\x_1x_2>0\end{cases}}\)hay m>0
Điều kiện để pt có 2 nghiệm dương x1;x2 là \(0< m< \frac{25}{4}\)(*)
Ta có \(\left(\sqrt{x_1}+\sqrt{x_2}\right)^2=x_1+x_2+2\sqrt{x_1x_2}=5+2\sqrt{m}\)
=> \(\sqrt{x_1}+\sqrt{x_2}=\sqrt{5+2\sqrt{m}}\)
Ta có \(x_1\sqrt{x_2}+x_2\sqrt{x_1}=6\Leftrightarrow\sqrt{x_1x_2}\left(\sqrt{x_1}+\sqrt{x_2}\right)=6\)
hay \(\sqrt{m}\sqrt{5+2\sqrt{m}}=6\Leftrightarrow2m\sqrt{m}+5m-36=0\left(1\right)\)
Đặt \(t=\sqrt{m}\ge0\)khi đó (1) trở thành
\(\Leftrightarrow2t^2+5t^2-36=0\)
\(\Leftrightarrow\left(t-2\right)\left(2t^2+9t+18\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}t-2=0\\2t^2+9t+18=0\end{cases}\Rightarrow t=2\Rightarrow m=4\left(tmđk\right)}\)
(vì 2t2+9t+18 vô nghiệm)
Vậy m=4 thì pt đã cho có 2 nghiệm dương x1;x2 thỏa mãn \(x_1\sqrt{x_2}+x_2\sqrt{x_1}=6\)

Do \(x_1x_2=-\frac{2019}{2017}< 0\Rightarrow\) pt có 2 nghiệm trái dấu.
\(\sqrt{x_1^2+2018}-x_2=\sqrt{x_2^2+2018}+x_1\)
\(\Rightarrow x_1^2+x_2^2+2018-2x_2\sqrt{x^2_1+2018}=x_1^2+x_2^2+2018+2x_1\sqrt{x_2^2+2018}\)
\(\Leftrightarrow-x_2\sqrt{x_1^2+2018}=x_1\sqrt{x_2^2+2018}\)
\(\Rightarrow x_2^2\left(x_1^2+2018\right)=x_1^2\left(x_2^2+2018\right)\)
\(\Rightarrow x_1^2=x_2^2\Rightarrow x_1=-x_2\) (do \(x_1;x_2\) trái dấu)
\(\Rightarrow x_1+x_2=0\Rightarrow\frac{m-2018}{2017}=0\Rightarrow m=2018\)
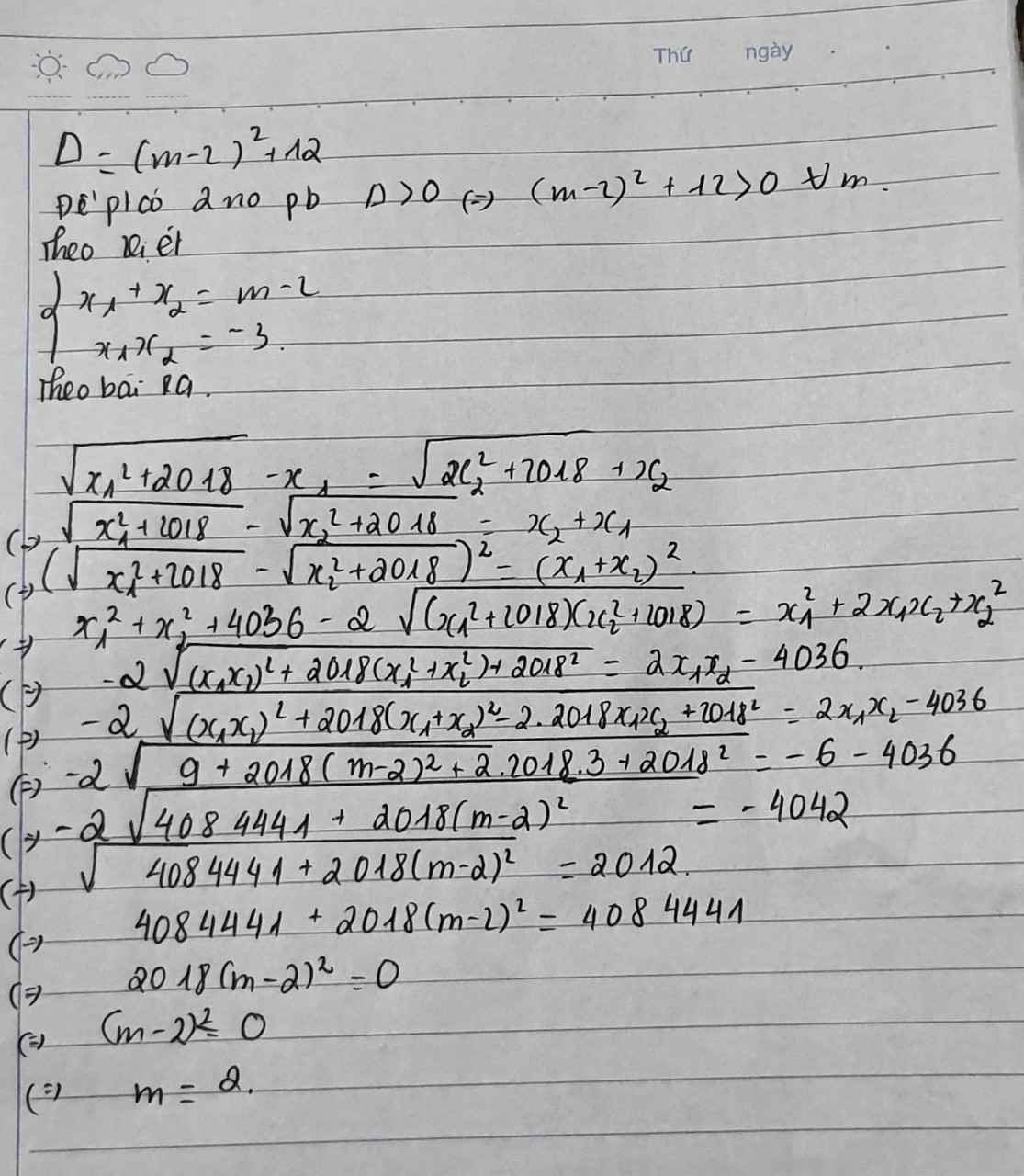
\(\Delta'=\left(m+1\right)^2-\left(2m-3\right)=m^2+4>0;\forall m\)
Để pt có 2 nghiệm không âm: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m+1\right)>0\\x_1x_2=2m-3\ge0\end{matrix}\right.\) \(\Rightarrow m\ge\frac{3}{2}\)
Theo Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m+1\right)\\x_1x_2=2m-3\end{matrix}\right.\)
\(\sqrt{x_1}+\sqrt{x_2}=3\Leftrightarrow x_1+x_2+2\sqrt{x_1x_2}=9\)
\(\Leftrightarrow2\left(m+1\right)+2\sqrt{2m-3}=9\)
\(\Leftrightarrow2m-3+2\sqrt{2m-3}-4=0\)
Đặt \(\sqrt{2m-3}=t\ge0\)
\(\Rightarrow t^2+2t-4=0\Rightarrow\left[{}\begin{matrix}t=\sqrt{5}-1\\t=-\sqrt{5}-1\left(l\right)\end{matrix}\right.\)
\(\Rightarrow\sqrt{2m-3}=\sqrt{5}-1\)
\(\Leftrightarrow2m-3=6-2\sqrt{5}\Rightarrow m=\frac{9-2\sqrt{5}}{2}\)