Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(a,\Delta=m^2-4m+4=\left(m-2\right)^2\ge0\forall m\)
Nên pt đã cho luôn có 2 nghiệm phân biệt với mọi m
b, Theo Vi-ét \(\hept{\begin{cases}x_1+x_2=m\\x_1x_2=m-1\end{cases}}\)
Ta có \(B=\frac{2x_1x_2+3}{x_1^2+x_2^2+2\left(1+x_1x_2\right)}=1\)
\(\Leftrightarrow\frac{2x_1x_2+3}{\left(x_1+x_2\right)^2+2}=1\)
\(\Leftrightarrow\frac{2\left(m-1\right)+3}{m^2+2}=1\)
\(\Leftrightarrow\frac{2m+1}{m^2+2}=1\)
\(\Leftrightarrow2m+1=m^2+2\)
\(\Leftrightarrow m^2-2m+1=0\)
\(\Leftrightarrow\left(m-1\right)^2=0\)
\(\Leftrightarrow m=1\)

\(x^2-4x-m^2=0\) (1)
\(a)\) Để pt (1) có hai nghiệm phân biệt \(x_1,x_2\) thì \(\Delta'=\left(-2\right)^2-\left(-m\right)^2=4+m^2>0\) ( luôn đúng )
Vậy pt (1) luôn có hai nghiệm phân biệt \(x_1,x_2\) với mọi m
\(b)\) Ta có : \(A=\left|x_1^2-x_2^2\right|=\left|\left(x_1+x_2\right)\left(x_1-x_2\right)\right|\)
\(\Leftrightarrow\)\(A^2=\left(x_1+x_2\right)^2\left(x_1-x_2\right)^2=\left(x_1+x_2\right)^2\left(x_1^2+x_2^2-2x_1x_2\right)=\left(x_1+x_2\right)^2\left[\left(x_1+x_2\right)^2-4x_1x_2\right]\) (*)
Theo định lý Vi-et ta có : \(\hept{\begin{cases}x_1+x_2=4\\x_1x_2=-m^2\end{cases}}\)
(*) \(\Leftrightarrow\)\(A^2=4^2\left[4^2-4\left(-m^2\right)\right]=16\left(16+4m^2\right)=64m^2+256\ge256\)
\(\Leftrightarrow\)\(A\ge\sqrt{256}=16\)
Dấu "=" xảy ra \(\Leftrightarrow\)\(64m^2=0\)\(\Leftrightarrow\)\(m=0\)
Vậy GTNN của \(A=16\) khi \(m=0\)

a,\(x^2-4x-m^2=0\)(*)
\(\Delta=4^2-4\left(-m^2\right)=16+4m^2\ge16>0\)
Vậy pt luôn có 2 nghiệm phân biệt với mọi giá trị của m.
b,\(x_1=\frac{4-\sqrt{4m^2+16}}{2};x_2=\frac{4+\sqrt{4m^2+16}}{2}\)
\(\Rightarrow\left|x_1+x_2\right|=\left|\frac{4-\sqrt{4m^2+16}+4+\sqrt{4m^2+16}}{2}\right|=\left|\frac{8}{2}\right|=4\)
pt luôn = 4
Sửa câu b
\(A=\left|x_1^2-x_2^2\right|=\left|\left(x_1-x_2\right)\left(x_1+x_2\right)\right|=\left|\left(\frac{4-\sqrt{4m^2+16}}{2}-\frac{4+\sqrt{4m^2+16}}{2}\right)\left(\frac{4-\sqrt{4m^2+16}}{2}+\frac{4+\sqrt{4m^2+16}}{2}\right)\right|\)\(\Leftrightarrow A=\left|-\left(\sqrt{4m^2+16}\right).4\right|\)
Vì \(4m^2+16>0\)
\(\Rightarrow A=\sqrt{4m^2+16}.4\ge\sqrt{16}.4=4^2=16\)
Vậy MinA = 16
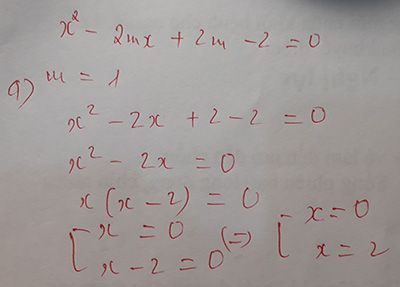
Xét \(\Delta=\left(2m-1\right)^2-8\left(m-1\right)=4m^2-12m+9=\left(2m-3\right)^2\ge0\)
=> PT luôn có 2 nghiệm x1,x2 với mọi m
Theo hệ thức Viet ta có \(\hept{\begin{cases}x_1+x_2=\frac{1-2m}{2}\\x_1x_2=\frac{m-1}{2}\end{cases}}\)
\(\Rightarrow A=\left(x_1+x_2\right)^2-3x_1x_2=\left(\frac{1-2m}{2}\right)^2-\frac{3\left(m-1\right)}{2}\)
\(=\frac{1-4m+4m^2-6m+6}{4}=\frac{4m^2-10m+7}{4}\)
\(=\frac{\left(2m-\frac{5}{2}\right)^2+\frac{3}{4}}{4}\ge\frac{3}{16}\)
Dấu "=" xảy ra khi \(2m=\frac{5}{2}\Rightarrow m=\frac{5}{4}\Rightarrow\frac{a}{b}=\frac{5}{4}\)
\(\Rightarrow4a=5b\Rightarrow2a=\frac{5b}{2}\)
lúc đó \(P=\frac{5b}{2}+2b=\frac{9b}{2}\)