Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
1: D thuộc Ox nên D(x;0)
vecto AB=(-3;4)
vecto DC=(-3-x;-1)
Để ABDC là hình thang thì \(\dfrac{-3}{-x-3}=\dfrac{4}{-1}=-4\)
=>3/x+3=4
=>x+3=3/4
=>x=-9/4
2: \(\overrightarrow{MA}=\left(3-x;0\right)\)
vectoMC=(-3-x;-1)
Để |vecto MA+vecto MC| nhỏ nhất thì vecto MA+vecto MC=vecto 0
=>M là trung điểm của AC
=>M(0;-1/2)
MA+MC= MA-MB
<=> 2 MI=BA
=> MI=BA/2
=> I thuộc đường tròn I bán kính AB/2
nãy mk quên giải thik:
a, gọi I la trung điểm của AC=> MA+MC=2MI
hok tốt
\(\overrightarrow{NC}=2\overrightarrow{ND}=2\overrightarrow{NC}+2\overrightarrow{CD}\Rightarrow\overrightarrow{NC}=2\overrightarrow{DC}\Rightarrow\overrightarrow{CN}=2\overrightarrow{CD}\)
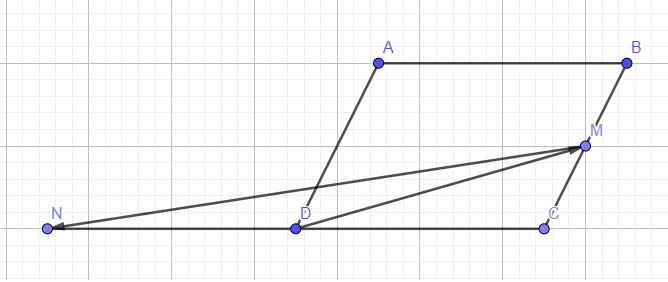
a.
\(\overrightarrow{DM}=\overrightarrow{DC}+\overrightarrow{CM}=\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{CB}=\overrightarrow{AB}-\dfrac{1}{2}\overrightarrow{AD}\)
\(\overrightarrow{MN}=\overrightarrow{MC}+\overrightarrow{CN}=\dfrac{1}{2}\overrightarrow{BC}+2\overrightarrow{CD}=-2\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{AD}\)
b.
\(\left\{{}\begin{matrix}\overrightarrow{AC}=\overrightarrow{AB}+\overrightarrow{AD}\\\overrightarrow{BD}=\overrightarrow{BA}+\overrightarrow{AD}=-\overrightarrow{AB}+\overrightarrow{AD}\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{AB}=\dfrac{1}{2}\overrightarrow{AC}-\dfrac{1}{2}\overrightarrow{BD}\\\overrightarrow{AD}=\dfrac{1}{2}\overrightarrow{AC}+\dfrac{1}{2}\overrightarrow{BD}\end{matrix}\right.\)
\(\Rightarrow\overrightarrow{MN}=-2\left(\dfrac{1}{2}\overrightarrow{AC}-\dfrac{1}{2}\overrightarrow{BD}\right)+\dfrac{1}{2}\left(\dfrac{1}{2}\overrightarrow{AC}+\dfrac{1}{2}\overrightarrow{BD}\right)=-\dfrac{3}{4}\overrightarrow{AB}+\dfrac{5}{4}\overrightarrow{BD}\)
Gọi tọa độ điểm \(M\) là \(M\left(x;y\right).\)
\(\overrightarrow{MA}=\left(1-x;3-y\right);\overrightarrow{MB}=\left(4-x;-y\right);\overrightarrow{MC}=\left(2-x;-5-y\right).\)
Ta có: \(\overrightarrow{MA}+\overrightarrow{MB}-3\overrightarrow{MC}=\overrightarrow{0}.\)
\(\left\{{}\begin{matrix}1-x+4-x-3\left(2-x\right)=0.\\3-y-y-3\left(-5-y\right)=0.\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}-2x+5-6+3x=0.\\3-2y+15+3y=0.\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x-1=0.\\y+18=0.\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=1.\\y=-18.\end{matrix}\right.\) \(\Rightarrow M\left(1;-18\right).\)