TQ
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
23 tháng 12 2023
Câu 5:
a: Khi m=3 thì \(f\left(x\right)=\left(2\cdot3+1\right)x-3=7x-3\)
\(f\left(-3\right)=7\cdot\left(-3\right)-3=-21-3=-24\)
\(f\left(0\right)=7\cdot0-3=-3\)
b: Thay x=2 và y=3 vào f(x)=(2m+1)x-3, ta được:
\(2\left(2m+1\right)-3=3\)
=>2(2m+1)=6
=>2m+1=3
=>2m=2
=>m=1
c: Thay m=1 vào hàm số, ta được:
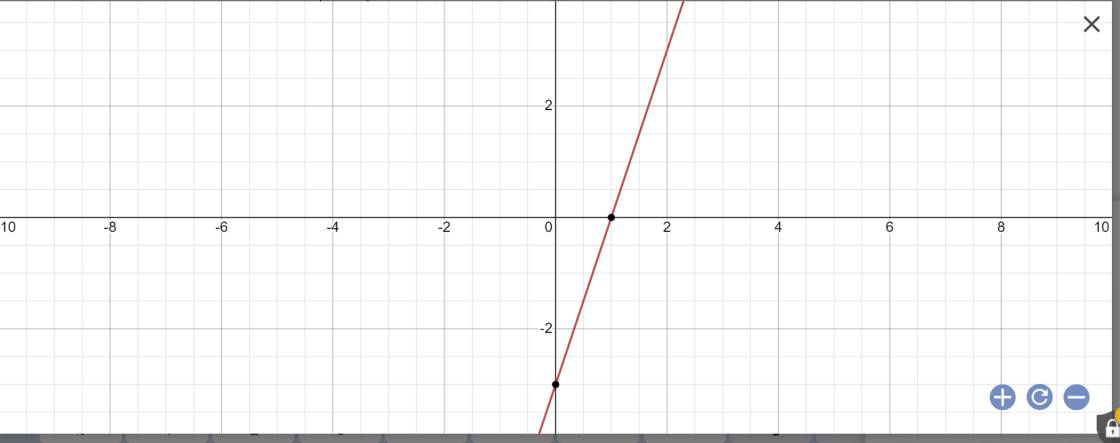
\(y=\left(2\cdot1+1\right)x-3=3x-3\)
*Vẽ đồ thị
d: Để hàm số y=(2m+1)x-3 là hàm số bậc nhất thì \(2m+1\ne0\)
=>\(2m\ne-1\)
=>\(m\ne-\dfrac{1}{2}\)
e: Để đồ thị hàm số y=(2m+1)x-3 song song với đường thẳng y=5x+1 thì \(\left\{{}\begin{matrix}2m+1=5\\-3\ne1\end{matrix}\right.\)
=>2m+1=5
=>2m=4
=>m=2
AH
Akai Haruma
Giáo viên
27 tháng 11 2023
Lời giải:
a. Để hs trên là hàm bậc nhất thì:
$4m2-4m+1\neq 0$
$\Leftrightarrow (2m-1)^2\neq 0$
$\Leftrightarrow 2m-1\neq 0$
$\Leftrightarrow m\neq \frac{1}{2}$
b.
$f(1)=(4m^2-4m+1).1-3=4m^2-4m-2=6$
$\Leftrightarrow 4m^2-4m-8=0$
$\Leftrightarrow m^2-m-2=0$
$\Leftrightarrow (m+1)(m-2)=0$
$\Leftrightarrow m=-1$ hoặc $m=2$
19 tháng 1
a: 
b: \(f\left(2\right)=\dfrac{1}{2}\cdot2=1\)
\(f\left(1\right)=\dfrac{1}{2}\cdot1=\dfrac{1}{2}\)
\(f\left(-2\right)=\dfrac{1}{2}\cdot\left(-2\right)=-1\)
\(f\left(-1\right)=\dfrac{1}{2}\cdot\left(-1\right)=-\dfrac{1}{2}\)
\(f\left(0\right)=\dfrac{1}{2}\cdot0=0\)
c: f(x)=2
=>\(\dfrac{1}{2}x=2\)
=>x=2*2=4
f(x)=1
=>\(\dfrac{1}{2}x=1\)
=>\(x=1:\dfrac{1}{2}=2\)
f(x)=-1
=>\(\dfrac{1}{2}x=-1\)
=>\(x=-1\cdot2=-2\)
d: \(f\left(-1\right)=\dfrac{1}{2}\cdot\left(-1\right)=-\dfrac{1}{2}\ne\dfrac{1}{2}=y_A\)
=>A(-1;1/2) không thuộc đồ thị hàm số y=1/2x
\(f\left(-1\right)=\dfrac{1}{2}\cdot\left(-1\right)=-\dfrac{1}{2}=y_B\)
=>\(B\left(-1;-\dfrac{1}{2}\right)\) thuộc đồ thị hàm số y=1/2x
20 tháng 11 2022
a: y tỉ lệ thuận với x theo hệ số tỉ lệ 1/2 nên y=1/2x
f(x)=-5
=>1/2x=-5
=>x=-10
b: Vì a=1/2>0
nên f(x) đồng biến trên R
=>KHi x1>x2 thì f(x1)>f(x2)
13 tháng 11 2023
a: \(F\left(3\right)=3\left(3-2\right)=3\cdot1=3\)
\(\left[F\left(\dfrac{2}{3}\right)\right]^2=\left[\dfrac{2}{3}\cdot\left(\dfrac{2}{3}-2\right)\right]^2\)
\(=\left[\dfrac{2}{3}\cdot\dfrac{-4}{3}\right]^2=\left(-\dfrac{8}{9}\right)^2=\dfrac{64}{81}\)
\(G\left(-\dfrac{1}{2}\right)=-\left(-\dfrac{1}{2}\right)+6=6+\dfrac{1}{2}=\dfrac{13}{2}\)
b: F(x)=0
=>x(x-2)=0
=>\(\left[{}\begin{matrix}x=0\\x-2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=2\end{matrix}\right.\)
c: F(a)=G(a)
=>\(a\left(a-2\right)=-a+6\)
=>\(a^2-2a+a-6=0\)
=>\(a^2-a-6=0\)
=>(a-3)(a+2)=0
=>\(\left[{}\begin{matrix}a-3=0\\a+2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}a=3\\a=-2\end{matrix}\right.\)
R
1
R
1
11 tháng 2 2017
A=\(2^0+2+...+2^8-\left(0+1+...+8\right)-9.4\)
=\(2^9-1-36-36=439\)
17 tháng 8 2016
a) Ta lần lượt có :
f ( - 2 ) = | 2-(-2)-3 | = | -4 - 3 | = | -7 | = 7
f( 8 ) = | 2x - 3 | = | 2 . 8 - 3 | = | 16 - 3 | = | 13 | = 13
b) Ta lần lượt có :
- Với y = -1 thì | 2x - 3 | = -1 , vô nghiệm bởi | 2x - 3 | > 0
- Với y = 3 thì | 2x - 3 | = 3
↔ 2x - 3 = 3 hoặc 2x - 3 = -3
↔ 2x = 6 hoặc 2x = 0
↔ x = 3 hoặc x = 0
F(2)+F(1)=8
=>\(2^2\left(m^2+1\right)+2\left(m^2+1\right)-5+m^2+1+2\left(m^2+1\right)-5=8\)
=>\(8\left(m^2+1\right)+m^2+1-10=8\)
=>\(9\left(m^2+1\right)=18\)
=>\(m^2+1=2\)
=>\(m^2=1\)
=>\(\left[{}\begin{matrix}m=1\\m=-1\end{matrix}\right.\)